Problem of the Month
(January 2014)

This month we are interested in rigid packings of rectangular boxes with 2×7, 3×5, and 4×4 bricks.

1. For a given size rectangle, what is the largest amount of wasted space possible?

2. Restricting the rectangles to squares, what is the largest amount of wasted space possible?

3. Fixing the width of the rectangles, what is the largest proportion of wasted space possible?

ANSWERS

Contributions were received from Maurizio Morandi, George Sicherman, and Jeremy Galvagni.

1.

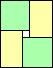
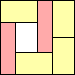
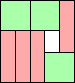
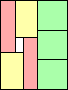
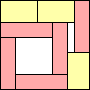
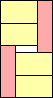
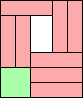
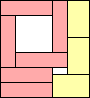
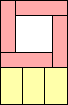
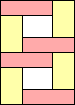
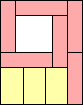
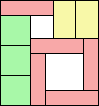
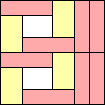
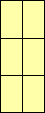
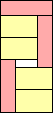
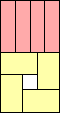
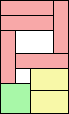
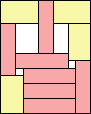
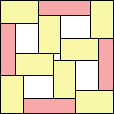
Here are the rigid packings of rectangles with the largest known wasted space.

Best Packings of Rectangles
m \ n23456789101112131415
4
5
6
7
8
9
10
11
12
13
14
 
 
 
 
 
 
 
 
 
(GS)
 
15
 
 
 
 
 
 
(GS)
 
 
(GS)
(GS)
(GS)
(GS)

Jeremy Galvagni noted that there are rigid rectangles with tilted rectangles. For example, a 2×7 brick can be tiled at an angle arctan(2/7) < θ < arctan(7/2) with wasted space 53sinθcosθ.

2.

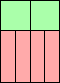
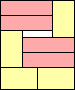
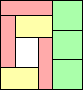
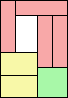
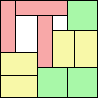
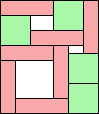
Here are the rigid packings of squares with the largest known wasted space.

Best Packings of Squares
4

wasted=0 		8

wasted=4 		9

wasted=25 		10

wasted=12 		11

wasted=16 		12

wasted=29 		13

wasted=19 		14

wasted=24 		15

wasted=49 (GS)
16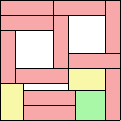

wasted=56 (GS) 		17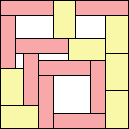

wasted=58 (GS) 		18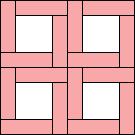

wasted=100 		19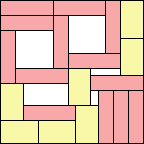

wasted=74 (GS) 		20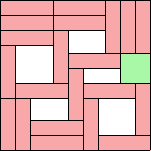

wasted=90 (GS)
3.

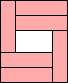
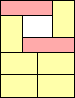
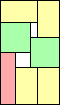
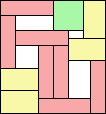
Here are the limited cases of rectangles with a given width with the largest known proportion of wasted space.

Best Limiting Cases With Fixed Width
2

0/14 		3

0/15 		4

0/16 		5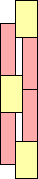

9/95
=.094+ 		6

10/54
=.185+ 		7

33/119
=.277+ 		8

26/160
=.162+ 		9

30/72
=.416+ 		10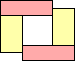

16/60
=.266+ 		11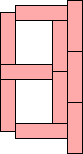

30/88
=.340+ 		12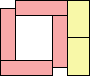

30/96
=.312+ 		13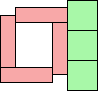

30/104
=.288+ 		14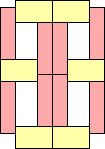

66/238
=.277+
Jeremy Galvagni noted that if the width is 11/√2, that 2×7 bricks can be tiled at a 45 degree angle to get a limit of 7/9 wasted space.

If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 1/1/14.