



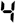


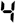


(MM)



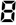









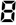


(MM)

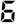
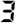


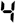

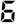
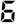

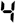
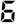
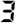

(BH)




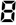



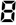




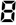



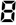
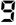




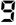


(MM)

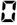



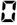

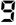

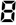



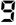

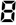

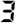




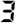











(MM)





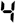



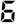

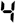

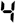



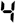

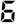


(MM)

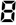

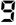



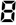





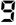
(BH)

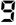

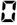



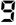



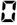





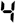

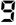





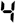



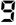
We can also use different counting systems. For each positive number, what is this shortest Roman Numeral Langford List, where the number of line segments between two numbers are counted? Or the shortest Digital Clock Langford List, where the number of LED segments between the two numbers are counted? Or the shortest Coinage Langford List, where the smallest number of US coins to represent that many cents are counted? By shortest, I mean the total number of letters, line segments, or coins.
Are there lists that contain each number 3 times, with the correct counts between neighboring pairs? What is the shortest example for each type?
Alex Rowen found a Langford list with three of each number: 1, 9, 1, 6, 1, 8, 2, 5, 7, 2, 6, 9, 2, 5, 8, 4, 7, 6, 3, 5, 4, 9, 3, 8, 7, 4, 3.
Then in 2025, he found a Langford list with four of each number: 4, 22, 9, 24, 14, 4, 6, 18, 20, 7, 4, 23, 9, 6, 15, 4, 21, 7, 12, 14, 6, 19, 9, 13, 22, 7, 18, 6, 24, 20, 15, 12, 9, 7, 14, 23, 5, 13, 21, 16, 17, 19, 5, 11, 12, 18, 15, 22, 5, 14, 20, 13, 10, 24, 5, 11, 16, 12, 17, 23, 21, 19, 15, 10, 18, 13, 2, 11, 8, 2, 22, 20, 2, 16, 10, 2, 17, 8, 24, 11, 3, 19, 21, 23, 3, 10, 8, 1, 3, 1, 16, 1, 3, 1, 17, 8.
Here are the shortest known Language Langford lists containing a given number n:
| 3, 5, 7, 10 | 4, 8, 9 | 6, 13 | 11, 16, 23 | 12, 17, 27 | 14, 22 | 15, 29 | 18, 21 |
|---|---|---|---|---|---|---|---|
|
THREE TEN THREE SEVEN TEN FIVE SEVEN FIVE |
FOUR NINE FOUR EIGHT NINE FIVE EIGHT FIVE |
SIX ELEVEN SIX FOURTEEN ELEVEN THIRTEEN FOURTEEN EIGHT THIRTEEN EIGHT |
ELEVEN TWENTYTHREE ELEVEN SEVEN SIXTEEN SEVEN TWENTYTHREE SIXTEEN |
SEVENTEEN TWENTYSEVEN TWELVE SEVENTEEN SIX TWELVE SIX TWENTYSEVEN |
NINE TWENTYTWO NINE EIGHT FOURTEEN EIGHT TWENTYTWO FOURTEEN |
SIX TWENTY SIX TWENTYNINE FIFTEEN TWENTY SEVENTEEN FIFTEEN TWENTYNINE SEVENTEEN |
NINE TWENTYONE NINE EIGHTEEN SEVENTEEN TWENTYONE EIGHTEEN SEVENTEEN |
| 19, 25 | 20 | 24 | 26 | 28 | 30 |
|---|---|---|---|---|---|
|
NINETEEN TWENTYTWO TWENTYFIVE NINETEEN NINE TWENTYTWO NINE TWENTYFIVE |
TWENTY TWENTYONE TWENTYTHREE TWENTY NINE TWENTYONE NINE TWENTYTHREE |
NINE TWENTYTWO NINE NINETEEN TWENTYFOUR TWENTYTWO NINETEEN FIFTEEN TWENTYFOUR SEVEN FIFTEEN SEVEN |
TWELVE TWENTYONE SIX TWELVE SIX TWENTYSIX TWENTYONE EIGHTEEN SEVENTEEN TWENTYSIX EIGHTEEN SEVENTEEN |
SEVEN SIXTEEN SEVEN TWENTYEIGHT SIXTEEN TWENTYFIVE TWENTYSEVEN TWENTYEIGHT TEN TWENTYFIVE TEN TWENTYSEVEN |
EIGHT EIGHTEEN EIGHT THIRTY SIXTEEN EIGHTEEN THIRTEEN SIXTEEN THIRTY THIRTEEN |
Bryce Herdt found this 2-dimensional Langford Array using the consecutive numbers from 3 to 12:
|
Here are the shortest known Roman Numeral Langford Lists containing a given number n:
| 2, 5, 6 | 3, 9 | 4, 10 | 7 | 8 | 11 | 12, 15 | 13 | 14 | 16 | 17 | 18 | 19 | 20 | 21, 23 | 22, 25 | 24
|
V | VI II V II VI
VI | IX III VI III IX
IV | V X IV V VI II X II VI (MM)
II | X II XV VII X VIII VII XV VIII (MM)
VIII | XII XV VIII IV XII IV XV (MM)
IX | XI II V II IX V XI
XII | XIV XV IV XII IV XIV XV
V | XIII V XII XV IX XIII XII IX XV (MM)
XIV | V VI II V II VI XIV
XV | VII XVI X VII XV II X II XVI (MM)
XI | XVII XXI XI III IX III XVII IX XXI (MM)
XVIII | VI IX III VI III IX XVIII
VIII | XIX XI VIII II V II XI V XIX (MM)
XX | IX XI II V II IX V XI XX
XXIII | XXXI XXI II V II IX V XXIII IX XXI XXXI (BH) ?
|
XXIV | XIV V VI II V II VI XIV XXIV (BH) |
|---|
Here are the shortest known Digital Clock Langford Lists containing a given number n:
| 4, 11, 12 | 5, 15, 28 | 6, 16 | 7, 8, 17, 25 | 9 | 10 | 13 | 14, 26 | 18, 29 | 19, 20, 27 | 21, 22, 23 | 24 | 30 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
    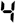   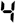   (MM) |
   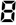          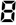   (MM) |
 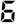 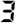   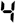  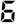 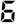  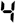 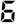 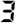  (BH) |
    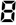    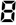   
|
 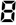    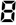 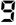     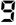   (MM) |
 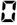    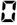  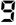  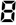    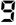  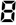
|
 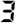     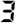            (MM) |
     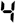    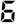  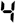  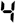    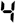  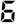   (MM) |
 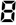  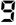    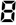      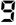 (BH) |
 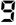  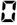    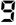    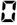    
| ? |
 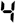  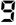      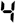    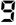
| ? |
Bryce Herdt's solution for 6 and 16 contains only even numbers, and Mark Mammel's list for 13 only contains odd numbers. Bryce wondered whether there were lists having greatest common factor higher than 2.
Here are the shortest known Coinage Langford Lists containing a given number n:
| 1, 4 | 2, 3, 5, 6 | 7 | 8 | 9 | 10 |
|---|---|---|---|---|---|
                        (MM) |
               
|
               
|
                          (MM) |
                      (MM) |
                  (MM) |
| 11 | 12 | 13 | 14 | 15 | 16 |
|---|---|---|---|---|---|
                  (MM) |
                    (MM) |
                        (MM) |
                          |
                      (MM) |
                      (BH) |
| 17 | 18 | 19 | 20 |
|---|---|---|---|
                              (MM) |
                            (MM) |
                                          (MM) |
                          (MM) |
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 1/1/17.