
1

2

3
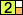
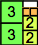
6
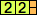
10

16
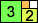
15
30
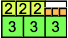
45

35

64

98

60
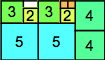
112
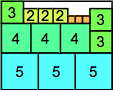
180

99
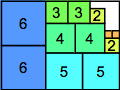
192
280
154
286
429

231
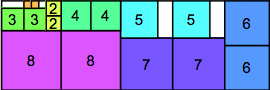
432

630
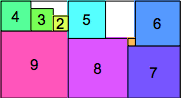
312
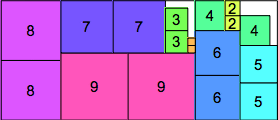
592
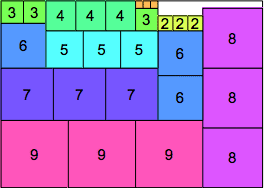
875
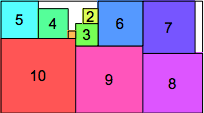
405
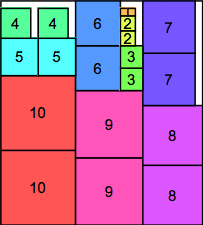
810
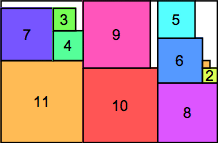
551
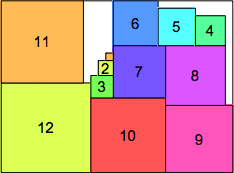
713
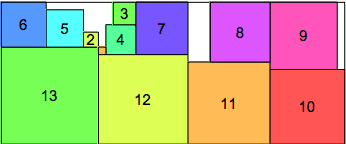
874
Here are the best known results.
| n \ k | 1 | 2 | 3 |
|---|---|---|---|
| 1 |  1 |  2 |  3 |
| 2 | 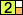 6 | 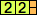 10 |  16 |
| 3 | 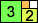 15 |  30 |  45 |
| 4 |  35 |  64 |  98 |
| 5 |  60 |  112 |  180 |
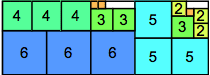
| 6 |  99 |  192 |  280 |
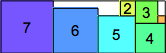
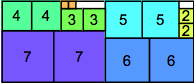
| 7 |  154 |  286 |  429 |
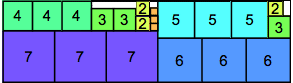
| 8 |  231 |  432 |  630 |
| 9 |  312 |  592 |  875 |
| 10 |  405 |  810 | 1178 |
| 11 |  551 | 1026 | 1548 |
| 12 |  713 | 1342 | 1995 |
| 13 |  874 | 1694 | 2486 |
| 14 | 1081 | 2075 | ? |
| 15 | 1352 | 2548 | ? |
| 16 | 1610 | 3060 | ? |
| 17 | 1875 | 3648 | ? |
| 18 | 2240 | 4320 | ? |
| 19 | 2673 | 5100 | ? |
| 20 | 3045 | 5848 | ? |
| 21 | 3498 | ? | ? |
| 22 | 4025 | ? | ? |
| 23 | 4620 | ? | ? |
| 24 | 5145 | ? | ? |
| 25 | 5852 | ? | ? |
| 26 | 6594 | ? | ? |
| 27 | 7332 | ? | ? |
| 28 | 8127 | ? | ? |
| 29 | 8976 | ? | ? |
| 30 | 10036 | ? | ? |
| 31 | 10935 | ? | ? |
| 32 | 12036 | ? | ? |
| 33 | 13338 (ML) | ? | ? |
| 34 | 14455 (ML) | ? | ? |
| 35 | 15635 (ML) | ? | ? |
| 36 | 17226 (ML) | ? | ? |
| 37 | 18492 (ML) | ? | ? |
| 38 | 19965 (ML) | ? | ? |
| 39 | 21594 (ML) | ? | ? |
| 40 | 23270 (ML) | ? | ? |
| 41 | 25132 (ML) | ? | ? |
| 42 | 26775 (ML) | ? | ? |
| 43 | 28676 (ML) | ? | ? |
| 44 | 31070 (ML) | ? | ? |
| 45 | 32964 (ML) | ? | ? |
| 46 | 34914 (ML) | ? | ? |
| 47 | 37500 (ML) | ? | ? |
| 48 | 40089 (ML) | ? | ? |
| 49 | 42245 (ML) | ? | ? |
| 50 | 44891 (ML) | ? | ? |
| 51 | 47891 (ML) | ? | ? |
| n \ m | 2 | 3 | 4 |
|---|---|---|---|
| 2 | 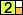 6 | ||
| 3 | 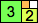 15 |  18 | |
| 4 |  35 |  36 |  40 |
| 5 |  63 |  60 |  65 |
| 6 |  99 |  105 |  108 |
| 7 | 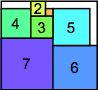 156 |  162 | 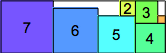 154 |
| 8 | 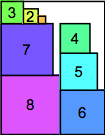 252 |  231 |  234 |
| 9 | 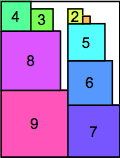 336 | 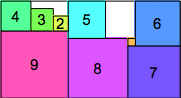 312 | 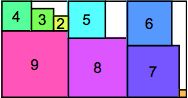 325 |
| 10 | 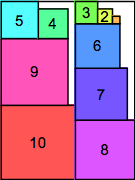 432 | 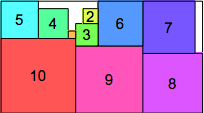 405 | 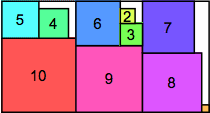 420 |
| 11 | 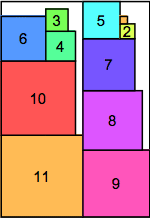 580 | 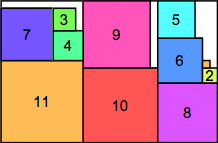 551 | 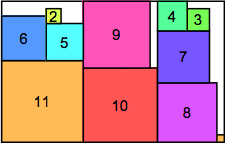 570 |
| 12 |  748 |  713 | 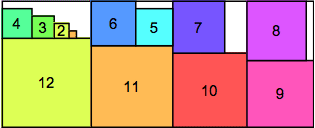 714 |
| 13 |  912 |  945 |  874 |
| 14 | 1200 | 1140 | 1081 |
| 15 | 1431 | 1368 | 1352 |
| 16 | 1740 | 1680 | 1650 |
| 17 | 2077 | 1980 | 1947 |
| 18 | 2464 | 2350 | 2331 |
| 19 | 2835 | 2754 | 2680 |
| 20 | 3348 | 3180 | 3128 |
| 21 | 3876 | 3640 | 3600 |
| 22 | 4480 | 4218 | 4104 |
| 23 | 5082 | 4819 | 4720 |
| 24 | 5676 | 5456 | 5330 |
| 25 | 6440 | 6090 | 6035 |
| 26 | 7238 | 6862 | 6750 |
| 27 | 8134 | 7700 | ? |
| 28 | 9100 | 8568 | ? |
| 29 | 10036 (ML) | ? | ? |
| 30 | 11124 (ML) | ? | ? |
| 31 | 12255 (ML) | ? | ? |
| 32 | 13398 (ML) | ? | ? |
| 33 | 14760 (ML) | ? | ? |
| 34 | 16368 (ML) | ? | ? |
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 2/9/19.