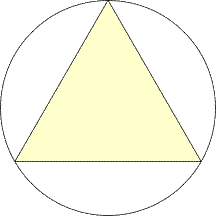
r = 1/√3 = .5773+
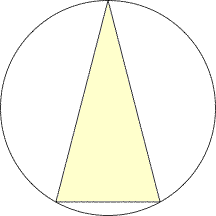
r = 4/√15 = 1.0327+
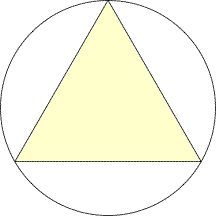
r = 2/√3 = 1.1547+
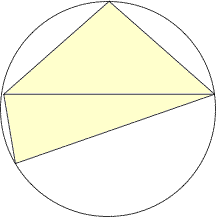
r = 1.5302+
3 r = 1/√3 = .5773+ | 5 r = 4/√15 = 1.0327+ | 6 r = 2/√3 = 1.1547+ | 7 r = 1.5302+ |
8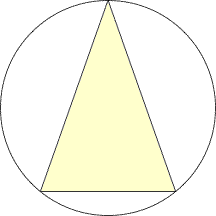 r = 9/4√2 = 1.5909+ | 9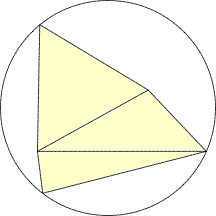 r = 2.5468+ | 10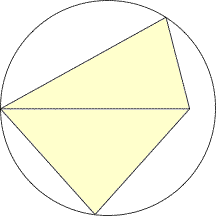 r = 12√(251-3√3)/37√5 = 2.2739+ | 11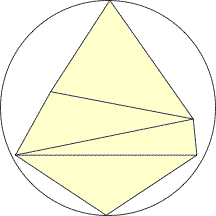 r = 2.9634+ |
12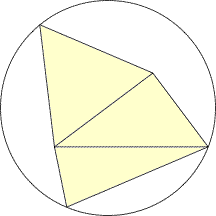 r = 3.4968+ | 13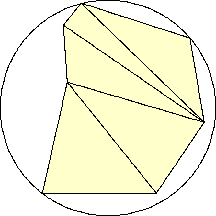 r = 3.7801+ (Jon Palin) | 14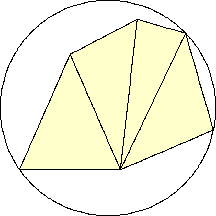 r = 4.2752+ (Jon Palin) | 15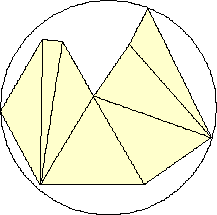 r = 5.1607+ (Maurizio Morandi) |
16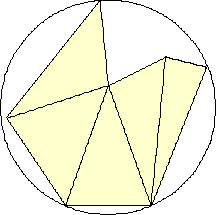 r = 5.0334+ (Maurizio Morandi) | 17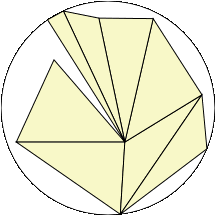 r = 5.9147+ (Joe DeVincentis) | 18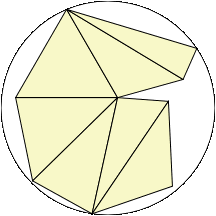 r = 6.2967+ (Joe DeVincentis) | 19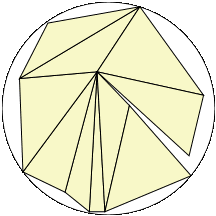 r = 6.8379+ (Joe DeVincentis) |
20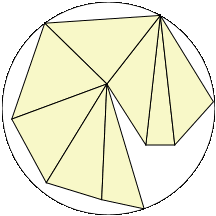 r = 7.3534+ (Joe DeVincentis) | 21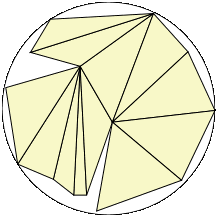 r = 8.2535+ (Joe DeVincentis) | 22 r = 8.5766+ (Joe DeVincentis) | 23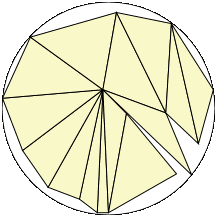 r = 9.5137+ (Joe DeVincentis) |
24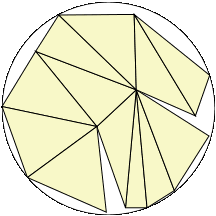 r = 9.8888+ (Joe DeVincentis) | 25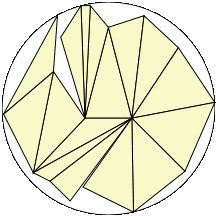 r = 11.3464+ (Joe DeVincentis) | 26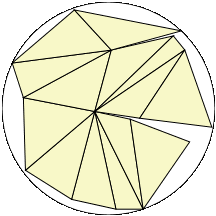 r = 11.7197+ (Joe DeVincentis) | 27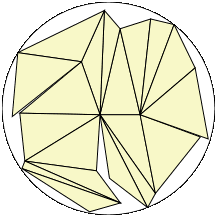 r = 13.2703+ (Joe DeVincentis) |
28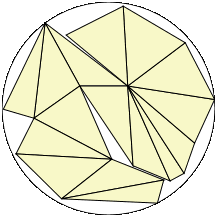 r = 13.3210+ (Joe DeVincentis) | 29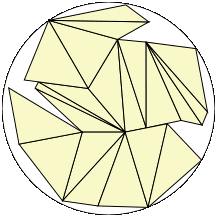 r = 14.8887+ (Joe DeVincentis) | 30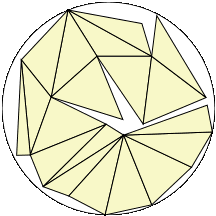 r = 15.5952+ (Joe DeVincentis) |
3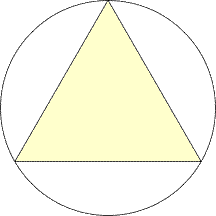 r = 1/√3 = .5773+ | 5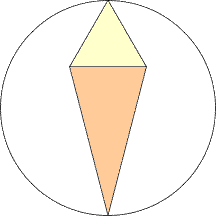 r = (√15+√3)/4 = 1.4012+ | 6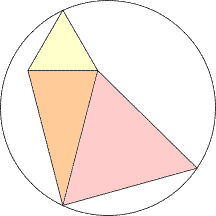 r = 2√(52+9√5)/11 = 1.5441+ | 7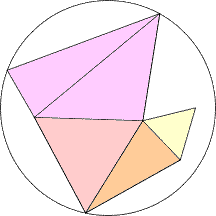 r = 1.9816+ |
8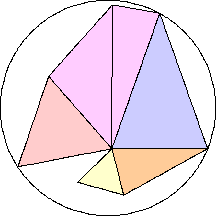 r = 2.2516+ (Jon Palin) | 9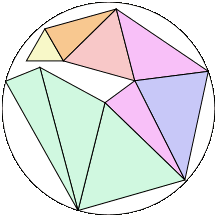 r = 2.8745+ (Joe DeVincentis) | 10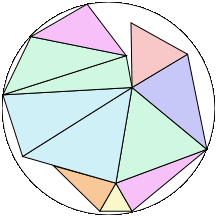 r = 3.2920+ (Joe DeVincentis) | 11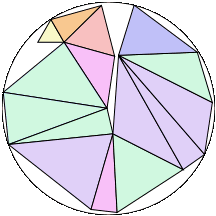 r = 4.0568+ (Joe DeVincentis) |
12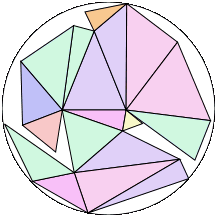 r = 5.0037+ (Joe DeVincentis) | 13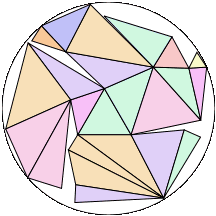 r = 5.9851+ (Joe DeVincentis) | 14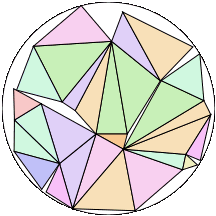 r = 6.7984+ (Joe DeVincentis) | 15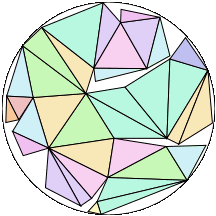 r = 8.0494+ (Joe DeVincentis) |
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 2/13/13.