
1 path
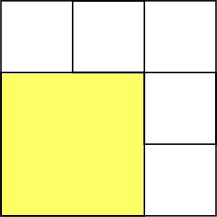
4 paths

8 paths
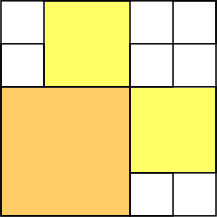
8 paths

14 paths
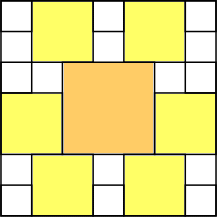
24 paths
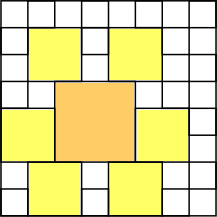
30 paths
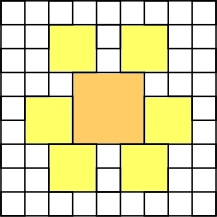
36 paths
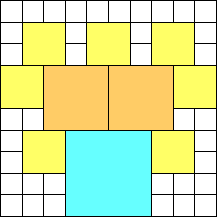
40 paths (JD)

50 paths (GS)

67 paths (GS)
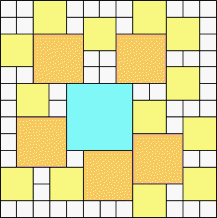
81 paths (MM)
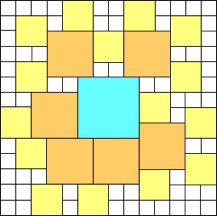
97 paths (MM)
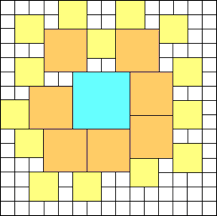
108 paths (MM)
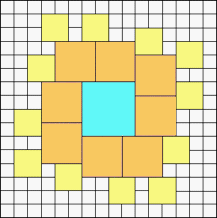
116 paths (MM)
n=1 1 path | n=3 4 paths | n=4 8 paths | n=5 8 paths |
n=6 14 paths | n=7 24 paths | n=8 30 paths | n=9 36 paths |
n=10 40 paths (JD) | n=11 50 paths (GS) | n=12 67 paths (GS) | n=13 81 paths (MM) |
n=14 97 paths (MM) | n=15 108 paths (MM) | n=16 116 paths (MM) |
n=1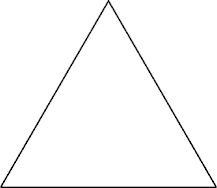 1 path | n=3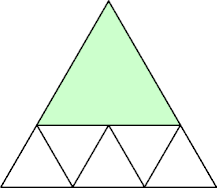 2 paths (GS) | n=4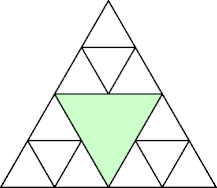 6 paths (GS) | n=5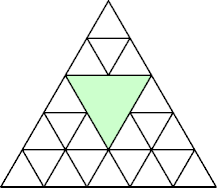 6 paths (GS) |
n=6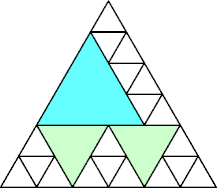 9 paths (GS) | n=7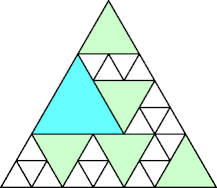 13 paths (GS) | n=8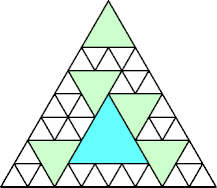 19 paths (GS) | n=9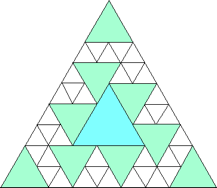 27 paths (MM) |
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 3/22/17.