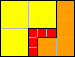
 | 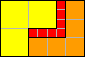 |
 |  |
What other rectangles can be packed with consecutive L's? Can you find a rectangle that can be packed with different numbers of consecutive L's? Is it possible to pack consecutive L's into a square? I'll offer $10 to the first person who finds such a packing.
| n | Rectangles |
|---|---|
| 3 | 6×8, 6×9 |
| 4 | 8×18, 8×22, 9×14, 9×16, 9×17, 9×19, 10×13, 10×14, 10×17, 10×18, 10×22 |
| 5 | 12×26, 15×18, 15×19, 15×20, 15×21, 15×23, 15×26, 15×27, 15×29, 15×30, 15×31, 15×32, 15×35, 15×38, 15×39, 15×43, 15×47, 15×50, 15×51, 15×55, 15×58, 15×59, 15×63, 15×66, 15×69, 15×70, 15×75 |
| 6 | 15×26, 15×36, 15×45, 15×66, 15×75, 15×80, 16×45, 16×75, 18×25, 18×26, 18×27, 18×29, 18×31, 18×32, 18×33, 18×37, 18×39, 18×40, 18×42, 18×43, 18×45, 18×49, 18×50, 18×51, 18×53, 18×55, 18×61, 18×63, 18×65, 18×66, 18×69, 18×73, 18×74, 18×75, 18×79, 18×81, 19×28, 19×61, 20×21, 20×25, 20×26, 20×32, 20×35, 20×36, 20×45, 20×51, 20×55, 20×56, 20×75, 21×24, 21×25, 21×26, 21×27, 21×32, 21×33, 21×34, 21×36, 21×38, 21×39, 21×41, 21×44, 21×45, 21×48, 21×50, 21×51, 21×56, 21×57, 21×61, 21×62, 21×63, 21×69, 21×70, 21×74, 21×75, 21×80, 21×81 |
Joseph DeVincentis showed that one dimension of a rectangle packed with n consecutive L's must be no larger than n(n+1)/2. His proof: Take a rectangle with height larger than n(n+1)/2. Each column must contain a vertical part of an L. But the total width of the vertical parts of L's is only n(n+1)/2, so the rectangle can only be that wide.
Joseph DeVincentis also showed that both dimensions of the bounding rectangle must be at least 2n, since that is the size of the largest L. He doubts whether a rectangle of width 2n can be achieved for more than 4 L's. He also remarked that n(n+1)/2 seems to be the most common width of these L packings.
Jeremy Galvagni and Joseph DeVincentis explained how to extend packings of n L's to (n+1) L's in certain cases.
Jeremy Galvagni found a way to pack n consecutive L's (where n is odd) into a n(n+1)/2 × (n+1)2/2 rectangle or a n(n+1)/2 × n(n+3)/2 rectangle. His method is illustrated below for n=9:
 |  |
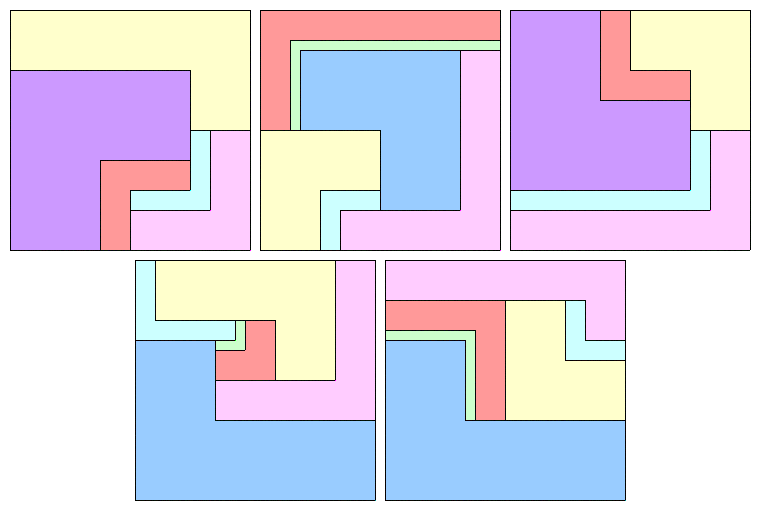
I managed to find several square packings of non-consecutive L's, the smallest of which was 24×24. Patrick Hamlyn did a computer search of all smaller squares and claimed that a 24×24 square is the smallest possible, and that there are 5 possible packings, shown below.
But then Joseph DeVincentis found this 20×20 square tiled with L's:
Jeremy Galvagni found a square ring made from 8 consecutive L's:
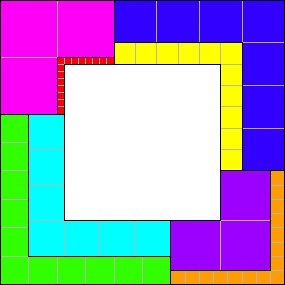
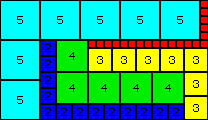
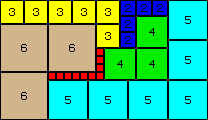
Joseph DeVincentis found many sets of two L packings of the same rectangle that use different numbers of L's. The 15×26 rectangles below are the smallest set.
 |
 |
Patrick Hamlyn found what he thinks is the smallest L packing for 7 consecutive L's, a 22×27 rectangle. What are the smallest packings of larger numbers of consecutive L's?
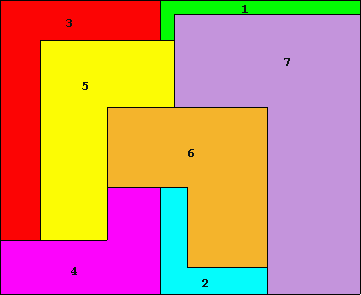
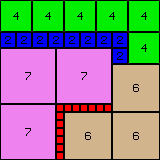
Joseph DeVincentis won my $10 prize for finding two 45×45 squares tiled with 9 consecutive L's, each of which can be extended to a square tiled with 10 or 11 consecutive L's. He also found a 78×78 square tiled with 12 consecutive L's that can be extended to a 13 L tiling. Below is his smallest square, a 36×36 square tiled with 8 consecutive L's
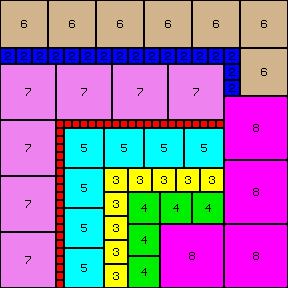
Then Patrick Hamlyn found a 28x28 square packed with 7 consecutive L's:
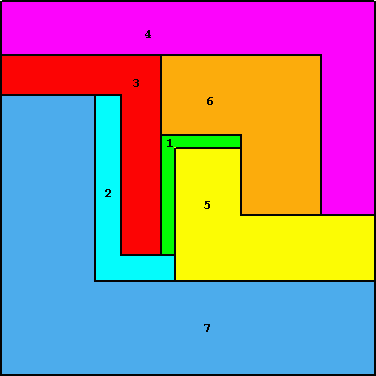
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 4/30/02.