Problem of the Month (April 2015)
What is the largest non-overlapping area that can be filled in a regular hexagon or an equilateral triangle by m congruent regular hexagons and n congruent equilateral triangles?
ANSWERS
Solutions this month were received from David Cantrell, Maurizio Morandi, Jeremy Galvagni, Joe DeVincentis, and George Sicherman.
Here are the best known answers:
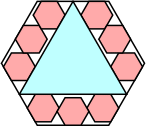
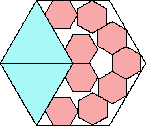
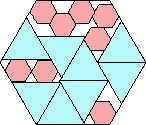
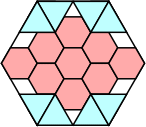
Best Packings of Hexagons
| m \ n | 0 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9
|
|---|
| 0
| 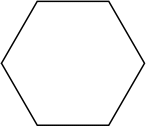
0 = .000
| 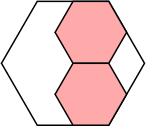
1/2 = .500
| 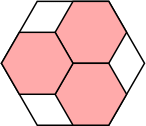
3/4 = .750
| 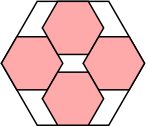
36/49 = .734+
| 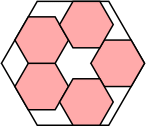
45/64 = .703+
| 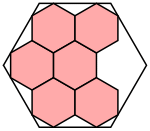
18/25 = .720 (MM)
| 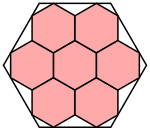
21/25 = .840 (MM)
| 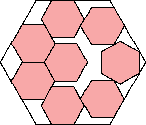
.728+ (MM)
| 
.683+ (MM)
|
|---|
| 1
| 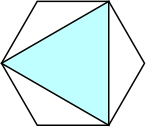
1/2 = .500
| 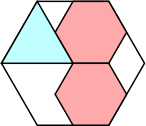
2/3 = .666+
| 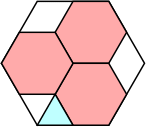
19/24 = .791+
| 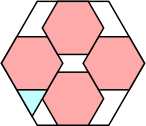
75/98 = .765+
| 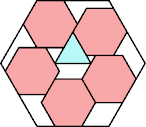
143/192 = .744+ (GS)
| 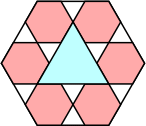
5/6 = .833+
| 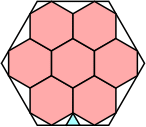
127/150 = .846+ (MM)
| 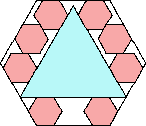
61/78 = .782+ (MM)
| 
59/72 = .819+
|
|---|
| 2
| 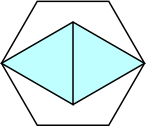
4/9 = .444+
| 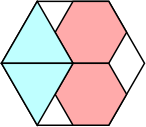
5/6 = .833+
| 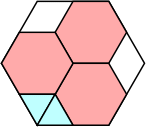
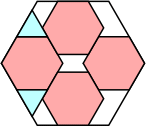
5/6 = .833+
| 
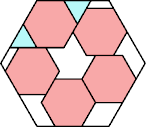
39/49 = .795+
| 
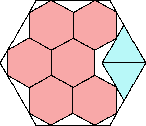
3/4 = .750 (GS)
| 
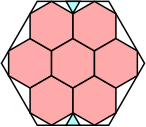
21/25 = .840 (MM)
| 
64/75 = .853+ (MM)
| 
5/6 = .833+
| 
.799+ (MM)
|
|---|
| 3
| 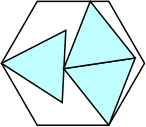
.510+
| 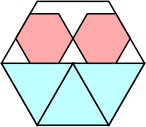
41/50 = .820
| 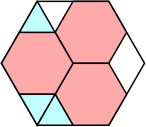
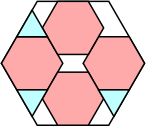
7/8 = .875
| 
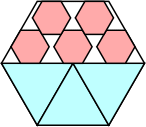
81/98 = .826+
| 
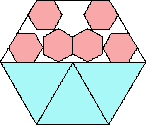
77/90 = .855+
| 
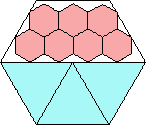
.835+ (MM)
| 
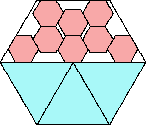
131/150 = .873+ (MM)
| 
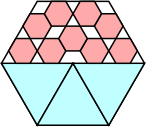
617/722 = .854+ (MM)
| 
43/50 = .860
|
|---|
| 4
| 
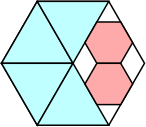
2/3 = .666+
| 
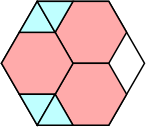
8/9 = .888+
| 
11/12 = .916+
| 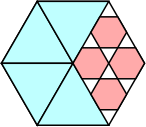
11/12 = .916+
| 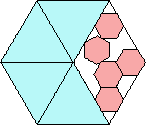
.870+ (MM)
| 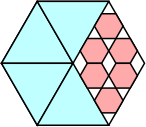
68/75 = .906+
| 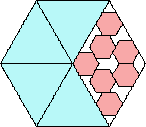
326/363 = .898+ (MM)
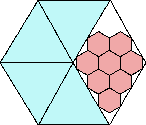
| 
68/75 = .906+ (MM)
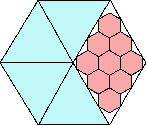
| 
281/300 = .936+ (MM)
|
|---|
| 5
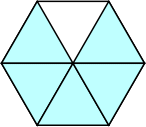
| 
5/6 = .833+
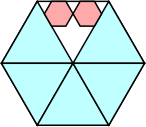
| 
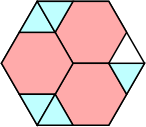
137/150 = .913+
| 
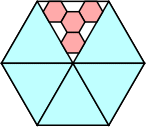
23/24 = .958+
| 
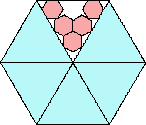
17/18 = .944+
| 
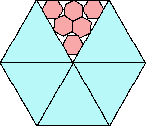
.940+ (MM)
| 
.959+ (MM)
| 
181/192 = .942+
| 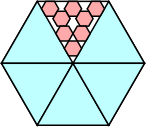
3557/3750 = .948+
| 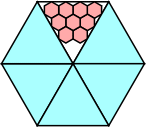
143/150 = .953+ (JD)
|
|---|
| 7
| 
.715+ (DC)
| 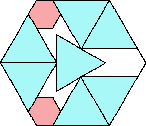
.802+ (MM)
| 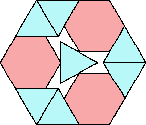
.916+ (MM)
| 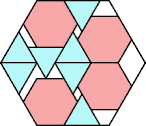
41/48 = .854+ (GS)
| 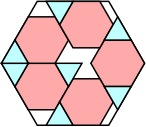
111/128 = .867+
| 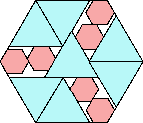
717/800 = .896+ (MM)
| 
49/54 = .907+
| 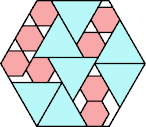
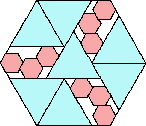
107/120 = .891+ (GS)
| 
897/1024 = .875+ (MM)
|
|---|
| 8
| 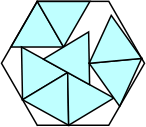
.725+ (DC)
| 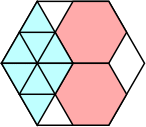
5/6 = .833+
| 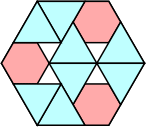
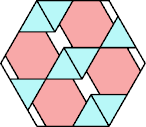
25/27 = .925+
| 
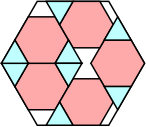
43/48 = .895+ (GS)
| 
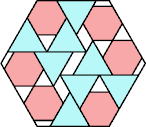
57/64 = .890+
| 
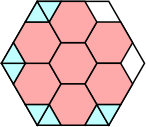
102/121 = .842+ (GS)
| 
25/27 = .925+
| 
8422/9747 = .864+ (MM)
| 
43/48 = .895+
|
|---|
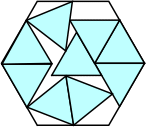
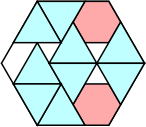
| 9
| 
.728+
| 
8/9 = .888+
| 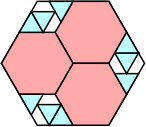
11/12 = .916+
| 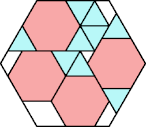
22/25 = .880 (GS)
| 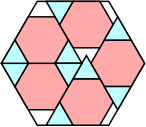
117/128 = .914+
| 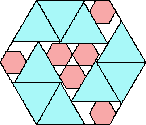
314/363 = .865+ (MM)
| 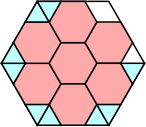
17/18 = .944+
| 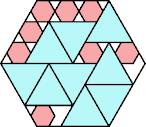
8/9 = .888+ (GS)
| 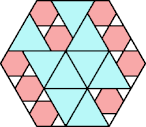
9/10 = .900 (GS)
|
|---|
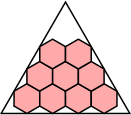
Best Packings of Triangles
| m \ n | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9
|
|---|
| 0
| 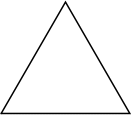
0 = .000
| 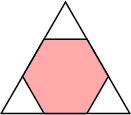
2/3 = .666+
| 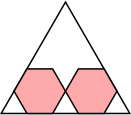
12/25 = .480
| 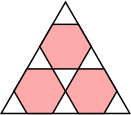
18/25 = .720
| 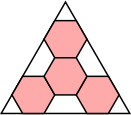
2/3 = .666+
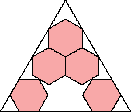
| 
.643+ (MM)
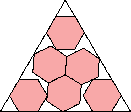
| 
.754+ (MM)
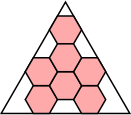
| 
21/32 = .656+
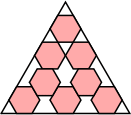
| 
432/625 = .691+
| 
18/25 = .720 (JD)
|
|---|
| 2
| 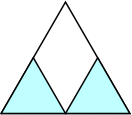
1/2 = .500
| 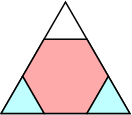
8/9 = .888+
| 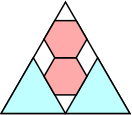
5/6 = .833+
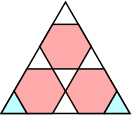
| 
4/5 = .800
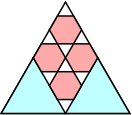
| 
7/8 = .875
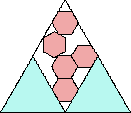
| 
.805+ (MM)
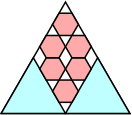
| 
43/50 = .860
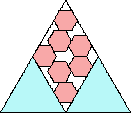
| 
205/242 = .847+ (MM)
| 
43/50 = .860 (MM)
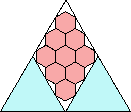
| 
181/200 = .905 (MM)
|
|---|
| 3
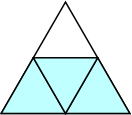
| 
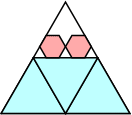
3/4 = .750
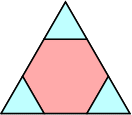
| 
1 = 1.000
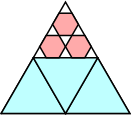
| 
87/100 = .870
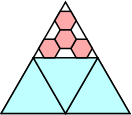
| 
93/100 = .930
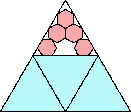
| 
11/12 = .916+
| 
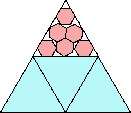
.910+ (MM)
| 
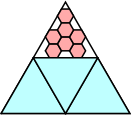
.938+ (MM)
| 
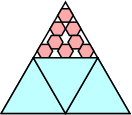
117/128 = .914+
| 
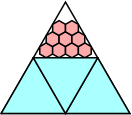
2307/2500 = .922+
| 
93/100 = .930 (JD)
|
|---|
| 5
| 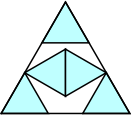
5(1–√3/2) = .669+
| 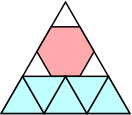
23/27 = .851+
| 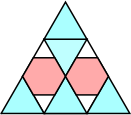
8/9 = .888+
| 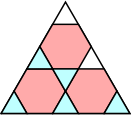
23/25 = .920
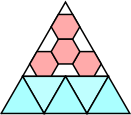
| 
23/27 = .851+
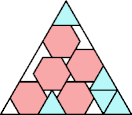
| 
165/196 = .841+ (GS)
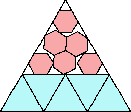
| 
.890+ (MM)
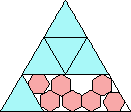
| 
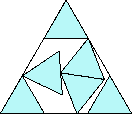
12941/15129=.855+(MM)
| 
7721/8649 = .892+ (MM)
| 
197/225 = .875+ (JD)
|
|---|
| 6
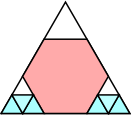
| 
.676+ (MM)
| 
5/6 = .833+
| 
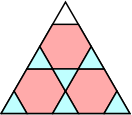
22/25 = .880
| 
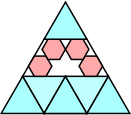
24/25 = .960
| 
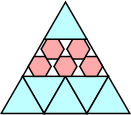
314/363 = .865+
| 
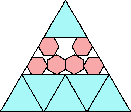
122/135 = .903+
| 
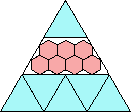
.890+ (MM)
| 
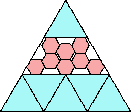
206/225 = .915+ (MM)
| 
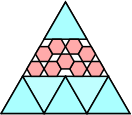
326/361 = .903+ (MM)
| 
68/75 = .906+
|
|---|
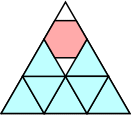
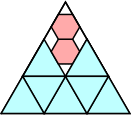
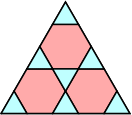
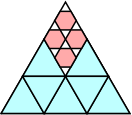
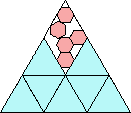
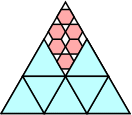
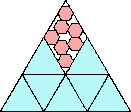
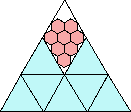
| 7
| 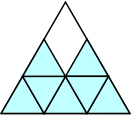
7/9 = .777+
| 
17/18 = .944+
| 
25/27 = .925+
| 
1 = 1.000
| 
17/18 = .944+
| 
.913+ (MM)
| 
211/225 = .937+
| 
1015/1089 = .932+ (MM)
| 
211/225 = .937+ (MM)
| 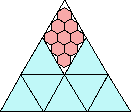
431/450 = .957+ (MM)
|
|---|
| 8
| 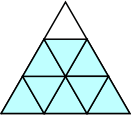
8/9 = .888+
| 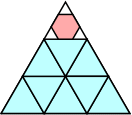
26/27 = .962+
| 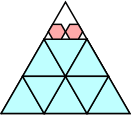
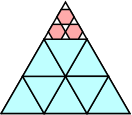
212/225 = .942+
| 
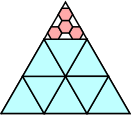
218/225 = .968+
| 
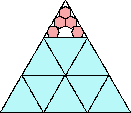
26/27 = .962+
| 
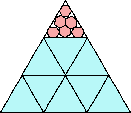
.960+ (MM)
| 
.972+ (MM)
| 
277/288 = .961+
| 
5432/5625 = .965+
| 
218/225 = .968+ (JD)
|
|---|
If you can extend any of these results, please
e-mail me.
Click here to go back to Math Magic. Last updated 5/1/15.






















































































































































