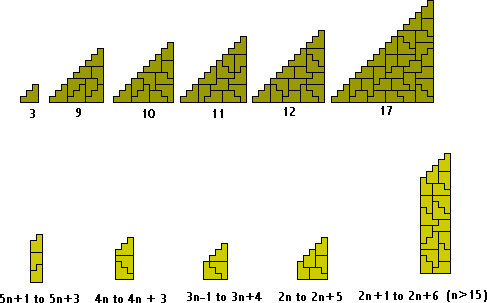Problem of the Month (May 2006)
The problem of which polyominoes can tile rectangles is well known (for example, see Mike Reid's Rectifiable Polyomino Page). Combinations of two polyominoes, neither of which tiles a rectangle separately, were considered in a previous monthly problem (January 2001). But which pairs of polyominoes can tile a triangle? How about a pyramid? How about a diamond? And what are the smallest such shapes that can be so tiled?
ANSWERS
Joe DeVincentis proved that straight polyominoes alone cannot tile any of these shapes, except of course the monomino. He also found the smallest tilings for tetrominoes and smaller polyominoes.
George Sicherman found the smallest tilings for tetrominoes, and found a diamond tiling using only R pentominoes.
Patrick Hamlyn found many smallest tilings with his tiling program.
Mike Reid found several large tilings with his tiling program. He also found many symmetric tilings, and proved the impossibility of some hexomino tilings.
Claudio Baiocchi found a few diamond tilings including bent triominoes.
Corey Plover used a tiling program to count the number of solutions for pentominoes in a triangle, and found that the smallest L+W, P+Y, P+W, and T+W tilings are unique.
Bernd Rennhak used a tiling program to count the number of solutions for pentominoes, and found that the smallest pyramid W+V tiling, and the smallest diamond W+U, F+U, and F+V tilings are unique.
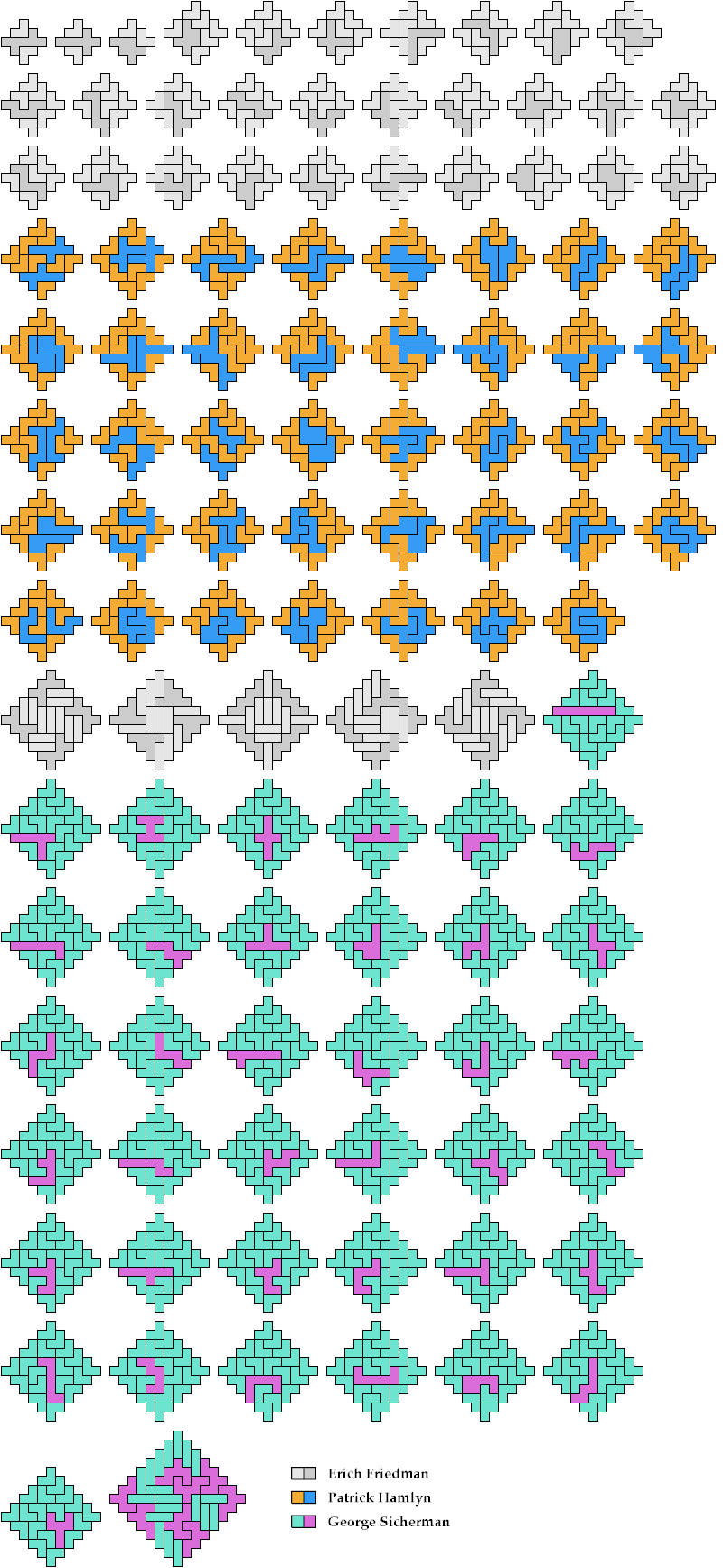
Here are the smallest known tilings for triangles, pyramids, and diamonds:
Triangles
|
|
| 3/5
| 
|
|
|
| 4/5
| 
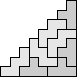
| 
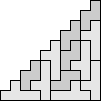
| 
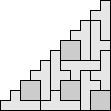
| 
| 
(Patrick Hamlyn)
| 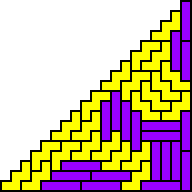
(Mike Reid)
|
|
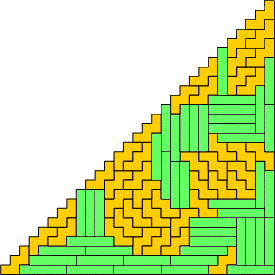
(Bernd Rennhak)
|
|
| 6
| 
|
|
|
|
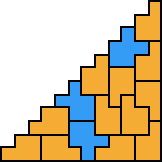
(Patrick Hamlyn)
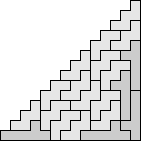
| 
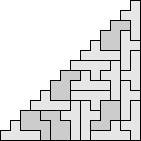
| 
| 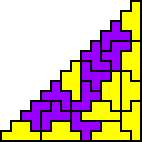
(Mike Reid)
| 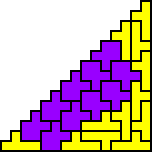
(Mike Reid)
|
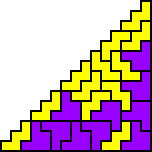
(Mike Reid)
| 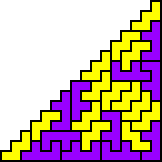
(Mike Reid)
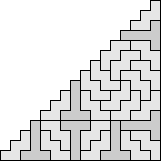
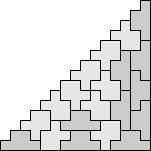
| 
| 
(Mike Reid)
|
(Mike Reid)
|
|
| 6/6
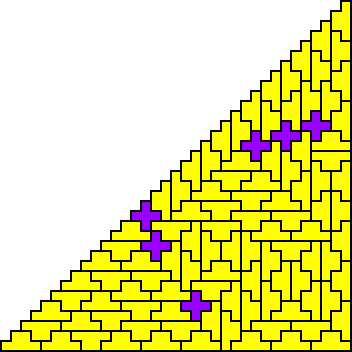
| 
| 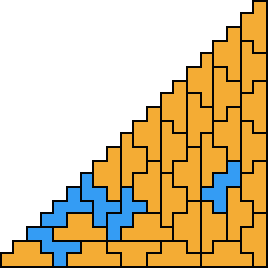
(Patrick Hamlyn)
| 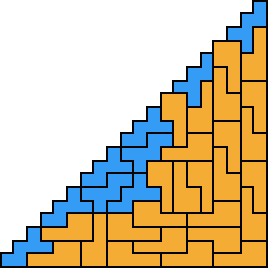
(Patrick Hamlyn)
|

(Mike Reid)
| 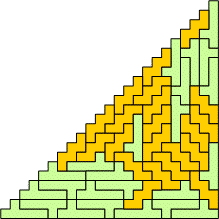
(Bernd Rennhak)
| 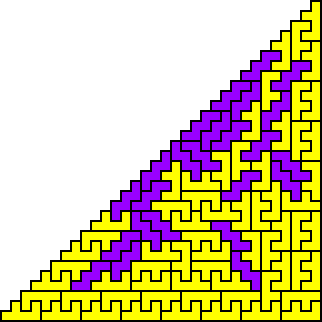
(Mike Reid)
|
|
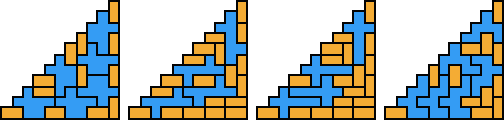
(Patrick Hamlyn)
|
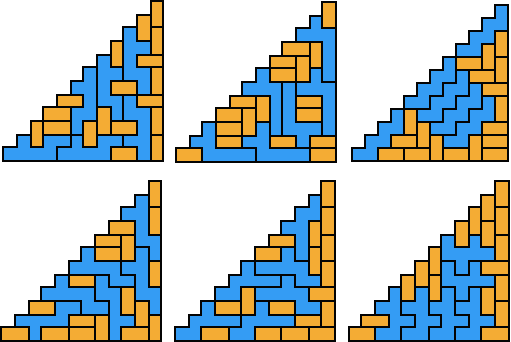
(Patrick Hamlyn)
|
|
| 3/7
| 
| 
(George Sicherman)
|
|
| 4/7
| 
| 
(Patrick Hamlyn)
| 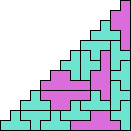
(George Sicherman)
| 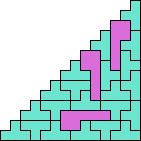
(George Sicherman)
|
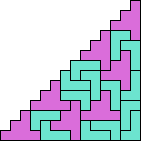
(George Sicherman)
| 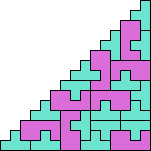
(George Sicherman)
| 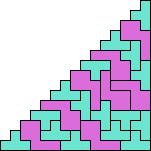
(George Sicherman)
| 
(George Sicherman)
|
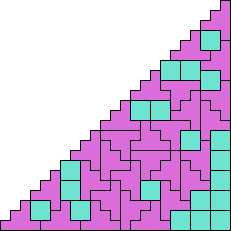
(George Sicherman)
| 
(George Sicherman)
| 
(George Sicherman)
|
|
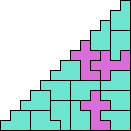
(George Sicherman)
| 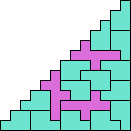
(George Sicherman)
| 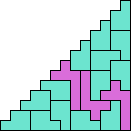
(George Sicherman)
| 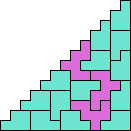
(George Sicherman)
| 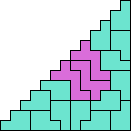
(George Sicherman)
| 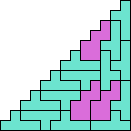
(George Sicherman)
|
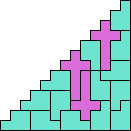
(George Sicherman)
| 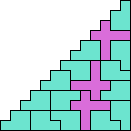
(George Sicherman)
| 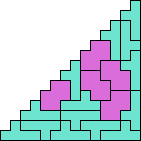
(George Sicherman)
| 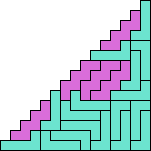
(George Sicherman)
| 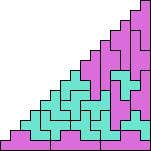
(George Sicherman)
|

(George Sicherman)
| 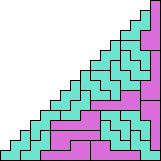
(George Sicherman)
| 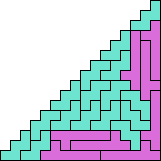
(George Sicherman)
| 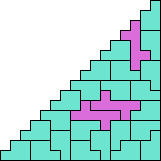
(George Sicherman)
| 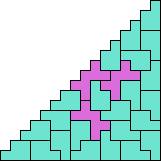
(George Sicherman)
|
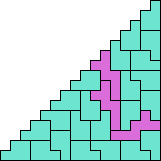
(George Sicherman)
| 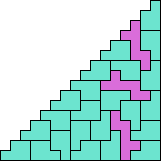
(George Sicherman)
| 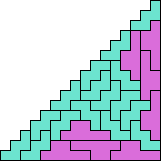
(George Sicherman)
| 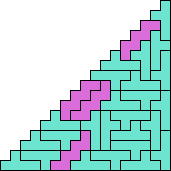
(George Sicherman)
|
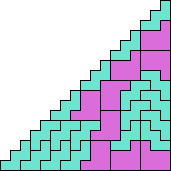
(George Sicherman)
| 
(George Sicherman)
| 
(George Sicherman)
| 
(George Sicherman)
|

(George Sicherman)
| 
(George Sicherman)
| 
(George Sicherman)
|
|
| 6/7
| 
(George Sicherman)
|
|
| 7/7
| 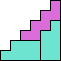
(George Sicherman)
| 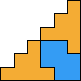
(Patrick Hamlyn)
| 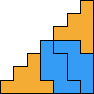
(Patrick Hamlyn)
| 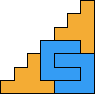
(Patrick Hamlyn)
| 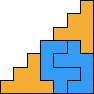
(Patrick Hamlyn)
| 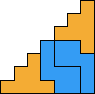
(Patrick Hamlyn)
|

(George Sicherman)
| 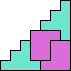
(George Sicherman)
| 
(Bernd Rennhak)
|
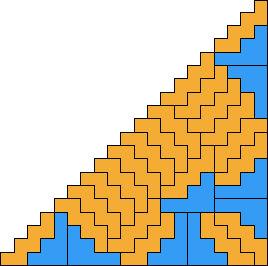
(Patrick Hamlyn)
| 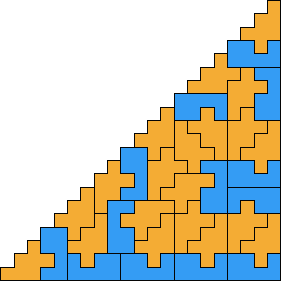
(Patrick Hamlyn)
|

(Bernd Rennhak)
| 
(Bernd Rennhak)
|
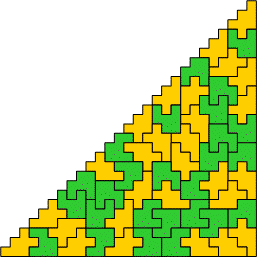
(Bernd Rennhak)
| 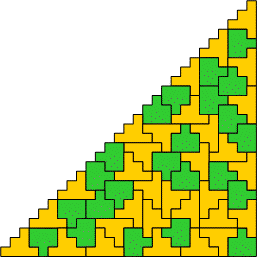
(Bernd Rennhak)
|
(Bernd Rennhak)
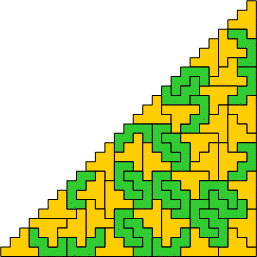
| 
(Bernd Rennhak)
|
|
| 8/8
| 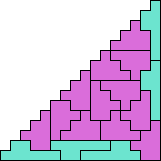
(George Sicherman)
| 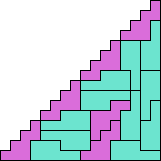
(George Sicherman)
| 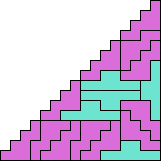
(George Sicherman)
|
|
| 9/9
| 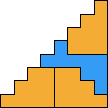
(Patrick Hamlyn)
| 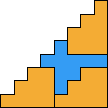
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
| 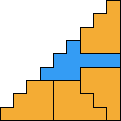
(Patrick Hamlyn)
| 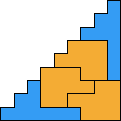
(Patrick Hamlyn)
|
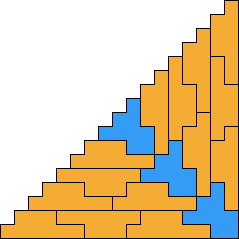
(Patrick Hamlyn)
| 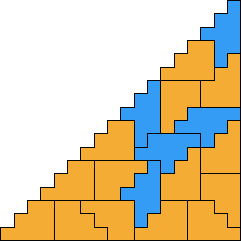
(Patrick Hamlyn)
| 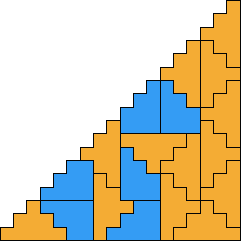
(Patrick Hamlyn)
|
|
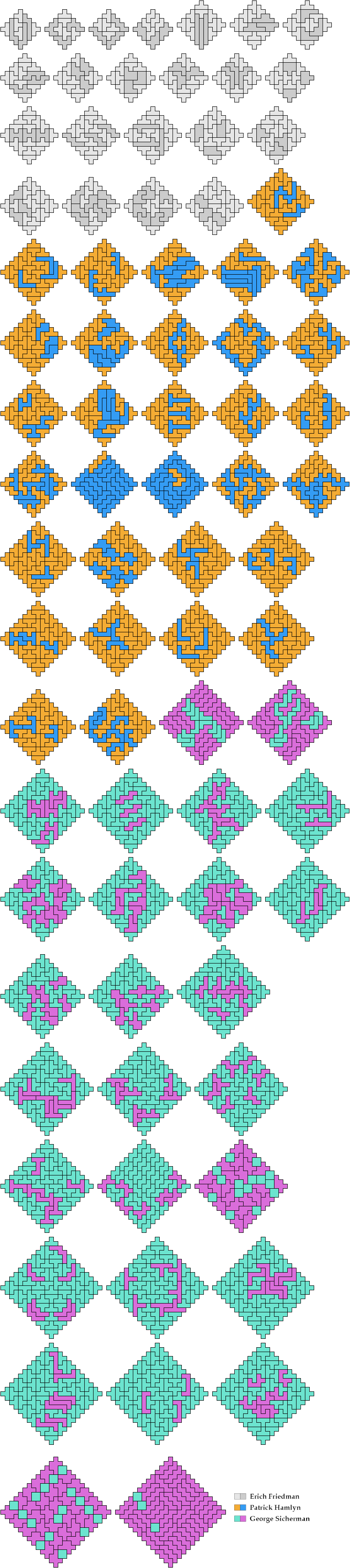
Pyramids
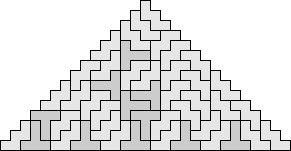
| 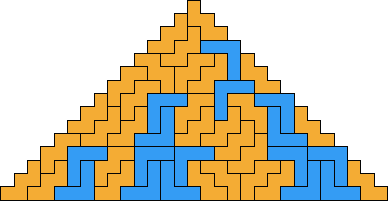
(Patrick Hamlyn)
|
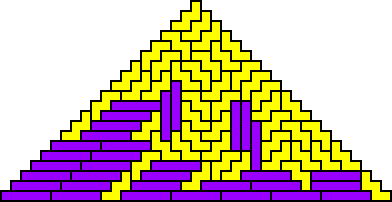
(Mike Reid)
|
|
| 6
| 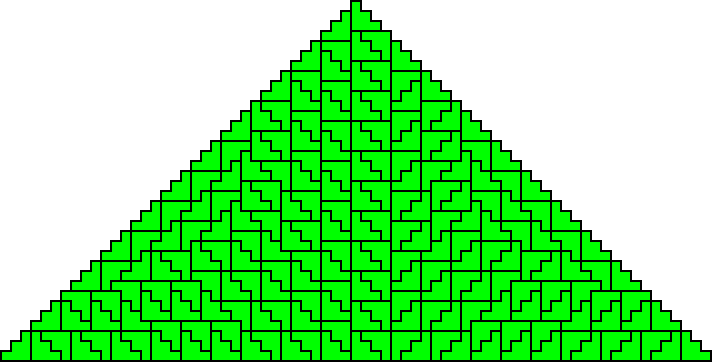
(Mike Reid)
|
|
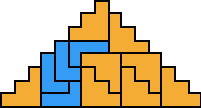
(Patrick Hamlyn)
| 
(Mike Reid)
| 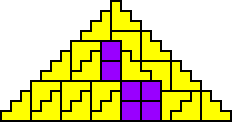
(Mike Reid)
|
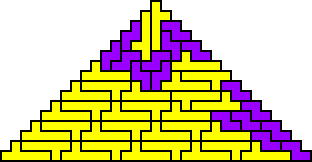
(Mike Reid)
|
|
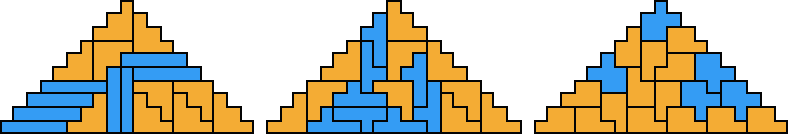
(Patrick Hamlyn)
|

(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
| 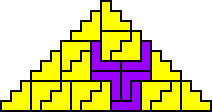
(Mike Reid)
| 
(Mike Reid)
|
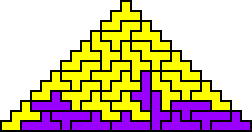
(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
| 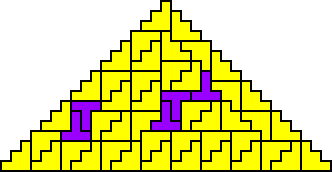
(Mike Reid)
|
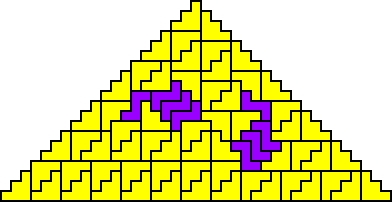
(Mike Reid)
| 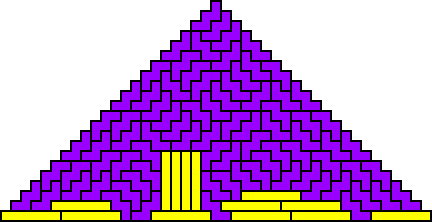
(Mike Reid)
|
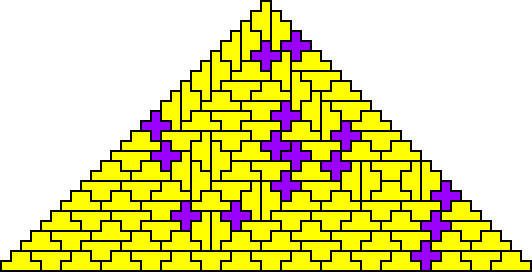
(Mike Reid)
|
|

(Patrick Hamlyn)
| 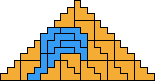
(Patrick Hamlyn)
| 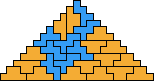
(Patrick Hamlyn)
| 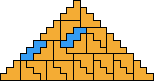
(Patrick Hamlyn)
| 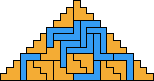
(Patrick Hamlyn)
|
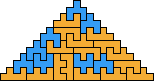
(Patrick Hamlyn)
| 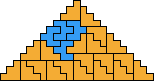
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
|
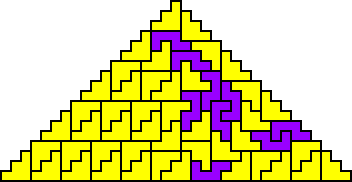
(Mike Reid)
| 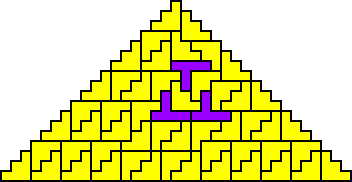
(Mike Reid)
|
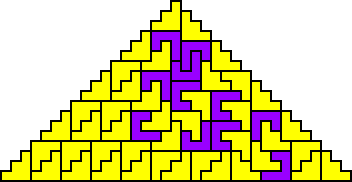
(Mike Reid)
| 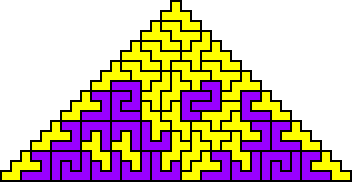
(Mike Reid)
|
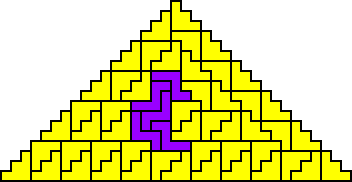
(Mike Reid)
| 
(Mike Reid)
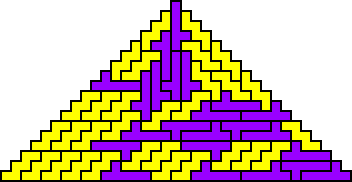
|
(Mike Reid)
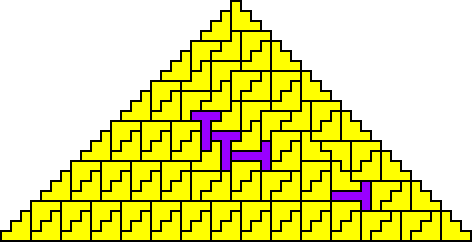
|
(Mike Reid)
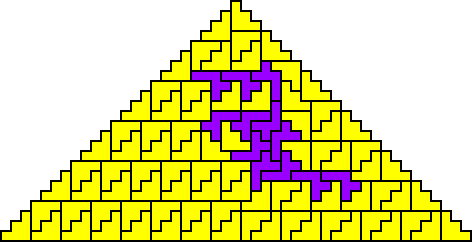
|
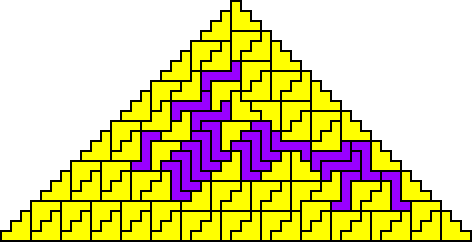
(Mike Reid)
|
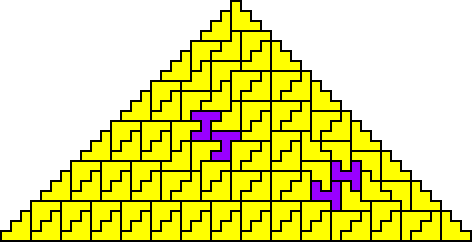
(Mike Reid)
|
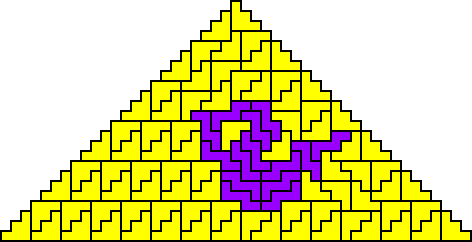
(Mike Reid)
|
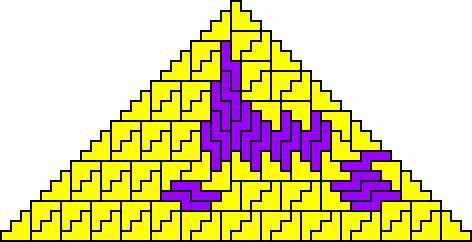
(Mike Reid)
|

(Mike Reid)
|
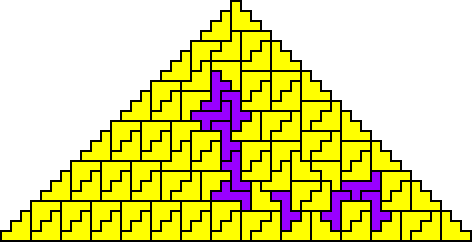
(Mike Reid)
|
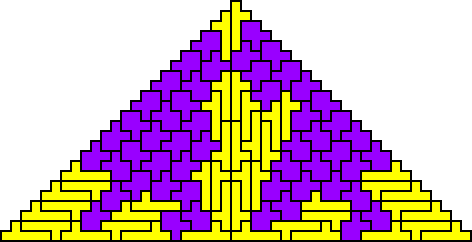
(Mike Reid)
|
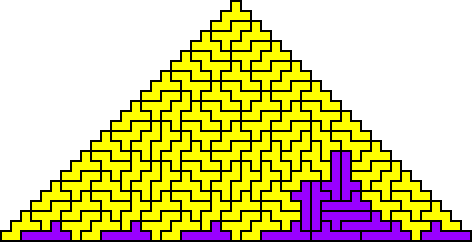
(Mike Reid)
|
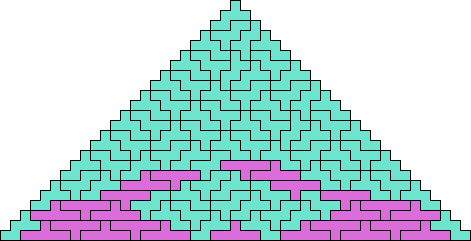
(George Sicherman)
|
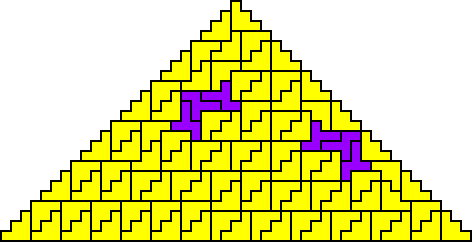
(Mike Reid)
|
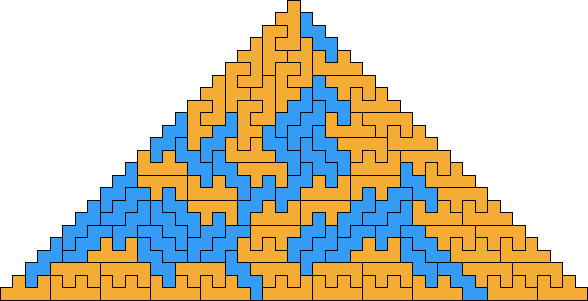
(Patrick Hamlyn)
|
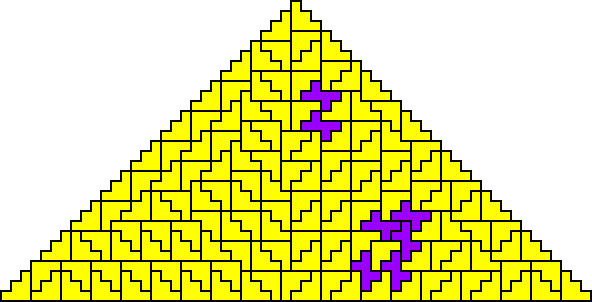
(Patrick Hamlyn)
|
| unknown cases:
| 
| 
|
|

(Patrick Hamlyn)
|

(Patrick Hamlyn)
|
|
| 3/7
| 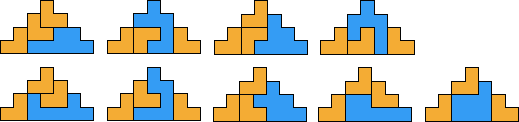
(Patrick Hamlyn)
|
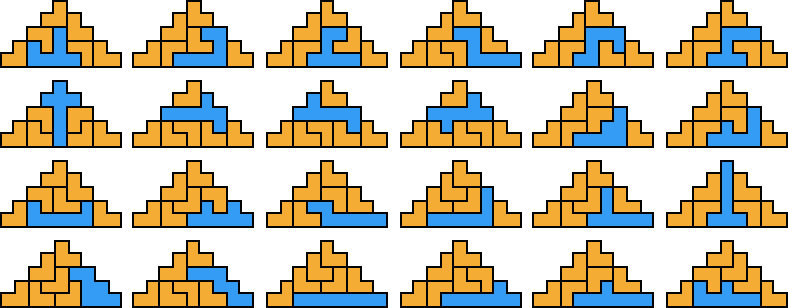
(Patrick Hamlyn)
|
(Patrick Hamlyn)
| 
(George Sicherman)
|
|
| 4/7
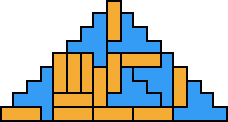
| 
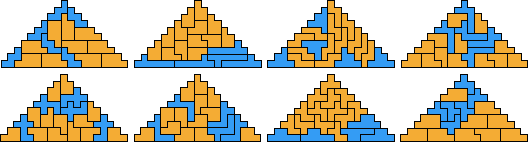
(George Sicherman)
| 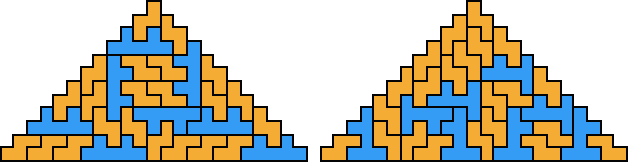
(Patrick Hamlyn)
|
(George Sicherman)
|
|

(Patrick Hamlyn)
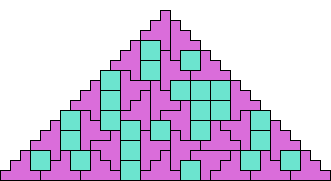
| 
(Patrick Hamlyn)
|
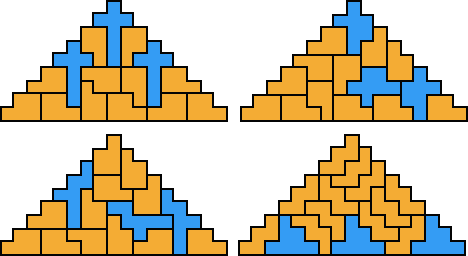
(Patrick Hamlyn)
|
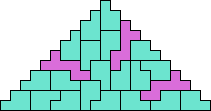
(George Sicherman)
| 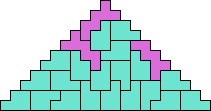
(George Sicherman)
| 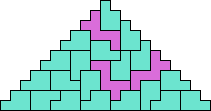
(George Sicherman)
|
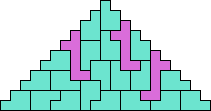
(George Sicherman)
| 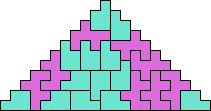
(George Sicherman)
| 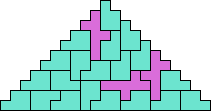
(George Sicherman)
|
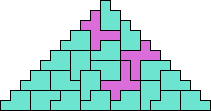
(George Sicherman)
| 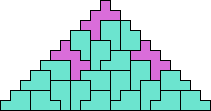
(George Sicherman)
| 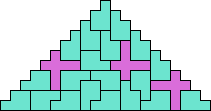
(George Sicherman)
|

(Patrick Hamlyn)
|
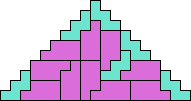
(Patrick Hamlyn)
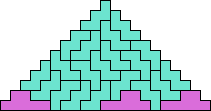
| 
(George Sicherman)
| 
(George Sicherman)
|
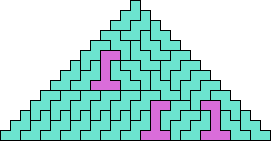
(George Sicherman)
| 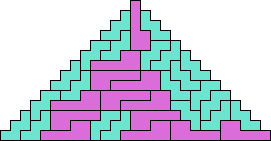
(George Sicherman)
| 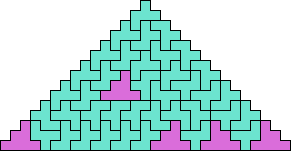
(George Sicherman)
|
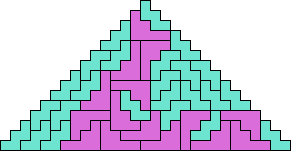
(George Sicherman)
| 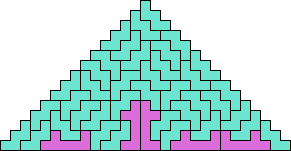
(George Sicherman)
| 
(George Sicherman)
|
|
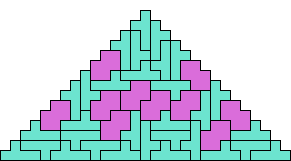
| 6/7
| 
|
|
| 7/7
| 
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
|
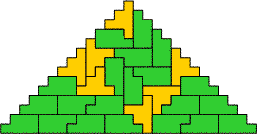
(Bernd Rennhak)
| 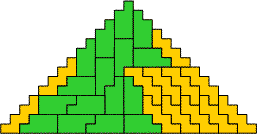
(Bernd Rennhak)
| 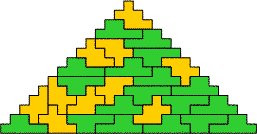
(Bernd Rennhak)
|
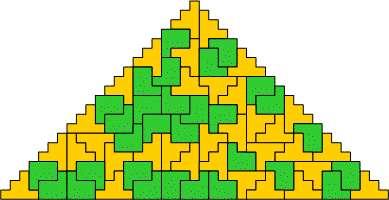
(Bernd Rennhak)
| 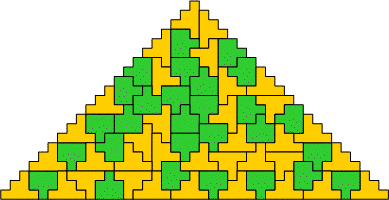
(Bernd Rennhak)
|
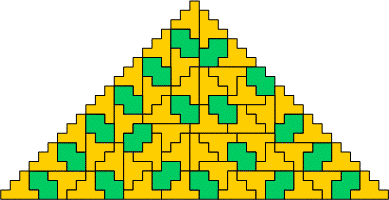
(Bernd Rennhak)
| 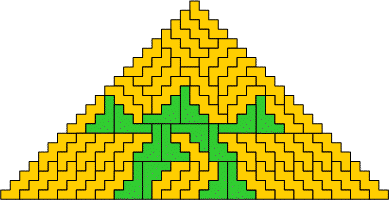
(Bernd Rennhak)
|
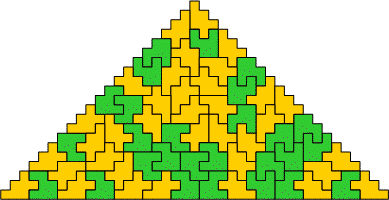
(Bernd Rennhak)
| 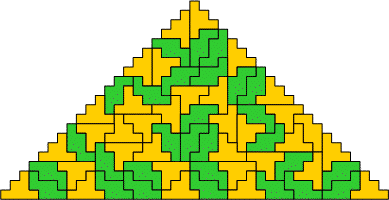
(Bernd Rennhak)
|
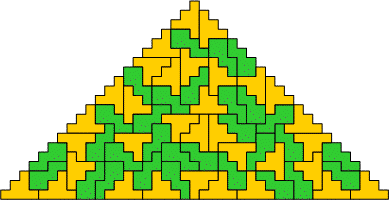
(Bernd Rennhak)
| 
(Bernd Rennhak)
|
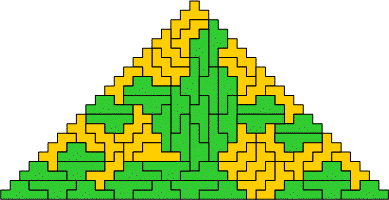
(Patrick Hamlyn)
|
|
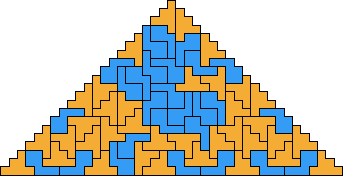
| 8/8
| 
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
|
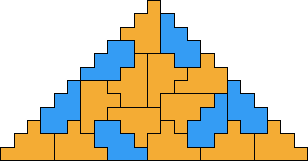
(Patrick Hamlyn)
| 
(Patrick Hamlyn)
|

(Patrick Hamlyn)
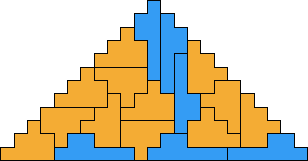
| 
(Patrick Hamlyn)
|
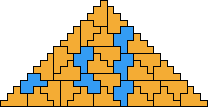
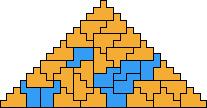
(Patrick Hamlyn)
| 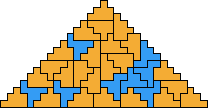
(Patrick Hamlyn)
|
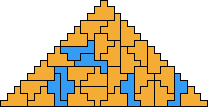
(Patrick Hamlyn)
| 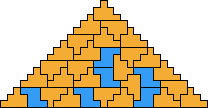
(Patrick Hamlyn)
| 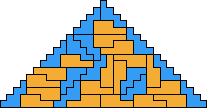
(Patrick Hamlyn)
|
|
| 9/9
| 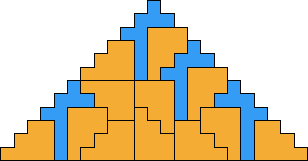
(Patrick Hamlyn)
| 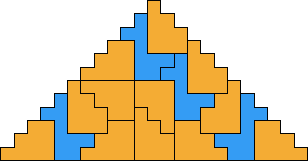
(Patrick Hamlyn)
|
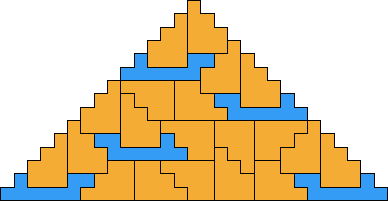
(Patrick Hamlyn)
|
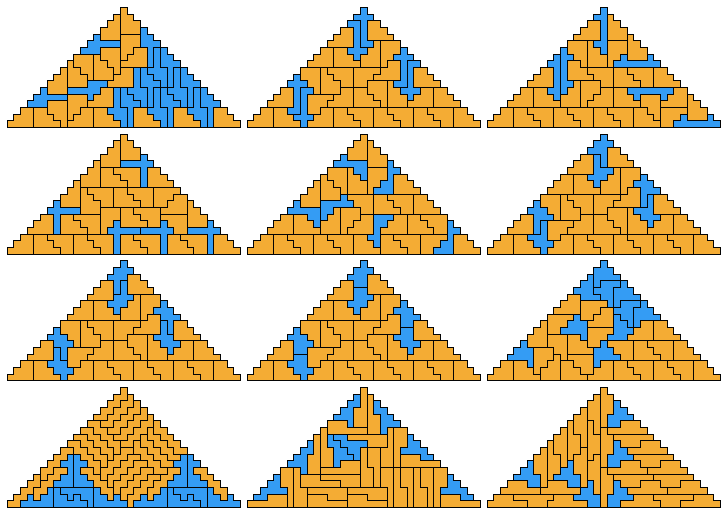
(Patrick Hamlyn)
|
|
Diamonds
| 1
| 
|
|
|
| 2/3
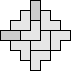
| 
|
|
|
|

(Mike Reid)
| 
(Mike Reid)
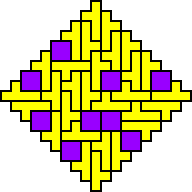
| 
(Mike Reid)
| 
(Mike Reid)
|
|
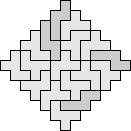
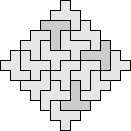
| 
| 
(Bernd Rennhak)
| 
(Bernd Rennhak)
|
|
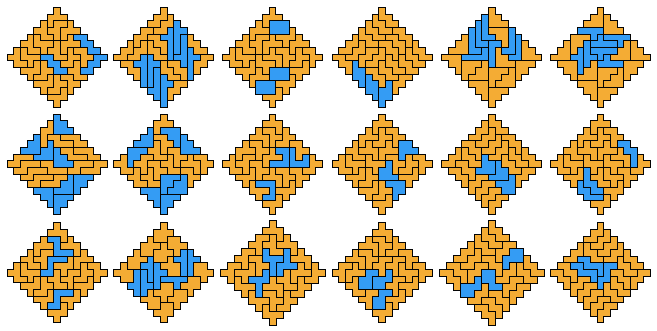
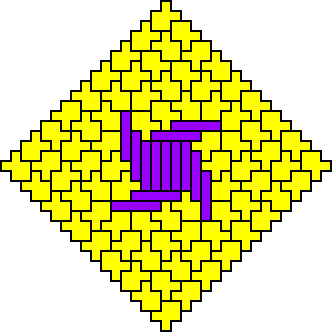
(Patrick Hamlyn)
|

(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
| 
(Mike Reid)
|

(Mike Reid)
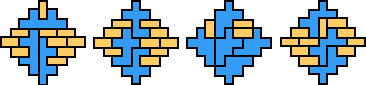
| 
(Mike Reid)
|
|
| 2/7
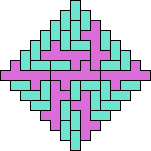
| 
(Patrick Hamlyn)
| 
(George Sicherman)
| 
(George Sicherman)
| 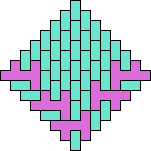
(George Sicherman)
| 
(George Sicherman)
|
|
| 3/7
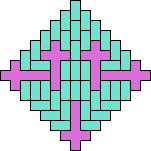
| 
|
|
| 4/7
| 
|
|
| 5/7
| 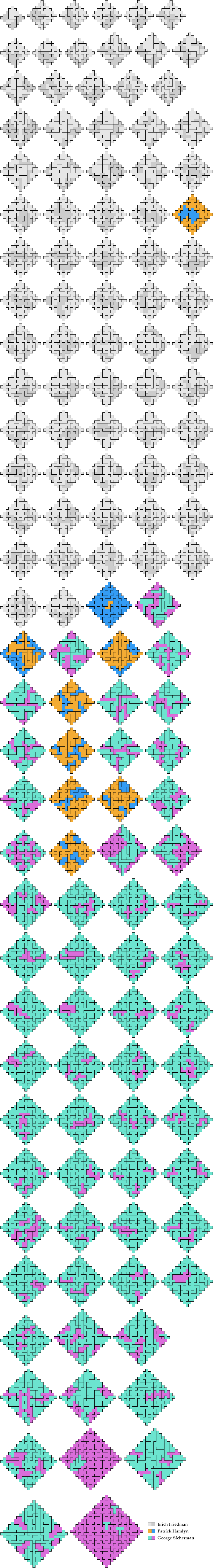
|
|
| 6/7
| 
|
|
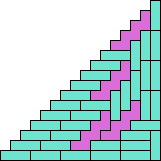
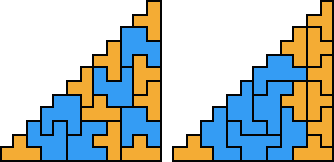
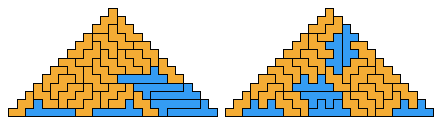
Andrew Clarke was interested in all the triangles that shapes could tile, not just the smallest. He proved that these two shapes can tile a triangle of size 3, and 8 or more:
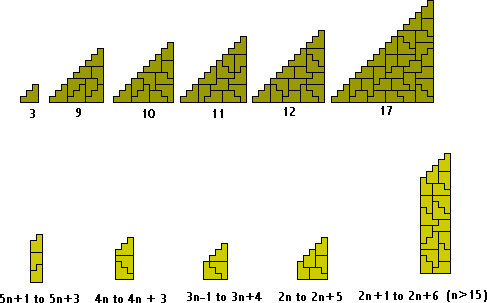
Since some diamonds can be tiled with only one polyomino, we can generalize this problem and look for single polyomino tilings of the smallest possible tilted rectangles. Here is what was discovered a few years ago. Thanks to Mike Reid for making me aware of this progress, and Patrick Hamlyn for sending me the images.

| 
(Jorge Mireles)
| 
(Jorge Mireles)
| 
(Jorge Mireles)
| 
(Jorge Mireles)
| 
(Dario Uri)
| 
(Jorge Mireles)
|

(Jorge Mireles)
| 
(Patrick Hamlyn)
| 
(Jorge Mireles)
| 
(Jorge Mireles)
| 
(Jorge Mireles)
| 
(Jorge Mireles)
|
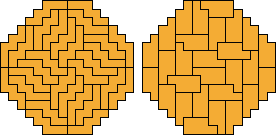
Patrick Hamlyn decided to look for the smallest tilings of octagons by one polyomino. His results are shown below.
If you can extend any of these results, please
e-mail me.
Click here to go back to Math Magic. Last updated 8/30/10.