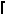 log2
log2 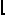 log10 n
log10 n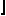
 palindromes.
palindromes.
Brendan Owen and Joseph DeVincentis found that if exponentiation is allowed, extremely large palindromes like 1+(1+9)99 could be formed.
Here are the largest palindromes that we can create using a small number of digits. There is still a lot of work to be done here.
| Number of Digits | Calculation | Palindrome | Author |
|---|---|---|---|
| 1 | 9 | 9 | Trivial |
| 2 | 9+2 | 11 | Trivial |
| 3 | 7×7×7 | 343 | Proved by Erich Friedman, 1999 |
| 4 | 9 × (9×9+2) | 747 | Proved by Matt Galati, 1999 |
| 5 | 9×9×9×9–5 | 6556 | Proved by Erich Friedman, 2002 |
| 6 | 3×7×7×8×8×8 | 65856 | Proved by Erich Friedman, 2002 |
| 7 | 8×8×8×8×9 × (9+2) | 405504 | Proved by Erich Friedman, 2002 |
| 8 | 8×9×9×9×9×9×9–4 | 4251524 | Proved by Erich Friedman, 2002 |
| 9 | 8×8 × (5×6×8×8×8×9+2) | 8847488 | Proved by Erich Friedman, 2007 |
| 10 | 7×7×7×8 × (7×8×8×8×9–4) | 88499488 | Found by Erich Friedman, 2004 |
| 11 | 6×8×8×8×9 × (6×7×9×9×9+8) | 846747648 | Found by Erich Friedman, 2007 |
| 12 | 7×8×8×8×8×9 × (4×7×7×9×9–3) | 4095995904 | Found by Erich Friedman, 2004 |
| 13 | 9×9×9×9×9×9 × (6×7×7×7×7×9–8) | 68899199886 | Found by Marc Lapierre, 2017 |
| 14 | (9×9×9×9×9×5+4) × (8×8×8×8×9×9–5) | 97955055979 | Found by Marc Lapierre, 2017 |
| 15 | 7×8 × (5×9×9×9×9×9–7) × (8×9×9×9×9–7) | 867685586768 | Found by Marc Lapierre, 2017 |
| 16 | 9×8×8×8×8×8×8×7×4 × (7×7×7×7×7×8–5) | 8881871781888 | Found by Marc Lapierre, 2017 |
| 17 | 8×8×8×8×8×9 × (9×9×7+2) × (9×9×9×9×8×7+6) | 61655222255616 | Found by Marc Lapierre, 2017 |
| 18 | 9×8×8 × (8×8×8×8×8×7+1) × (9×9×9×9×9×9×9–1) | 631931242139136 | Found by Marc Lapierre, 2017 |
| 19 | 9×8×8 × (6×8×8×8×8×8×8×9×9+5) × (7×8×8×8×9+7) | 2367573333757632 | Found by Marc Lapierre, 2017 |
| 20 | 9×9×7 × (8×8×8×8×8×9×9×9×9+3) × (6×9×9×9×9×9+8) | 43189347374398134 | Found by Marc Lapierre, 2017 |
| 21 | 7×9 × (8×8×8×8×8×9×9×9+5) × (2×8×8×9×9×9×9×9×9+3) | 86925062526052968 | Found by Marc Lapierre, 2017 |
| 22 | 7×9×9×9 × (6×9×9×9×9×9×9×9×9+3) × (7×7×7×7×9×9×9+7) | 2306950757570596032 | Found by Marc Lapierre, 2017 |
| 23 | 9 × (8×8×8×9×9×9×9×9×9×9–1) × ((7×9×9–2)×9×9×9×9×9×9–3) | 6617798452548977166 | Found by Marc Lapierre, 2017 |
| 24 | 9×9×9×9×9 × (7×9×9×9×9×9×9–8) × ((6×8×8×8×8×8+5)×8×9×9+1) | 9539972462642799359 | Found by Marc Lapierre, 2017 |
No one has yet found any other palindromes which are the product of 3 or more consecutive integers. Patrick De Geest showed that there are no such products less than 6×1029. Ulrich Schimke searched products of 3 consecutive integers up to 8×1015. Andrew Bayly searched for products up to 109.
Brendan Owen explained why no palindrome is the product of 5 or more consecutive integers: because one of these will be divisible by 5, and another will be divisible by 2, making the palindrome end in 0, an impossibility. Using similar reasoning, he showed that any palindrome which is the product of 4 consecutive integers ends in 4, and any palindrome which is the product of 3 consecutive integers ends in 4 or 6. Bayly also made the latter observation.
Joseph DeVincentis showed that any palindrome which is the product of 4 consecutive integers ends in "024".
Several similar questions are investigated at this page of Patrick De Geest.
Let P(a,b) be the smallest palindrome in base a and b.
Ulrich Schimke showed that P(a,a+1)=2a2+3a+2 for a≥4. The sequence P(a,a+1): 6643, 10, 46, 67, 92, 121, . . . is now sequence A048268 of the Encyclopedia of Integer Sequences.
Schimke also showed that P(a,a+2)=(3a2+6a+2)/2 for even a≥8, and (a2+4a+3)/2 for odd a≥5. The sequence P(a,a+2): 5, 26, 21, 24, 154, 40, 121, 60, 181, 84, 253, 112, . . . is now sequence A048269 of the Encyclopedia of Integer Sequences. He believes that there are polynomials for every P(a,a+n).
Joseph DeVincentis and Ulrich Schimke note that if b is a power of a, then b+1 is a palindrome in base a and b. Schimke notes that this is enough to show that for every n, there is a number which is a palindrome in at least n different bases. Schimke also generalizes this to note that if b is a palindrome in base a, then the smallest palindrome in bases a and b-1 is b.
Matt Galati gave the smallest palindromes in bases a and b for a,b≤35. DeVincentis did this for a,b≤10. Schimke points out the largest P(a,b) for a,b≤35 is P(7,35)=16008.
It is surprising that P(2,3)=6643 is so large. DeVincentis has some explanations for this. Schimke has calculated that the only numbers less than 4.5×107 that palindromic in base 2 and 3 are 6643, 1422773, and 5415589.
Here are the smallest palindromes in two given bases:
| a \ b | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 6643 | |||||||||||||||||
| 4 | 5 | 10 | ||||||||||||||||
| 5 | 31 | 26 | 46 | |||||||||||||||
| 6 | 7 | 28 | 21 | 67 | ||||||||||||||
| 7 | 85 | 8 | 85 | 24 | 92 | |||||||||||||
| 8 | 9 | 121 | 63 | 18 | 154 | 121 | ||||||||||||
| 9 | 127 | 10 | 10 | 109 | 80 | 40 | 154 | |||||||||||
| 10 | 33 | 121 | 55 | 88 | 55 | 121 | 121 | 191 | ||||||||||
| 11 | 255 | 244 | 255 | 12 | 166 | 24 | 36 | 60 | 232 | |||||||||
| 12 | 65 | 13 | 65 | 26 | 104 | 78 | 65 | 91 | 181 | 277 | ||||||||
| 13 | 313 | 28 | 42 | 98 | 14 | 235 | 154 | 70 | 222 | 84 | 326 | |||||||
| 14 | 15 | 1210 | 15 | 408 | 135 | 135 | 45 | 30 | 323 | 60 | 253 | 379 | ||||||
| 15 | 693 | 16 | 1265 | 1612 | 80 | 16 | 316 | 80 | 828 | 48 | 241 | 112 | 436 | |||||
| 16 | 17 | 68 | 17 | 119 | 385 | 85 | 170 | 136 | 353 | 221 | 1172 | 170 | 337 | 497 | ||||
| 17 | 341 | 784 | 341 | 18 | 1211 | 307 | 18 | 528 | 252 | 36 | 290 | 126 | 90 | 144 | 562 | |||
| 18 | 325 | 1733 | 38 | 57 | 209 | 57 | 325 | 209 | 171 | 133 | 1462 | 209 | 323 | 361 | 433 | 631 | ||
| 19 | 381 | 20 | 514 | 428 | 80 | 40 | 260 | 20 | 666 | 60 | 362 | 140 | 60 | 80 | 514 | 180 | 704 | |
| 20 | 21 | 1604 | 21 | 126 | 21 | 1944 | 63 | 273 | 252 | 84 | 761 | 42 | 105 | 421 | 273 | 126 | 541 | 781 |
Joseph DeVincentis and Ulrich Schimke proved that there are infinitely many numbers which are not the sum of two palindromes, namely 2×10n+1 for n≥1. Each of these is too small to be the sum of two palindromes of length n+1, and too large to be the sum of two palindromes of length n, so can only be the sum of a palindrome of length n and a palindrome of length n+1 starting and ending with 1. But this means the palindrome of length n must end in 0, an impossibility.
The sequence of positive integers which are not the sum of two palindromes: 21, 32, 43, 54, 65, 76, 87, 98, 201, 1031, 1041, 1042, 1051, 1052, 1053, 1061, 1062, 1063, 1064, 1071, 1072, 1073, 1074, 1075, 1081, 1082, 1083, 1084, 1085, 1086, 1091, 1092, 1093, 1094, 1095, 1096, 1097, 1099, 1101, 1103, 1104, 1105, 1106, 1107, 1108, . . . is sequence A035137 of the Encyclopedia of Integer Sequences.
John Hoffman searched for numbers that were not the sum of 3 palindromes. He found none less than 9×108. He also notes all sufficiently large numbers seem to be the sum of 3 palindromes, one of which is the biggest or second biggest possible.
Hoffman notes that an integer n is the sum of at most
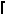 log2
log2 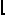 log10 n
log10 n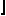
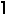 palindromes.
palindromes.
Hoffman also asks the following question: For what values of θ does ∑ nθ converge, where the sum is over palindromes n? The answer is for θ<–½.
DeVincentis showed that if a positive integer n with d digits is expressible as the difference of two palindromes, it is expressible as the difference of two palindromes each having no more than 2d-1 digits. He also showed that the smallest number that is not the difference of palindromes is 1020.
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 10/12/17.