Problem of the Month (July 2001)

Call a tiling of the plane an n-neighbor tiling if every tile has the same number of neighbors, tiles that touch the tile in at least one point. For example, the usual square tiling of the plane is an 8-neighbor tiling, but there are also 7-neighbor tilings and 6-neighbor tilings of the plane using squares. The question is this: among all n-neighbor tilings for a fixed value of n, what is the fewest number of different tiles needed?

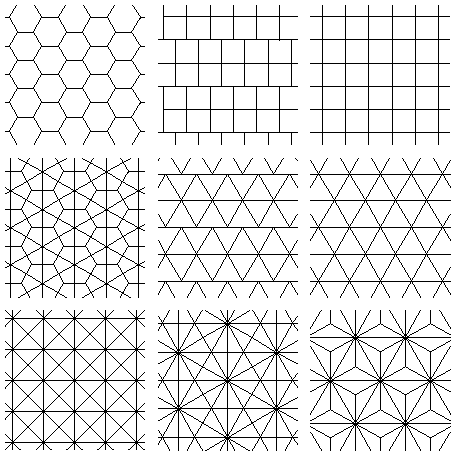
For example, there are monohedral tilings, tilings which use congruent copies of one tile only, which are n-neighbor tilings for n=6, 7, 8, 9, 10, 12, 14, 16, and 21. Can you find them? Are these the only ones? For dihedral tilings, tiling using two different tiles, n-neighbor tilings exist for n=11, 13, and 15. Can you find these? Are there any others?

ANSWERS

Joseph DeVincentis, Andrew Bayly, and Berend Jan van der Zwaag found monohedral n-neighbor tilings for n=6, 7, 8, 9, 10, 12, 14, 16, and 21. Such tilings are shown below.

Here are the dihedral 11-neighbor and 13-neighbor tilings.

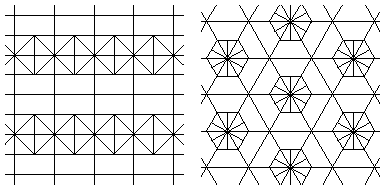
And here is my recently discovered dihedral 15-neighbor tiling. Berend Jan van der Zwaag found it too.

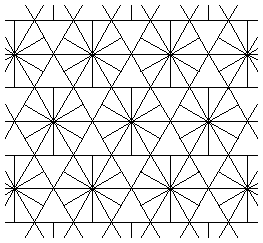
Berend Jan van der Zwaag also found dihedral tilings with 32 and 43 neighbors respectively.

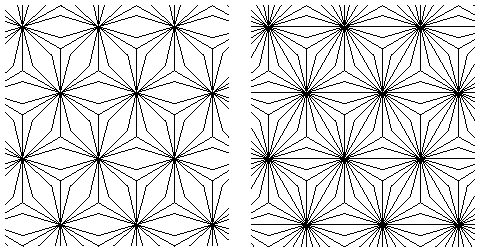
Of course by using the same trick we can get a k-hedral (22k–1)-neighbor tiling, and a k-hedral (22k-12)-neighbor tiling for all k. This led to us publishing a joint paper, that can be found here.

If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 8/12/01.