Problem of the Month (July 2003)

This month's problem concerns stacking L's. An L of size n is bent triomino, scaled up by a factor of n so that its area is 3n2. We are interested in stacking copies of L's of side 1 through n so that each L is fully supported below, and the stack is as high as possible. For example, we can stack L's of side 1 through 6 so that the stack is 26 units high:

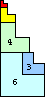
Can you find a way to stack L's of side 1 through 10 so that the stack is 56 units high? What is the highest stack of L's you can create with other numbers of L's?

ANSWERS

Bob Wainwright found the solution for n=10. Andrew Bayly found optimal configurations for n=10 through n=18. Philippe Fondanaiche made conjectures for 6≤n≤32.

Two people found solutions better than the ones I had found. Erika Brandner found configurations for n=5 and n=7. Clinton Weaver found configurations for n=9 and n=11.

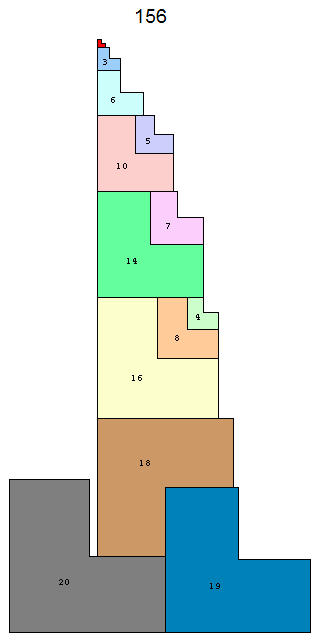
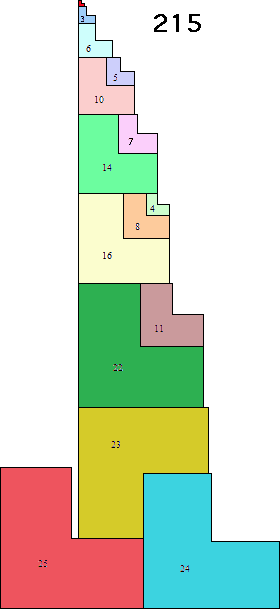
In late 2003, Clinton Weaver made a breakthrough and improved almost all the best known L stacks. His best are shown below.

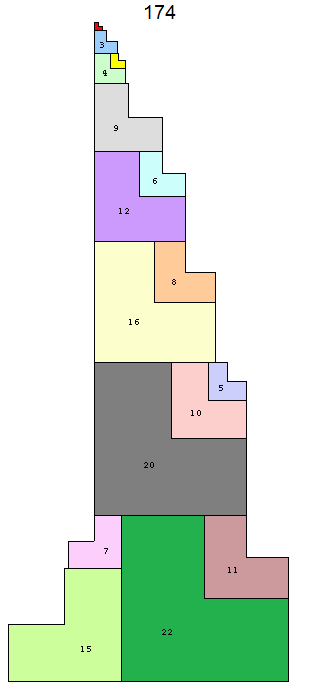
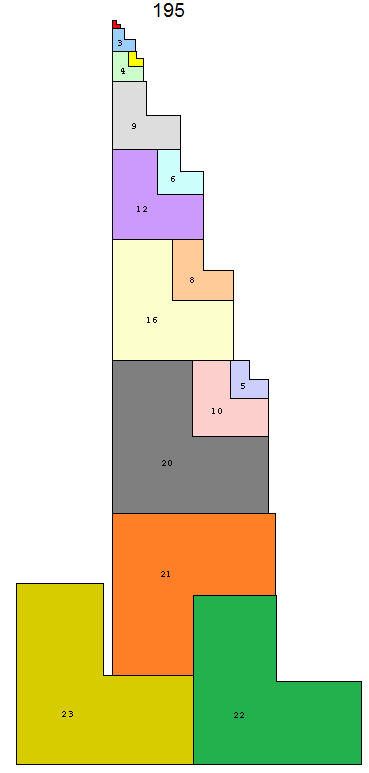
In 2011, Glisic Vedran extended the solutions up to n=25.

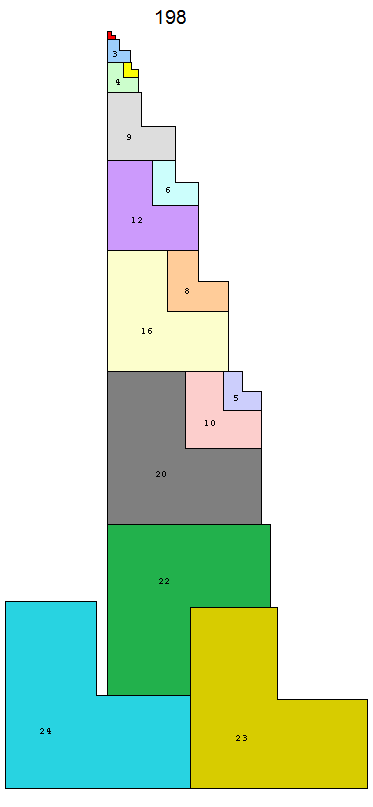
In 2012, Maurizio Morandi improved n=19, 21, and 25.

2
6
8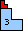
16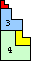
18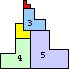
26
33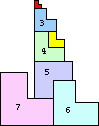
38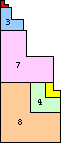
49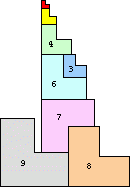
56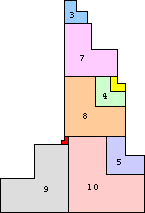
67
70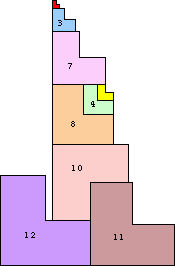
89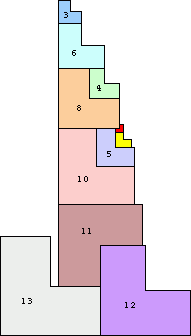
92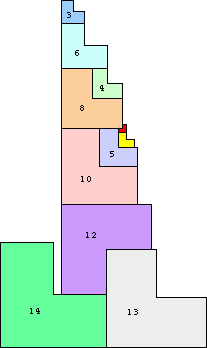
109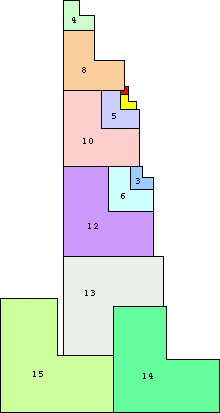
112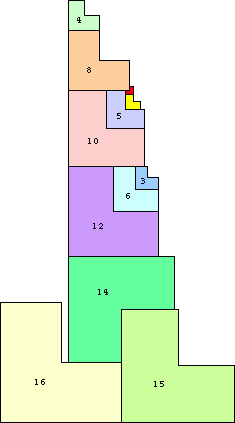
129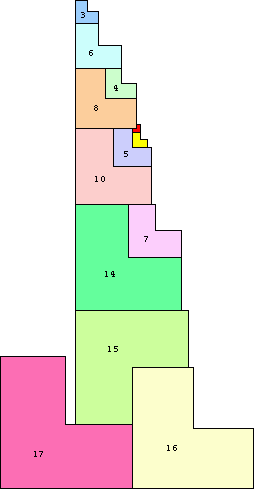
132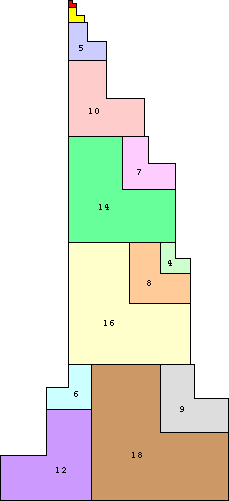

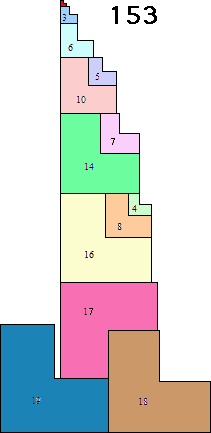
Another interesting problem is the tallest stack that can be made from consecutive L's if each L needs to be supported on both extremes, but not necessarily everywhere. Here is the best I could do:

2
6
8
16
22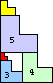
30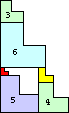
38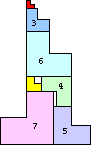
50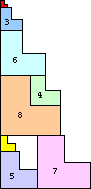
58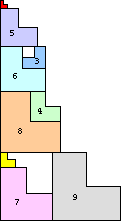
74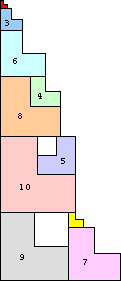
84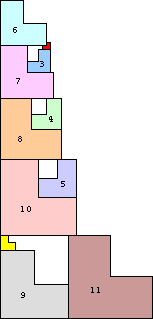
104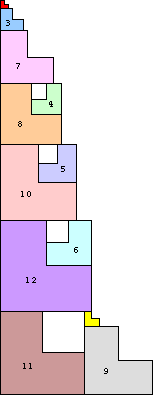

If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 10/30/11.