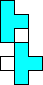Problem of the Month (July 2008)
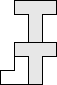
Given several copies of a polyomino P, and a polyomino frame F, define pack(P,F) to be the maximum number of copies of P that can be packed without overlap inside F. Given a polyomino P, what is the smallest frame F, so that for some positive integer n, P is the only polyomino with pack(P,F)=n ? In other words, how small can the frame be so that giving the maximum number of copies of a polyomino that fit uniquely determines the polyomino? Are the frames shown below the smallest ones? What about larger polyominoes?
ANSWERS
Here are the smallest known solutions:
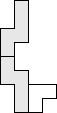
Small Polyominoes

| 
| 
| 
| 
| 
| 
(George
Sicherman)
| 
(George
Sicherman)
| 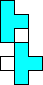
(George
Sicherman)
|
|
Pentominoes
| 
| 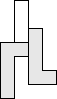
| 
(George
Sicherman)
| 
(George
Sicherman)
| 
(George
Sicherman)
|
|
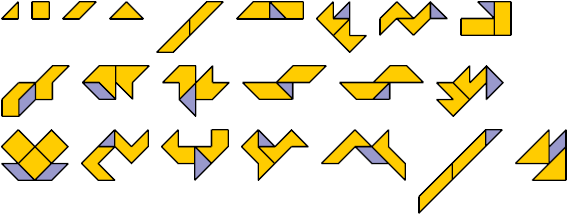
George Sicherman found these polyiamond, polyhex, and polyabolo solutions:
Polyiamonds
Polyhexes
Polyaboloes
If you can extend any of these results, please
e-mail me.
Click here to go back to Math Magic. Last updated 7/1/08.