Problem of the Month (July 2010)

The problem of packing squares of sides 1-n into the smallest square is well-known. Recently the problem of packing these squares into the smallest rectangle has also been studied. This month we study how to pack these squares into the smallest circle, and the smallest isosceles right triangle. What are the best packings for various n?

ANSWERS

Smallest Known Circles Containing Squares of Sides 1-n

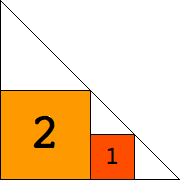
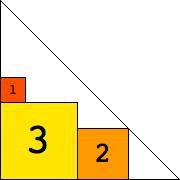
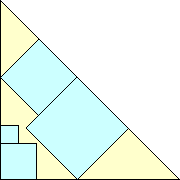
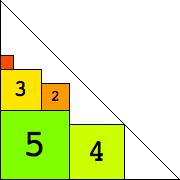
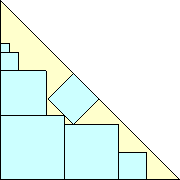
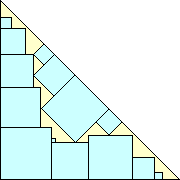
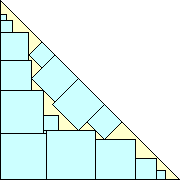
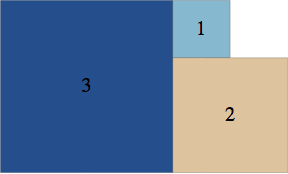
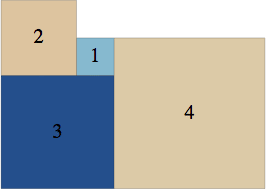
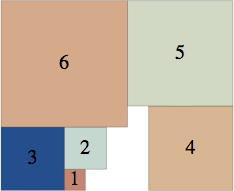
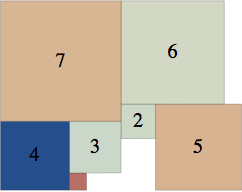
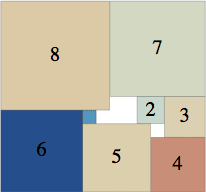
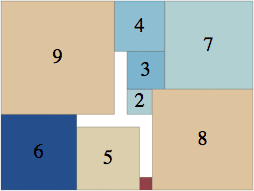
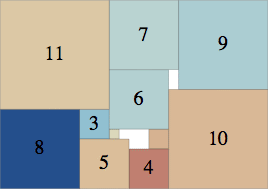
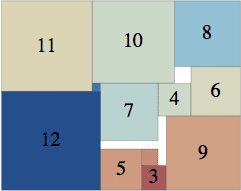
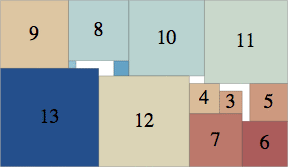
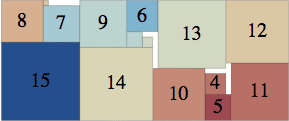
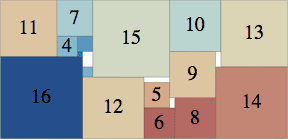
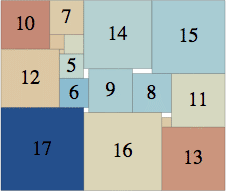
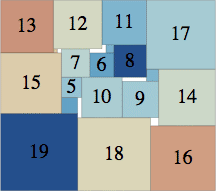
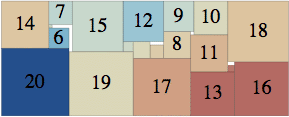
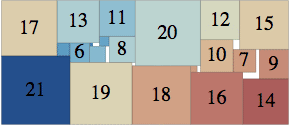
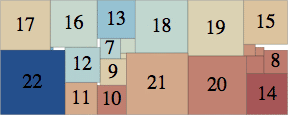
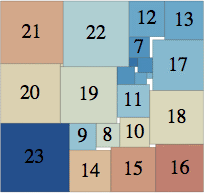
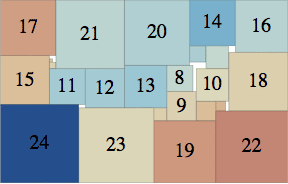
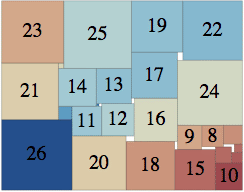
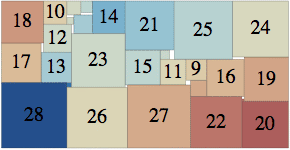
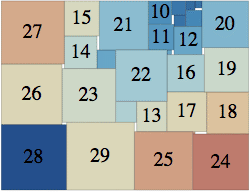
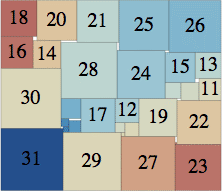
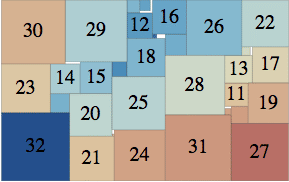
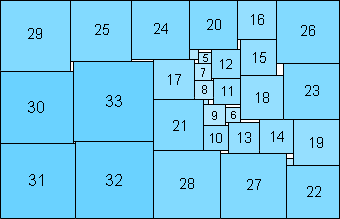
r = √2 / 2

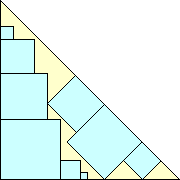
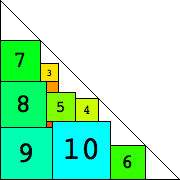
r = √185 / 8

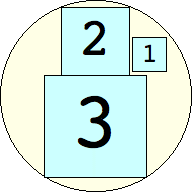
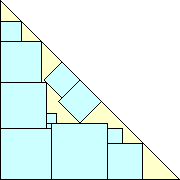
r = √505 / 8

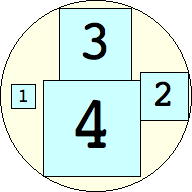
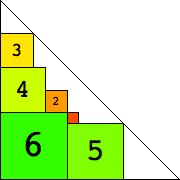
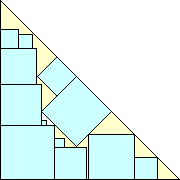
r = 3.967+
(Maurizio Morandi)

 

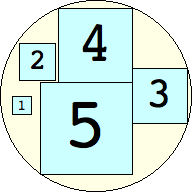
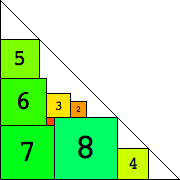
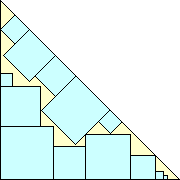
r = 5.193+
(Maurizio Morandi)

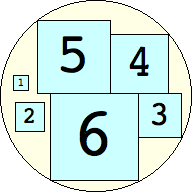
r = 6.553+

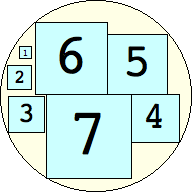
r = 7.935+

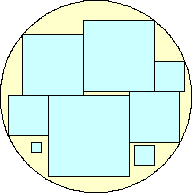
r = 9.475+
(Maurizio Morandi)

 

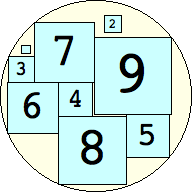
r = 11.216+
(Johan de Ruiter)

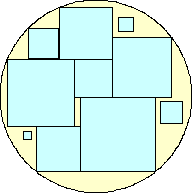
r = 12.869+
(Maurizio Morandi)

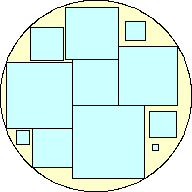
r = 14.513+
(Maurizio Morandi)

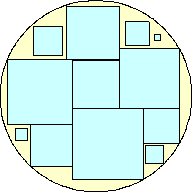
r = 16.161+
(Maurizio Morandi)

 

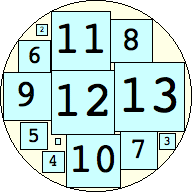
r = 17.924+
(Johan de Ruiter)

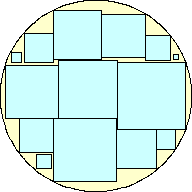
r = 19.670+
(Maurizio Morandi)

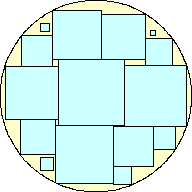
r = 21.631+
(Maurizio Morandi)

Smallest Known Isosceles Right Triangles Containing Squares of Sides 1-n

s = 2

s = 4

s = 7

s = 7√2
(Maurizio Morandi)

 

s = 13

s = 16

s = 14 + 4√2
(Maurizio Morandi)

s = 23

 

s = 7 + 14√2
(Maurizio Morandi)

s = 31

s = 28 + 5√2
(Maurizio Morandi)

s = 24 + 11√2
(Maurizio Morandi)

 

s = 44
(Maurizio Morandi)

s = 19 + 21√2
(Maurizio Morandi)

s = 10 + 31√2
(Maurizio Morandi)

s = 43 + 11√2
(Maurizio Morandi)

 

s = 15 + 69/√2
(Maurizio Morandi)

s = 51 + 13√2
(Maurizio Morandi)

Ed Pegg sent optimal solutions for squares in rectangles that he gathered from other sources. What are solutions for larger n?

Smallest Rectangles Containing Squares of Sides 1-n

 
 
 
 
 
 
 
 
 
 

(Maurizio Morandi)

If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 7/10/10.