Problem of the Month (July 2012)

Define an n-sliced square as a unit square with n consecutive corners (right isosceles triangles with area 1/8) cut off. What are the smallest squares that some number of n-sliced squares can be packed into? What about n-sliced squares packed into n-sliced squares? What about squares packed into n-sliced squares?

ANSWERS

Here are the best-known packings:

1-Sliced Squares in Squares
1

s = 1 		2

s = 3/2 = 1.5 		3

s = 3(1+√2)/4 = 1.810+ 		4

s = 2
5

s = (3+√2)/2 = 2.207+
  		6

s = 5/2 = 2.5
  		7

s = 2.700+
(DC after MM) 		8

s = 2√2 = 2.828+
(Haym Hirsh)
9

s = 3
  		10

s = 3.174+
(David W. Cantrell) 		11

s =(50+21√2)/24= 3.320+
(Maurizio Morandi) 		12

s =(29+√31)/10= 3.456+
(David W. Cantrell)
13

s=(52+19√2)/22=3.585+
(Maurizio Morandi) 		14

s = 3 + 1/√2 = 3.707+
(Maurizio Morandi) 		15

s=(66+19√2)/24=3.869+
(Maurizio Morandi) 		16

s = (6+7√2)/4 = 3.974+
(Maurizio Morandi)

1-Sliced Squares in 1-Sliced Squares
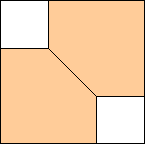
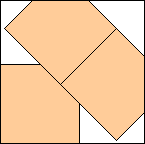
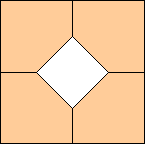
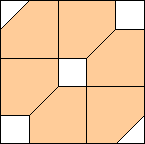
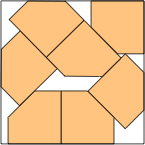
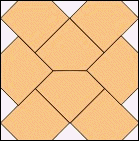
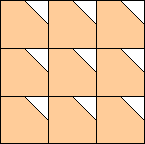
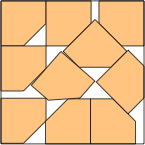
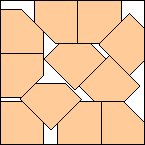
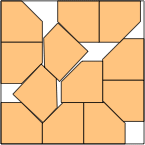
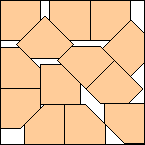
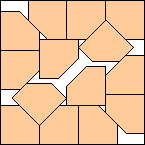
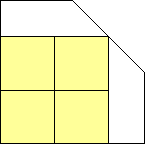
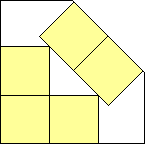
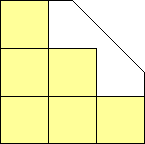
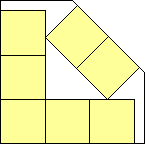
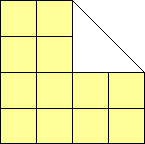
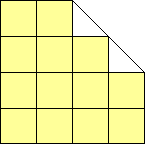
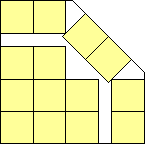
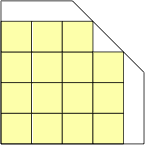
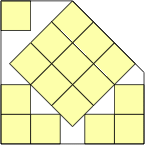
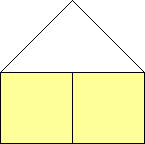
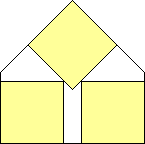
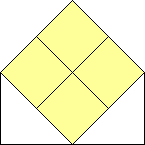
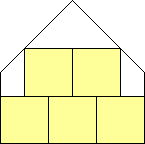
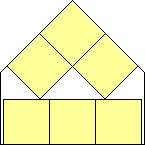
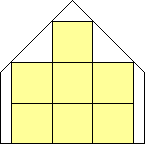
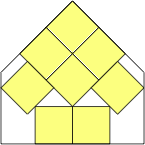
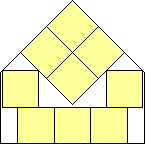
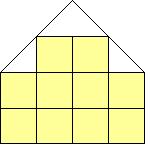
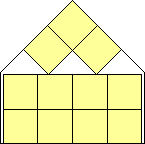
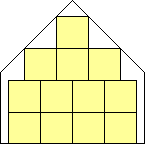
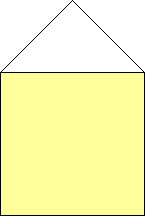
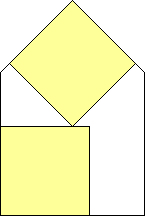
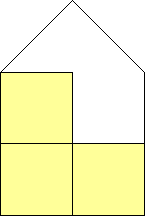
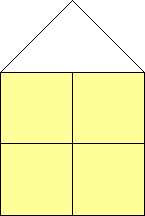
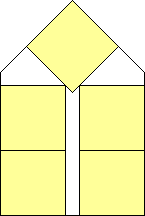
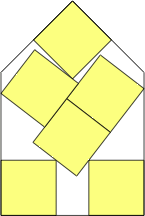
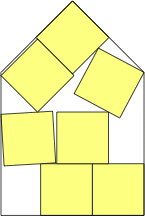
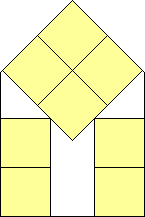
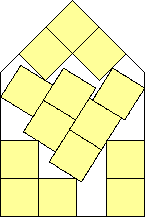
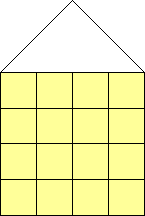
1

s = 1 		2

s = 5/3 = 1.666+ 		3

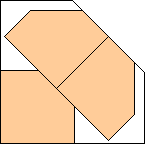
s = 1+2√2/3 = 1.942+ 		4

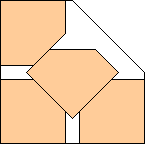
s = (3+√2)/2 = 2.207+
5

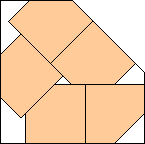
s = 1 + √2 = 2.414+
(Maurizio Morandi) 		6

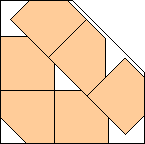
s = 8/3 = 2.666+
  		7

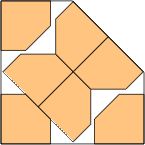
s = 1+4√2/3 = 2.885+
(David W. Cantrell) 		8

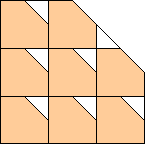
s = 3
 
9

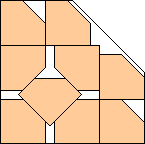
s = (5+√2)/2 = 3.207+
(Bryce Herdt) 		10

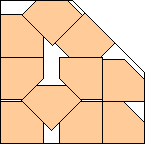
s=3(9+4√2)/13=3.382+
(Maurizio Morandi) 		11

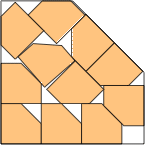
s = 3.550+
(David W. Cantrell) 		12

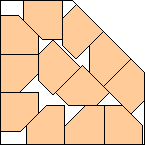
s =(19+5√2)/7= 3.724+
(Maurizio Morandi)
13

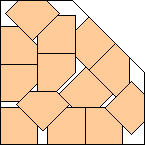
s=(59+37√2)/29=3.838+
(Maurizio Morandi) 		14

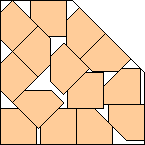
s=(36+31√2)/20=3.992+
(Maurizio Morandi) 		15

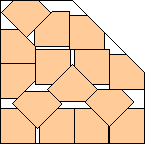
s=(61+21√2)/22=4.122+
(Maurizio Morandi) 		16

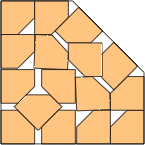
s = 4.247+
(David W. Cantrell)

Squares in 1-Sliced Squares
1

s = 4/3 = 1.333+ 		2

s = 2 		3

s = 2 		4

s = 8/3 = 2.666+
5

s = 2 + 2/√3 = 2.942+
(Maurizio Morandi) 		6

s = 3
  		7

s = 4(1+√2)/3 = 3.218+
  		8

s = 10/3 = 3.333+
 
9

s = 2+5√2/4 = 3.767+
  		10

s = 2+4√2/3 = 3.885+
(Maurizio Morandi) 		11

s = 4
  		12

s = 4
 
13

s = 4
  		14

s = 3 + √2 = 4.414+
  		15

s = 14/3 = 4.666+
(David W. Cantrell) 		16

s = 2 + 2√2 = 4.828+
(David W. Cantrell)

2-Sliced Squares in Squares
1

s = 1 		2

s = √2 = 1.414+ 		3

s = (1+√2)/2 = 1.707+ 		4

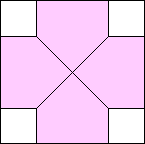
s = 2
5

s = 3√2/2 = 2.121+
  		6

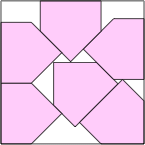
s = 2 + √2/4 = 2.353+
(David W. Cantrell) 		7

s = 1+11√2/10 = 2.555+
(Maurizio Morandi) 		8

s = 2 + 1/√2 = 2.707+
(Maurizio Morandi)
9

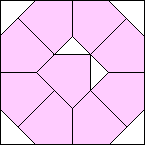
s = 2√2 = 2.828+
(Maurizio Morandi) 		10

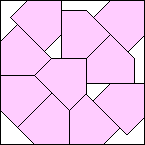
s =(2+7√2)/4= 2.974+
(Maurizio Morandi) 		11

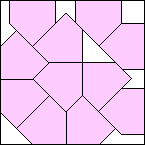
s = 1 + 3/√2 = 3.121+
(Maurizio Morandi) 		12

s =(6+5√2)/4= 3.267+
(Maurizio Morandi)
13

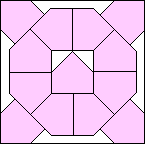
s = 2 + √2 = 3.414+
(Maurizio Morandi) 		14

s = 5/√2 = 3.535+
(Maurizio Morandi) 		15

s =1+15√2/8= 3.651+
(Maurizio Morandi) 		16

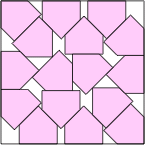
s=(15+16√2)/10=3.762+
(David W. Cantrell)

2-Sliced Squares in 2-Sliced Squares
1

s = 1
  		2

s = 1.873+
(Maurizio Morandi) 		3

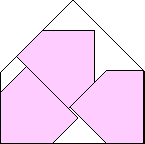
s = 5/4 + 1/√2 = 1.957+
(Maurizio Morandi) 		4

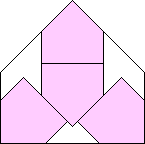
s = 2(2+√2)/3 = 2.276+
 
5

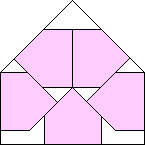
s = 5/2 = 2.5
(Maurizio Morandi) 		6

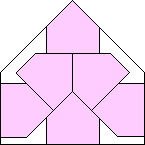
s = 2 + 1/√2 = 2.707+
(Maurizio Morandi) 		7

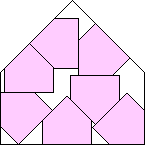
s=(32+19√2)/20=2.943+
(Maurizio Morandi) 		8

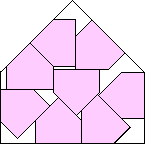
s=(25+13√2)/14=3.098+
(Maurizio Morandi)
9

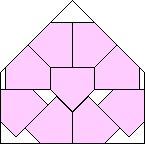
s = 4(1+√2)/3 = 3.218+
(Maurizio Morandi) 		10

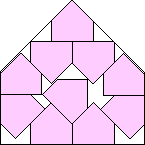
s =(11+2√2)/4= 3.457+
(Maurizio Morandi) 		11

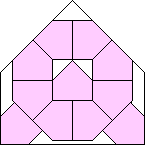
s= 2(4+√2)/3= 3.609+
(Maurizio Morandi) 		12

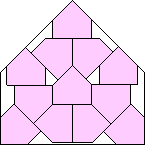
s = 7/3 + √2 = 3.747+
(Maurizio Morandi)
13

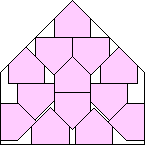
s = 47/12 = 3.916+
(Maurizio Morandi) 		14

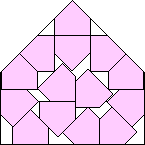
s=(30+19√2)/14=4.062+
(Maurizio Morandi) 		15

s =(7+4√2)/3= 4.218+
(Maurizio Morandi) 		16

s =(16+7√2)/6= 4.316+
(Maurizio Morandi)

Squares in 2-Sliced Squares
1

s = √2 = 1.414+
(Andrew Bayly) 		2

s = 2
  		3

s = 2(2+√2)/3 = 2.276+
  		4

s = 2√2 = 2.828+
 
5

s = 3
  		6

s = 1 + 3/√2 = 3.121+
(Maurizio Morandi) 		7

s = 7/2 = 3.5
  		8

s = 1 + 2√2 = 3.828+
(David W. Cantrell)
9

s = 5/2 + √2 = 3.914+ 		10

s = 4 		11

s = 2 + 3/√2 = 4.121+ 		12

s = 9/2 = 4.5
13

s = 10√2/3 = 4.714+ 		14

s = 2 + 2√2 = 4.828+ 		15

s = 2 + 2√2 = 4.828+ 		16

s = 5

3-Sliced Squares in Squares
1

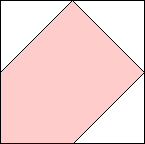
s = 1 		2

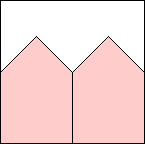
s = √2 = 1.414+ 		3

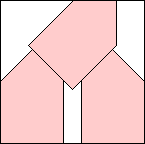
s = 2(1+√2)/3 = 1.609+ 		4

s = 5√2/4 = 1.767+
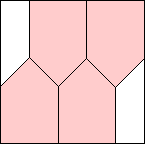
5

s = √2+√10/5 = 2.046+
(Maurizio Morandi) 		6

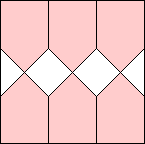
s = 3/√2 = 2.121+
  		7

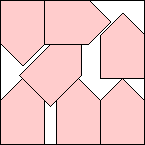
s =(4+7√2)/6= 2.316+
(Maurizio Morandi) 		8

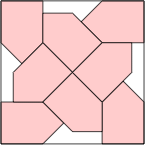
s = 1 + √2 = 2.414+
(David W. Cantrell)
9

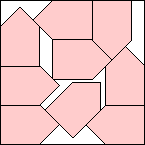
s = 11√2/6 = 2.592+
(Maurizio Morandi) 		10

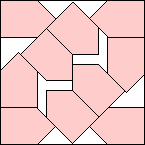
s =1+5√2/4= 2.767+
(Maurizio Morandi) 		11

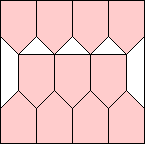
s = 2√2 = 2.828+
  		12

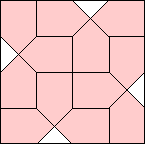
s = 2√2 = 2.828+
(Maurizio Morandi)
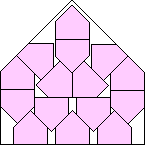
13

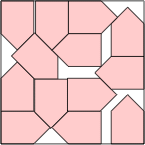
s=(6+35√2)/18=3.083+
(David W. Cantrell) 		14

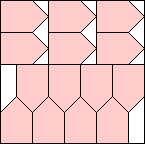
s = 9√2/4 = 3.181+
  		15

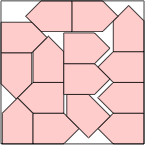
s=(4+53√2)/24=3.289+
(David W. Cantrell) 		16

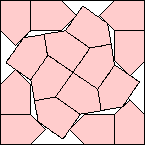
s = 3.384+
(Maurizio Morandi)

3-Sliced Squares in 3-Sliced Squares
1

s = 1
  		2

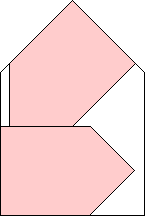
s = (2+2√2)/3 = 1.609+
  		3

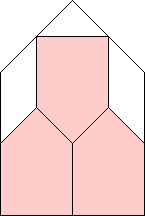
s = 2
  		4

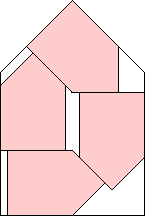
s = (3+√2)/2 = 2.207+
(Maurizio Morandi)
5

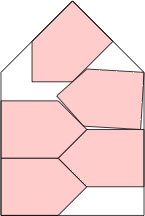
s = 2.499+
(David W. Cantrell) 		6

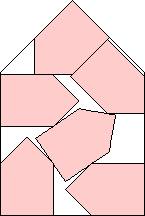
s = 2.742+
(Maurizio Morandi) 		7

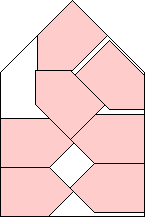
s = (9+4√2)/5 = 2.931+
(Maurizio Morandi) 		8

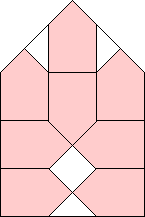
s = 3
(Maurizio Morandi)
9

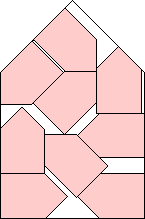
s = 11/6 + √2 = 3.247+
(Maurizio Morandi) 		10

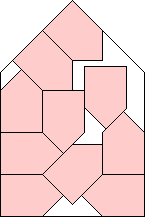
s = 2 + √2 = 3.414+
(Maurizio Morandi) 		11

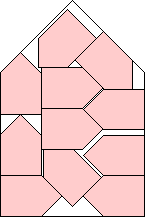
s =4(3+√2)/5= 3.531+
(Maurizio Morandi) 		12

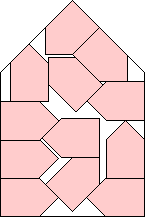
s=(29+6√2)/10=3.748+
(Maurizio Morandi)
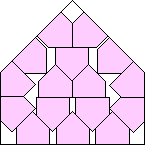
13

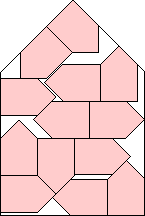
s=(23+3√2)/7=3.891+
(Maurizio Morandi) 		14

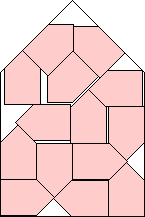
s=(33+2√2)/9=3.980+
(Maurizio Morandi) 		15

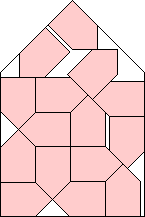
s = 3+4√2/5 = 4.131+
(Maurizio Morandi) 		16

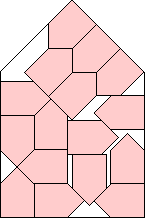
s =2(5+√2)/3= 4.276+
(Maurizio Morandi)

Squares in 3-Sliced Squares
1

s = √2 = 1.414+ 		2

s = 2(2+√2)/3 = 2.276+ 		3

s = 2√2 = 2.828+ 		4

s = 2√2 = 2.828+
5

s = 1 + 3/√2 = 3.121+
  		6

s = 3.665+
(David W. Cantrell) 		7

s = 3.912+
(David W. Cantrell) 		8

s = 2 + 3/√2 = 4.121+
 
9

s = 3√2 = 4.242+ 		10

s = 3√2 = 4.242+ 		11

s = 10√2/3 = 4.714+ 		12

s = 2 + 2√2 = 4.828+
13

s = 11√2/3 = 5.185+
(Maurizio Morandi) 		14

s = 5.388+
(Maurizio Morandi) 		15

s = 4/3+3√2 = 5.575+
(David W. Cantrell) 		16

s = 4√2 = 5.656+
 

If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 8/15/12.