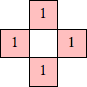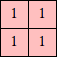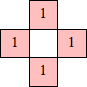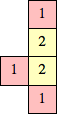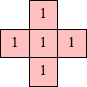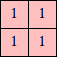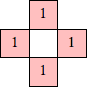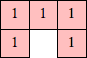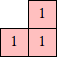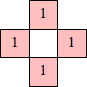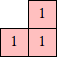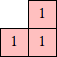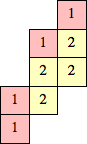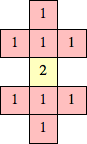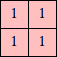Problem of the Month (July 2017)
Let S be a subset of the 8 vectors to the nearest neighbors on a square lattice. Let p be a fraction between 0 and 1. We are interested in finding a set of squares and positive integer values on those squares so that each square is p times the sum of the squares pointed to by S. What are the smallest solutions (in terms of the total of the squares) for various sets S? What about other fractions besides p=1⁄2? What if other vectors besides nearest neighbors are allowed? What if vectors are allowed to be repeated?
ANSWERS
Here are the best known solutions.
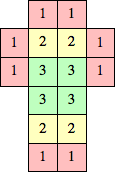
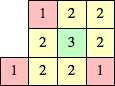
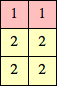
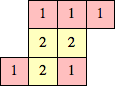
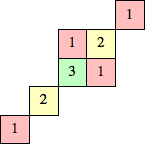
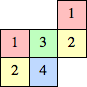
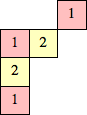
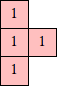
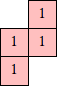
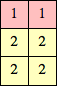
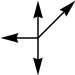
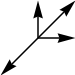
3 Directions, p=1⁄2
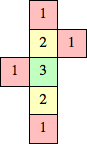
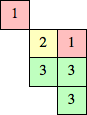
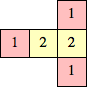
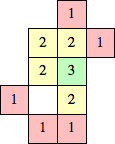
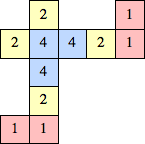
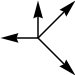
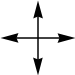
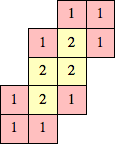
4 Directions, p=1⁄2
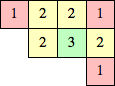
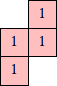
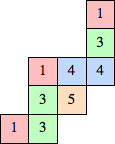
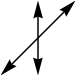
5 Directions, p=1⁄2
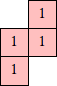
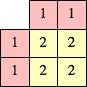
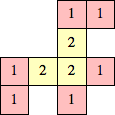
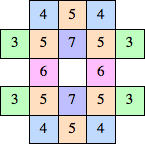
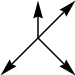
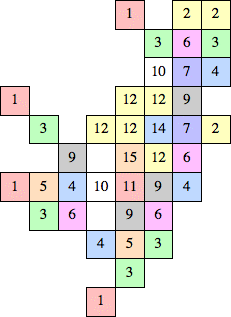
6+ Directions, p=1⁄2
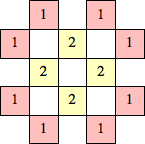
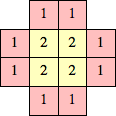
4 Directions, p=1⁄3
| 
| 
| 
| 
| 
| 
| 
|
| ?
| ?
| ?
| 
| 
(Johannes Waldmann)
| ?
| 
| 
|
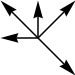
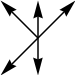
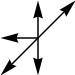
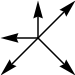
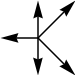
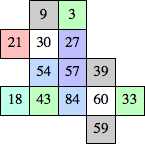
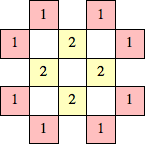
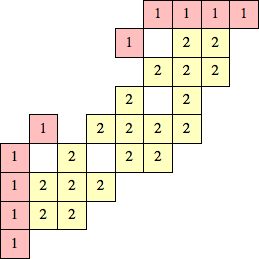
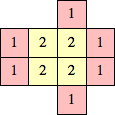
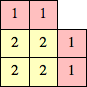
5 Directions, p=1⁄3
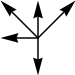
| 
| 
| 
| 
| 
| 
| 
|
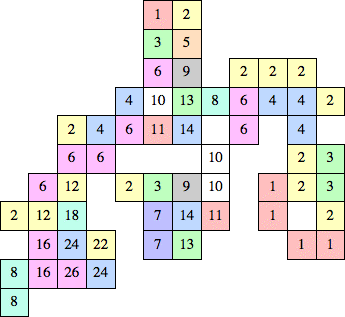
(Johannes Waldmann)
| 
| 
| 
| 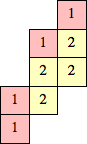
| 
(Johannes Waldmann)
| 
| 
|
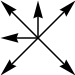
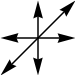
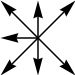
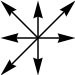
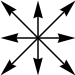
6+ Directions, p=1⁄3
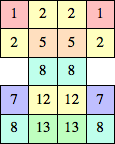
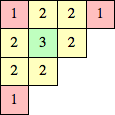
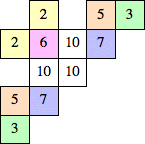
5 Directions, p=1⁄4
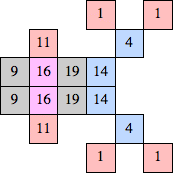
6+ Directions, p=1⁄4
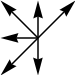
| 
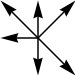
| 
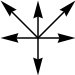
| 
| 
| 
| 
| 
| 
|
| ?
| ?
| ?
| 
| 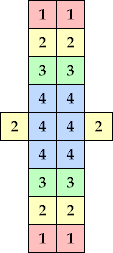
(George Sicherman)
| 
| 
| 
| 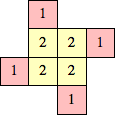
(George Sicherman)
|
6+ Directions, p=1⁄5
If you can extend any of these results, please
e-mail me.
Click here to go back to Math Magic. Last updated 7/25/17.