Problem of the Month (August 2015)
This month we consider packing polylines, collections of infinitesimally thin line segments of length 1 connected at right angles. Some of these present no problem at all, we can pack infinitely many of them in a finite region without crossing by "nesting" them. Others require more space. What are the best packings of these small polylines for various values of n?
ANSWERS
Solutions were received from Maurizio Morandi, Joe DeVincentis, George Sicherman, and Bernd van der Zwaag.
George Sicherman pointed out there was a tetraline missing from my catalogue.
Maurizio Morandi pointed out that 3 U's can be nested in a square arbitrarily close to side 1. Therefore, in many cases, the best known packings of this polyline reduce to the packing of squares.
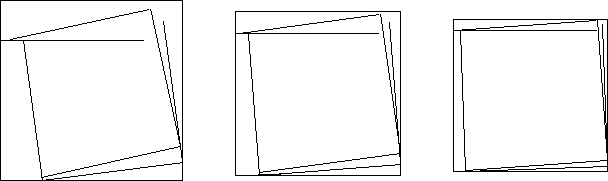
He also found limiting solutions for a few tetralines in a 2×2 square. Joe DeVincentis then used a similar technique to improve the best known solutions for 3×3 squares.
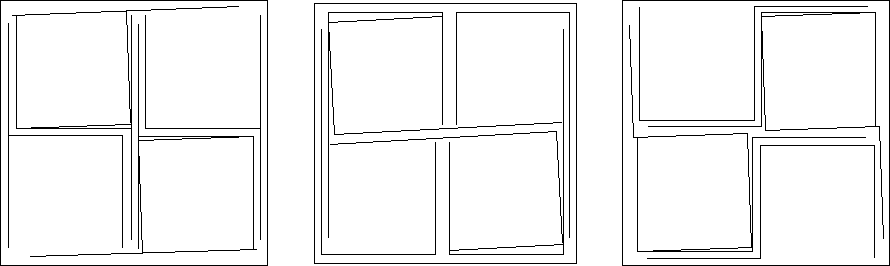
Then Berend van der Zwaag found limiting solutions for C's:
Here are the smallest known solutions:

| n=3

L = 1
(MM)
| n=4

L = 1 + √2/2
| n=12

L = 2
| n=15
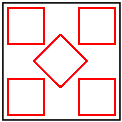
L = 2 + √2/2
| n=16
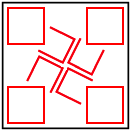
L = 2 + 2√5/5
(JD)
| n=27

L = 3
|
|

| n=2

L = √2
| n=3
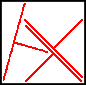
L = (√6+√2)/2
(MM)
| n=4
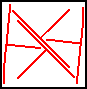
L = 1.989+
(MM)
| n=6
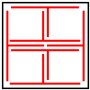
L = 2
| n=8
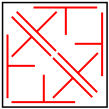
L = 1 + √2
| n=10
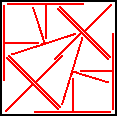
L = 2.663+
(MM)
| n=14
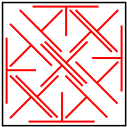
L = 2√2
| n=16
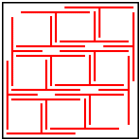
L = 3
|
|

| n=1

L = √2
| n=2

L = 3√2/2
| n=3

L = 7√2/4
| n=5

L = 2√2
| n=6

L = 9√2/4
|
n=8

L = 5√2/2
| n=9
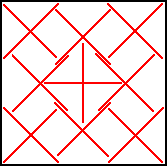
L = 1 + 2√2
(MM)
| n=13

L = 3√2
(JD)
|
|

| n=2

L = 2
| n=4

L = √5
| n=8
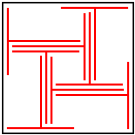
L = 3
| n=10
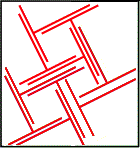
L = 8√5/5
(JD)
| n=11
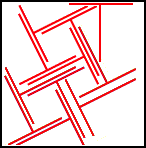
L = 3.647+
(JD)
| n=12
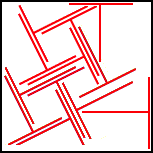
L = 2+4√5/5
(JD)
| n=18
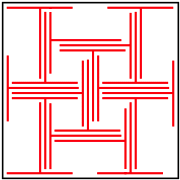
L = 4
(JD)
|
|

| n=2

L = 2
| n=4

L = √5
| n=5
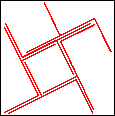
L = 6√5/5
(MM)
| n=8

L = 2√2
(MM)
|
|

| n=1

L = 4√5/5
| n=6

L = 2
(MM)
| n=8
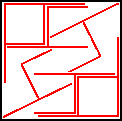
L = 1 + 4√5/5
(MM)
| n=16
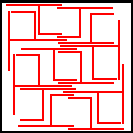
L = 3
(JD)
|
|

| n=1

L = 4√5/5
| n=2

L = √(2+√2)
(MM)
| n=6

L = 2
(MM)
| n=10

L = 2√2
(MM)
| n=18

L = 3
(JD)
|
|

| n=1

L = 4√5/5
| n=2

L = 1.983+
(MM)
| n=6

L = 2
(MM)
| n=10

L = 2√2
(MM)
| n=16
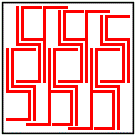
L = 3
(JD)
|
|

| n=1

L = 4√5/5
| n=2

L = √(2+√2)
(MM)
| n=6

L = 2
| n=8
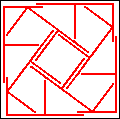
L = 2.768+
(MM)
| n=16
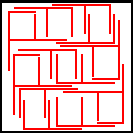
L = 3
(JD)
|
|

| n=6

L = 2
(BZ)
| n=7
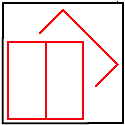
L = 2 + √2/2
(BZ)
| n=8
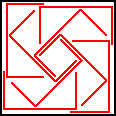
L = 2.748+
(MM)
| n=12

L = 3
(BZ)
|
|

| n=2

L = 3√2/2
| n=3

L = 3√((5+√5)/10)
(MM)
| n=4

L = √(15+√153)/2
(GS)
| n=6
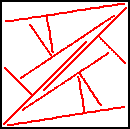
L = 2.962+
(MM)
| n=8
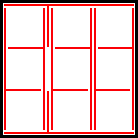
L = 3
|
|
If you can extend any of these results, please
e-mail me.
Click here to go back to Math Magic. Last updated 8/11/15.