Problem of the Month (August 2017)

For a set S of U.S. states, we say its adjacency set A(S) is the set of numbers that count how many states in S are adjacent to each state in S. (You can find the neighboring states here except we do not consider the point contacts of Colorado-Arizona, New Mexico-Utah, or the water connections of Michigan-Minnesota, Michigan-Illinois, or New York-Rhode Island neighbors.) If T is a non-empty subset of {1,2,3,4,5,6,7,8}, does there exist a collection of states S with A(S)=T? If so, what is the largest such set? Which largest sets are unique?

ANSWERS

Here are the best known solutions.

One Digit

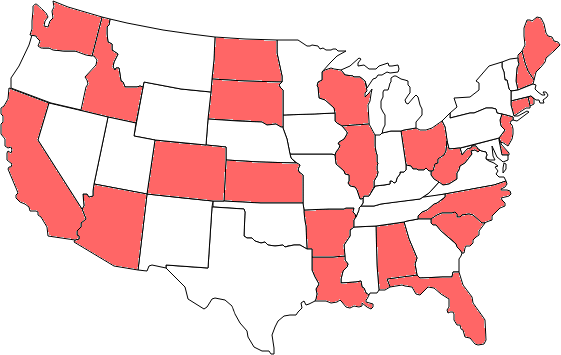
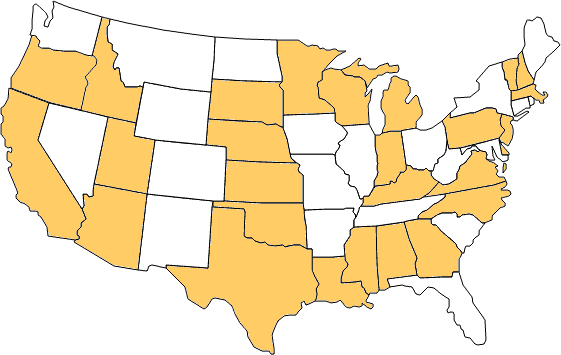
{1}: 24 states	{2}: 27 states (George Sicherman)
{3}: none	{4}: none
{5}: none	{6}: none
{7}: none	{8}: none

Two Digits

{1,2}: 31 states (George Sicherman)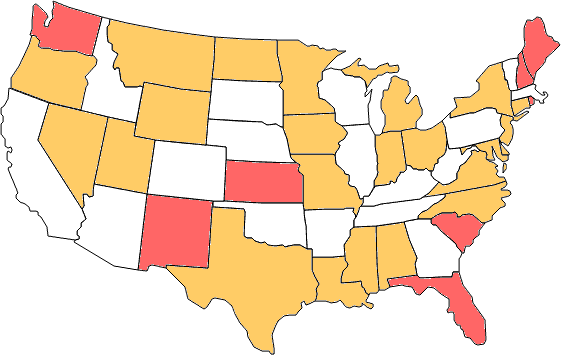	{1,3}: 30 states (George Sicherman)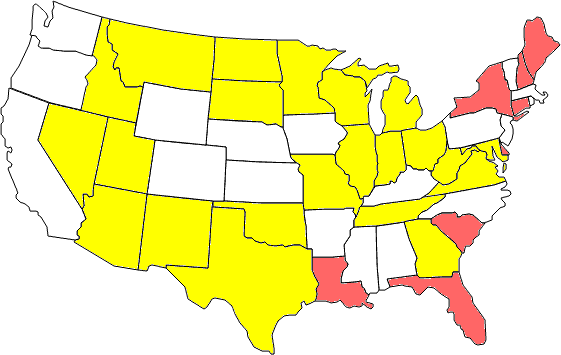
{1,4}: 23 states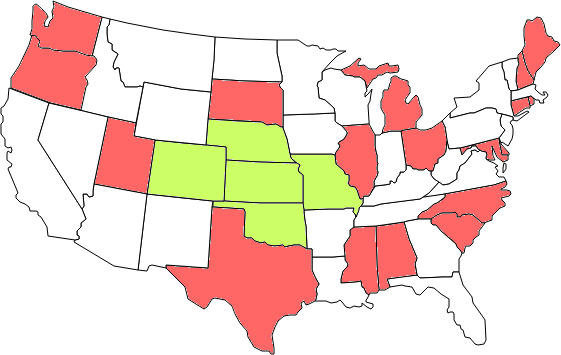	{1,5}: none (Bryce Herdt)
{1,6}: none	{1,7}: none
{1,8}: none	{2,3}: 34 states (Bryce Herdt)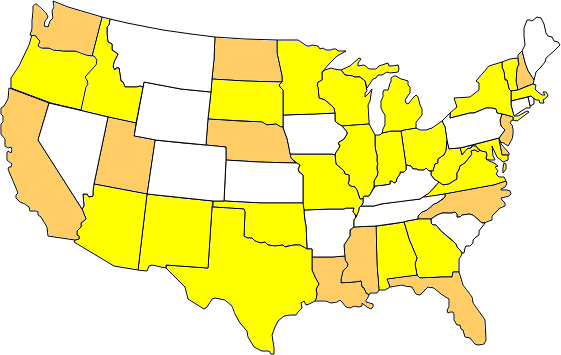
{2,4}: 32 states (Rob Pratt)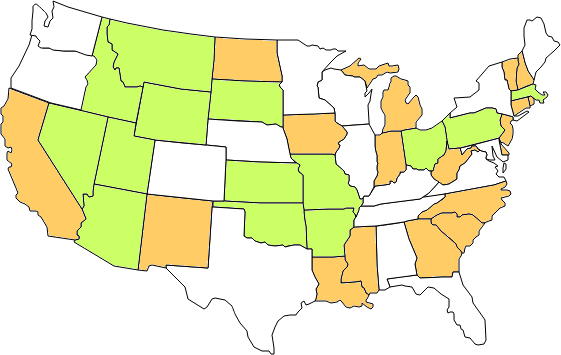	{2,5}: 23 states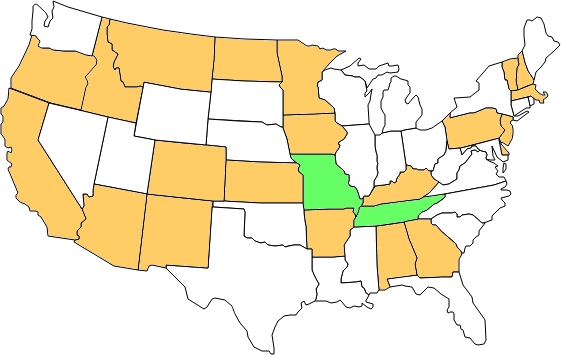
{2,6}: none	{2,7}: none
{2,8}: none	{3,4}: 25 states (George Sicherman)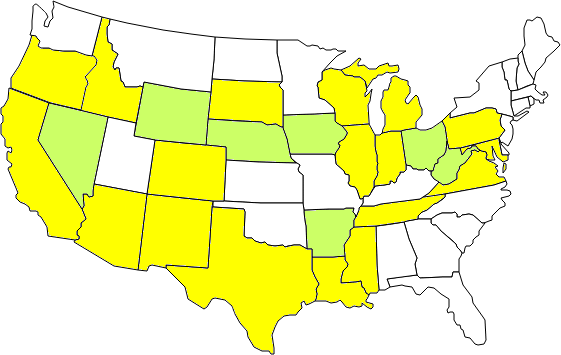
{3,5}: 20 states (George Sicherman)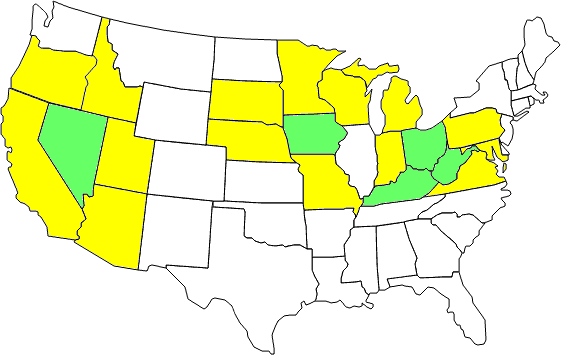	{3,6}: 13 states (George Sicherman)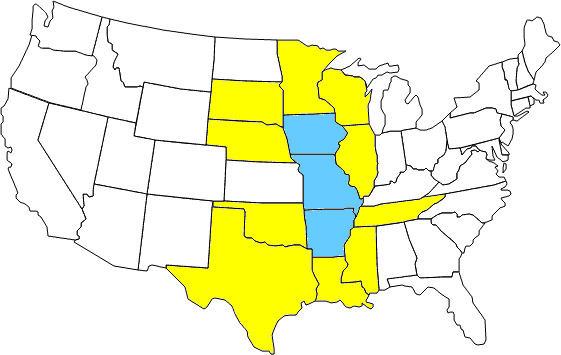
{3,7}: 8 states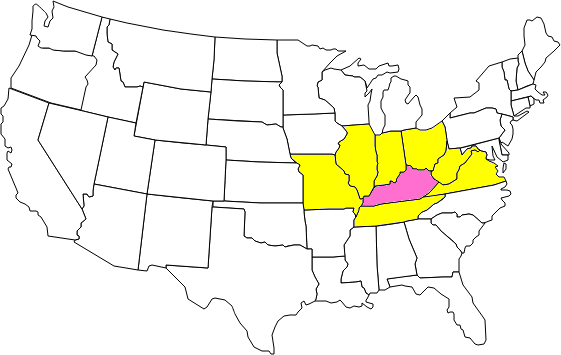	{3,8}: 9 states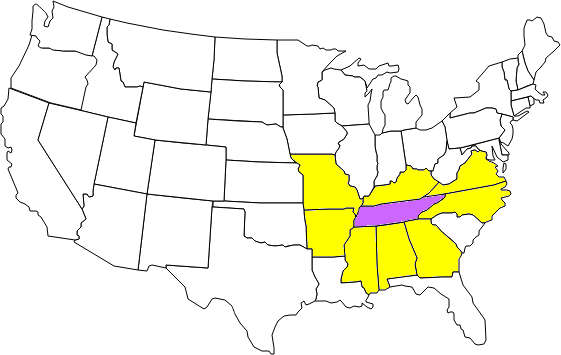
{4,5}: none (Bryce Herdt)	{4,6}: none (Bryce Herdt)
{4,7}: none (Bryce Herdt)	{4,8}: none (Bryce Herdt)
{5,6}: none (Bryce Herdt)	{5,7}: none (Bryce Herdt)
{5,8}: none	{6,7}: none
{6,8}: none	{7,8}: none

Three Digits

{1,2,3}: 36 states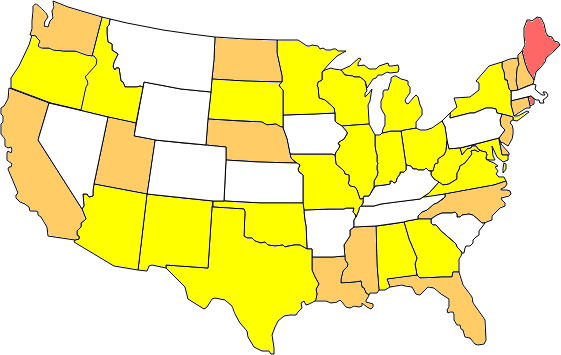	{1,2,4}: 35 states (Rob Pratt)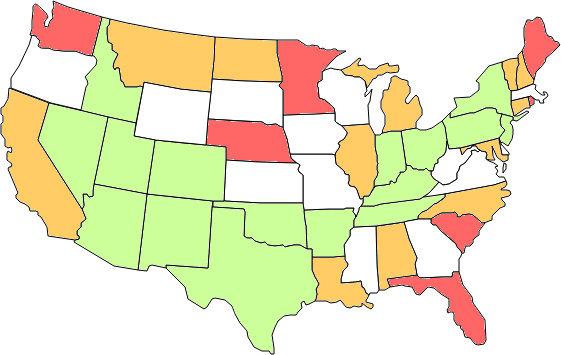
{1,2,5}: 29 states (Bryce Herdt)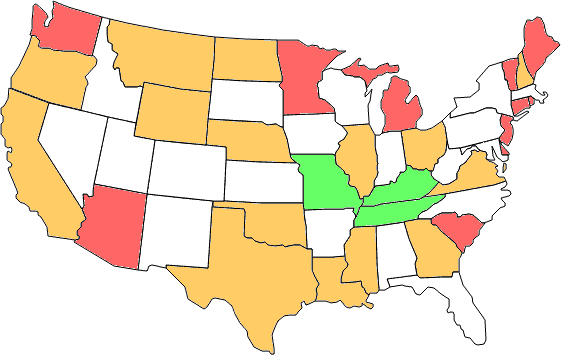	{1,2,6}: none
{1,2,7}: none	{1,2,8}: none
{1,3,4}: 36 states (Bryce Herdt)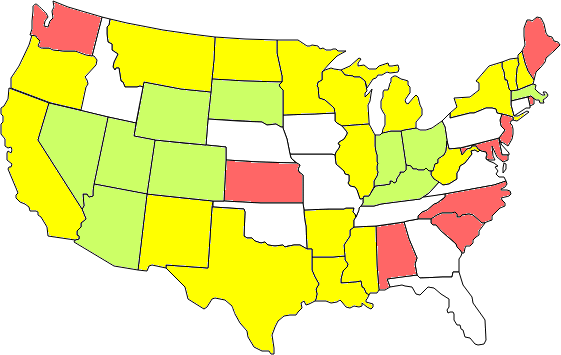	{1,3,5}: 34 states (Rob Pratt)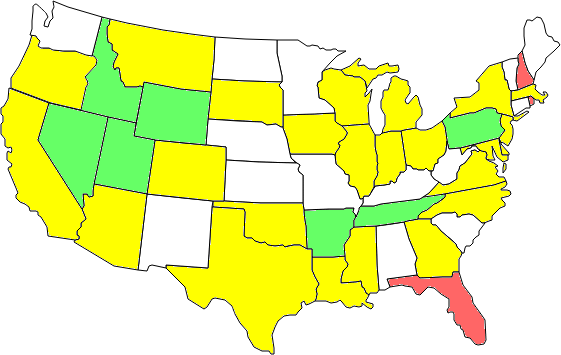
{1,3,6}: 31 states (Bryce Herdt)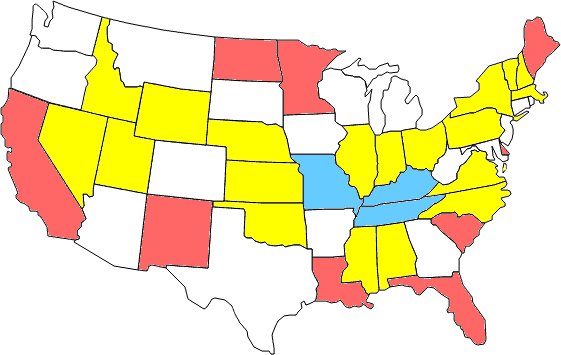	{1,3,7}: 28 states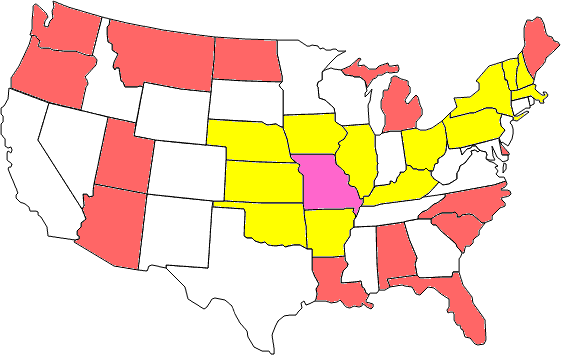
{1,3,8}: 25 states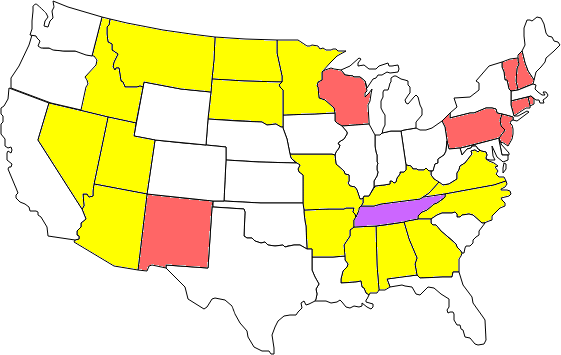	{1,4,5}: 23 states (Rob Pratt)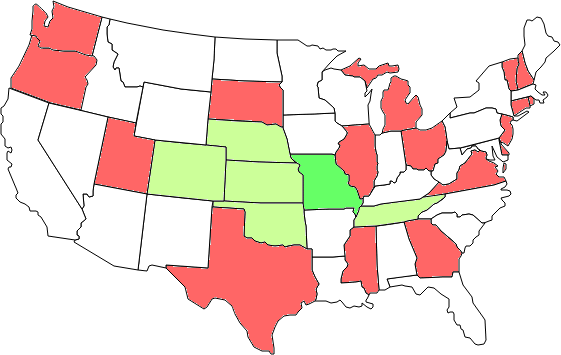
{1,4,6}: 26 states (George Sicherman)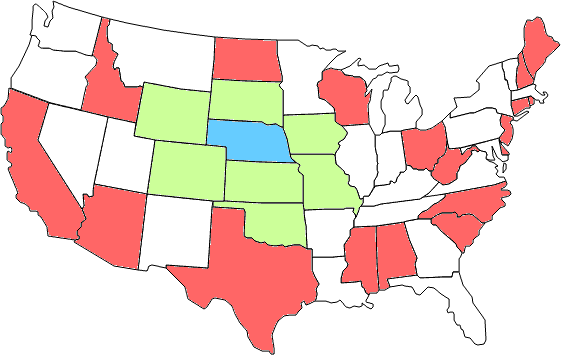	{1,4,7}: none (Rob Pratt)
{1,4,8}: none	{1,5,6}: none (Rob Pratt)
{1,5,7}: none (Rob Pratt)	{1,5,8}: none
{1,6,7}: none (Rob Pratt)	{1,6,8}: none
{1,7,8}: none (George Sicherman)	{2,3,4}: 39 states (Bryce Herdt)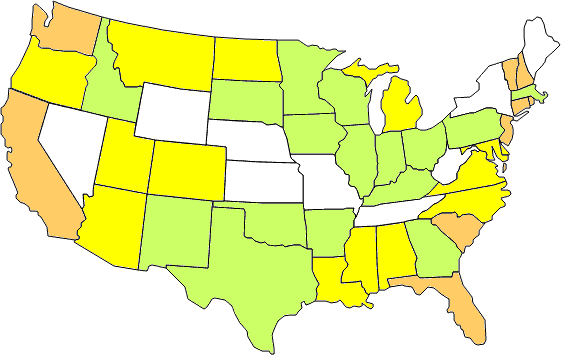
{2,3,5}: 37 states (Rob Pratt)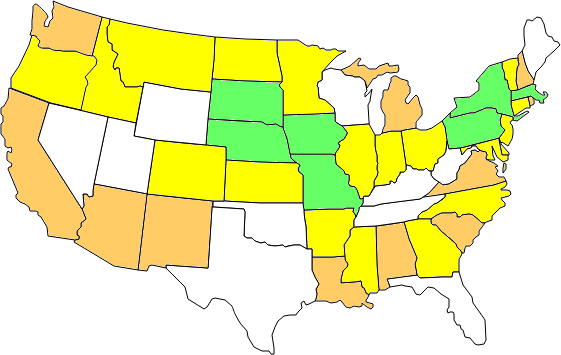	{2,3,6}: 33 states (Rob Pratt)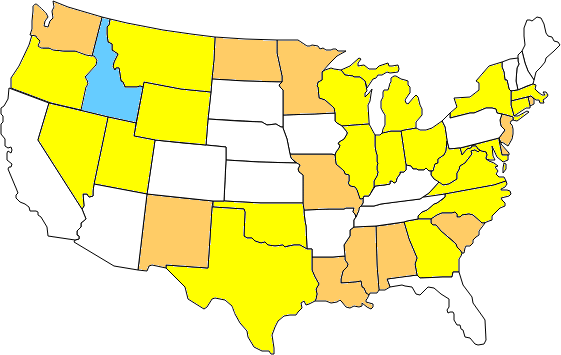
{2,3,7}: 31 states (Bryce Herdt)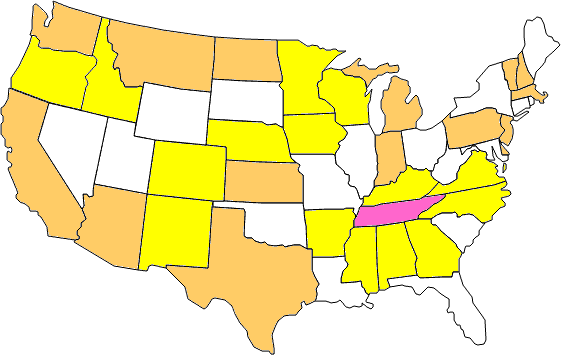	{2,3,8}: 26 states (Bryce Herdt)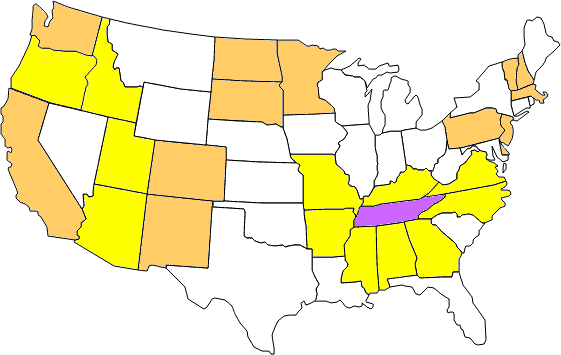
{2,4,5}: 36 states (Bryce Herdt)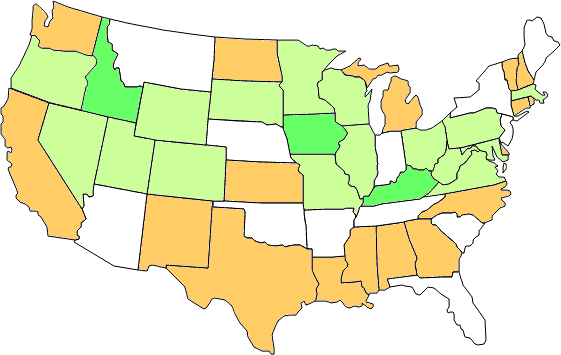	{2,4,6}: 35 states (George Sicherman)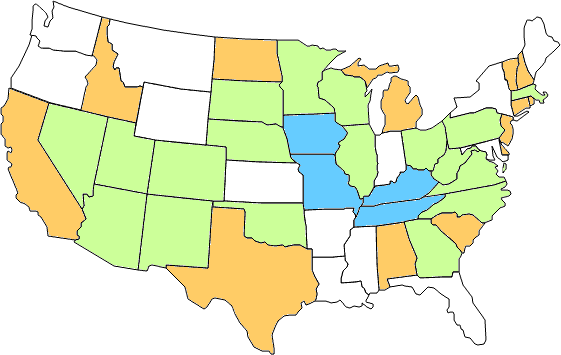
{2,4,7}: 31 states (Rob Pratt)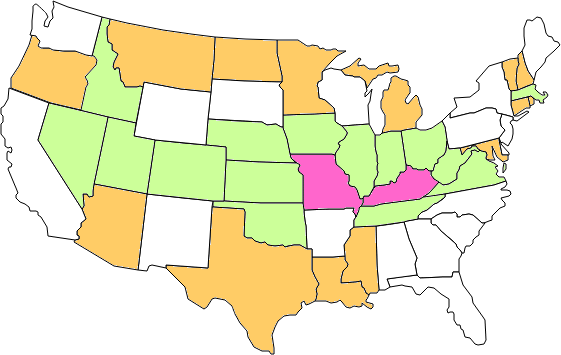	{2,4,8}: 28 states (George Sicherman)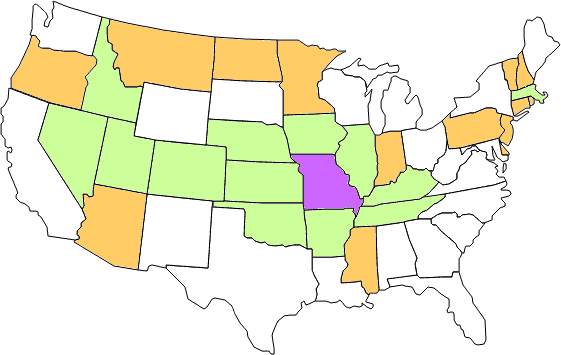
{2,5,6}: 23 states (Bryce Herdt)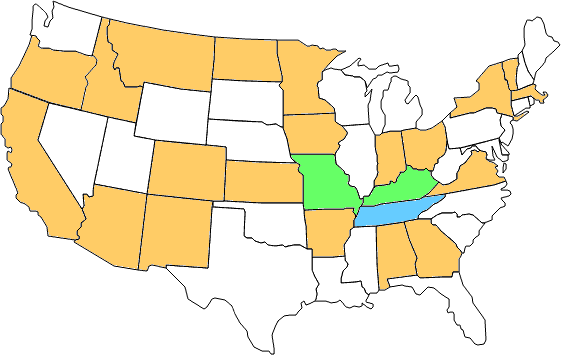	{2,5,7}: none (Rob Pratt)
{2,5,8}: none	{2,6,7}: none (Rob Pratt)
{2,6,8}: none	{2,7,8}: none (George Sicherman)
{3,4,5}: 30 states (Bryce Herdt)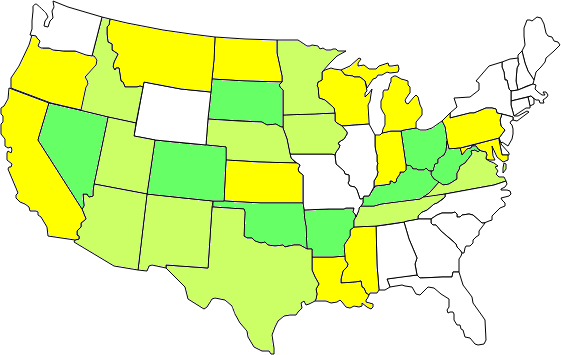	{3,4,6}: 29 states (George Sicherman)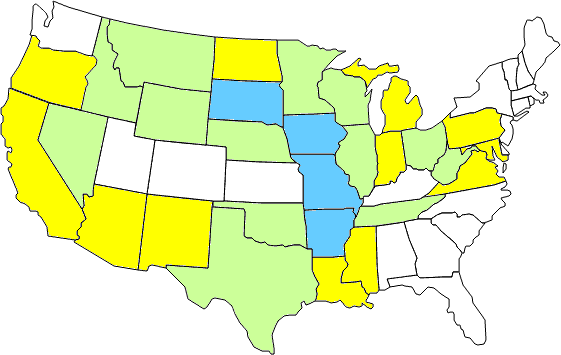
{3,4,7}: 29 states (Rob Pratt)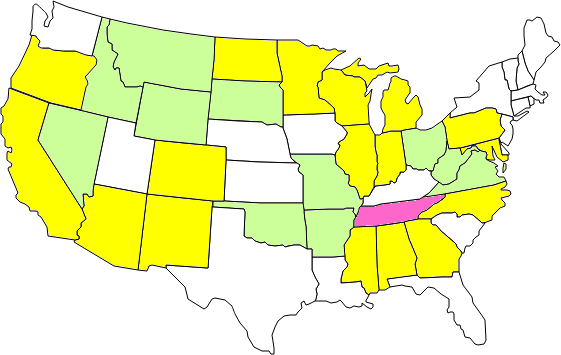	{3,4,8}: 15 states (George Sicherman)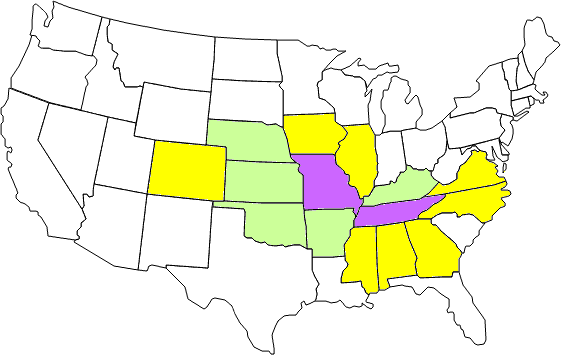
{3,5,6}: 23 states (Rob Pratt)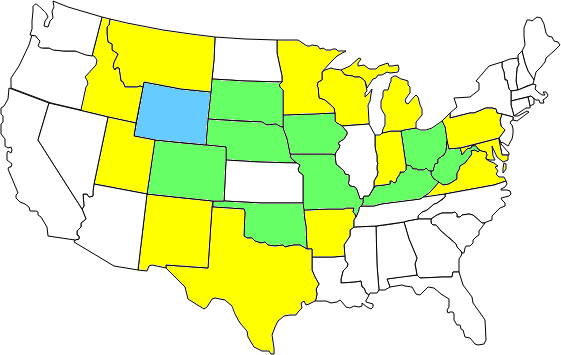	{3,5,7}: 14 states (George Sicherman)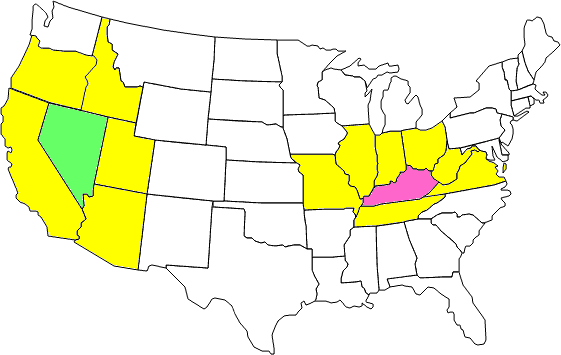
{3,5,8}: 23 states (George Sicherman)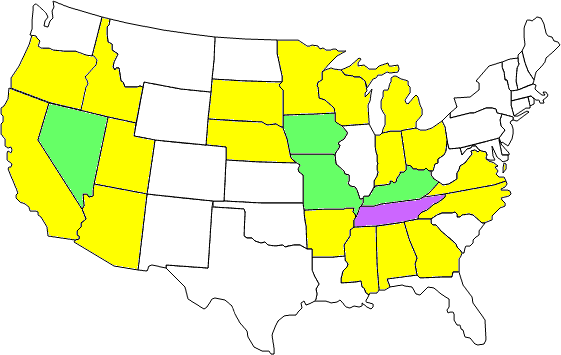	{3,6,7}: none (Rob Pratt)
{3,6,8}: 15 states (George Sicherman)	{3,7,8}: none (Bryce Herdt)
{4,5,6}: none (Bryce Herdt)	{4,5,7}: none (Bryce Herdt)
{4,5,8}: none (Bryce Herdt)	{4,6,7}: none (Bryce Herdt)
{4,6,8}: none (Bryce Herdt)	{4,7,8}: none (Bryce Herdt)
{5,6,7}: none (Bryce Herdt)	{5,6,8}: none (Bryce Herdt)
{5,7,8}: none (Bryce Herdt)	{6,7,8}: none (Bryce Herdt)