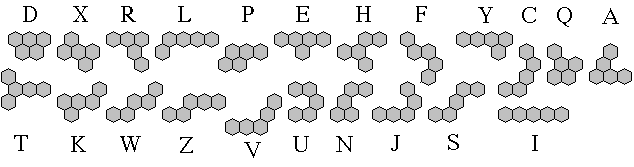
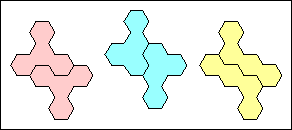
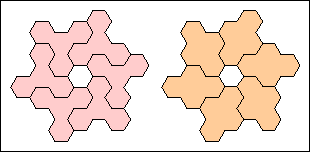
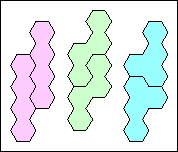
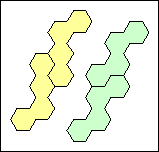
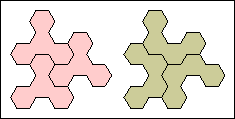
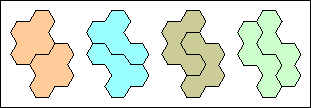
The problem for the 3 trihexes is trivial, since a pair of any of them will tile the same region. The problem for the 7 tetrahexes is more interesting, but still pretty easy. What are the results for the 22 pentahexes below?

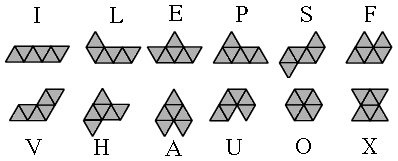
The problems for diaboloes and triaboloes are pretty easy. What are the best results for the 14 tetraboloes below?
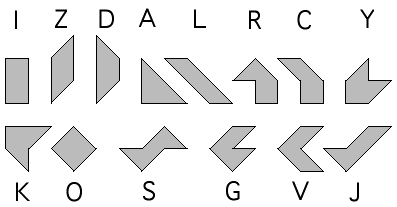
The problem for the generalized triominoes is trivial. What are the results for the 22 generalized tetrominoes?
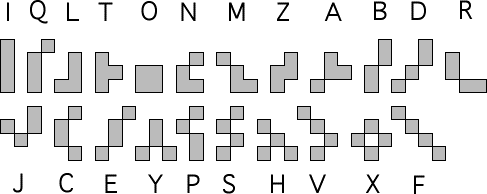
Joe DeVincentis sent a complete solution to the tetrahex problem, and found many of the best known solutions for the pentahex problem. Jeremy Galvagni sent some pentahex solutions. George Sicherman found better solutions for several pairs of pentahexes.
|  |
 |  |  |  |  | 
| |
 | 2 | 2 | 2 | 2 | 3 | ∞ |
 | 2 | 2 | 2 | 2 | 6 | |
 | 2 | 2 | 2 | 2 | ||
 | 2 | 2 | 2 | |||
 | 2 | 2 | ||||
 | 3 |
 |  |  |  |
 |  |  |
 |  |  |
| X | R | L | P | E | H | F | Y | C | Q | A | T | K | W | Z | V | U | N | J | S | I | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| D | 3 | 2 | 2 | 2 | 3 | 3 | 3 | 2 | 3 | 2 | 2 | 6 | 2 | 2 | 2 | 3 | 2 | 2 | 2 | 2 | 2 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| X | 2 | 8 | 2 | 2 | 3 | 6 | 2 | 30 | 2 | 3 | 60 | 2 | 6 | 4 | 24 | 78 | 3 | 3 | 15 | 35
| R | 2 | 2 | 2 | 3 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 2 | 3 | 2 | 2 | 2 | 3
| L | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 3 | 2 | 2 | 2 | 2 | 2 | 2
| P | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 6 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 3 | 2
| E | 2 | 3 | 2 | 3 | 2 | 2 | 3 | 2 | 2 | 2 | 18 | 3 | 2 | 4 | 2 | 2
| H | 2 | 6 | 3 | 2 | 2 | 2 | 2 | 2 | 2 | 11 | 3 | 2 | 2 | 2 | 10
| F | 6 | 2 | 3 | 2 | 2 | 2 | 2 | 2 | 6 | 2 | 2 | 2 | 2 | 9
| Y | 3 | 2 | 2 | 2 | 2 | 6 | 2 | 2 | 6 | 2 | 2 | 2 | 2
| C | 2 | 4 | 2 | 2 | 3 | 2 | 2 | 3 | 2 | 2 | 2 | 9
| Q | 3 | 2 | 2 | 2 | 2 | 4 | 6 | 3 | 2 | 2 | 8
| A | 6 | 2 | 8 | 3 | 2 | 6 | 2 | 2 | 6 | 3
| T | 2 | 6 | 2 | 2 | 3 | 3 | 3 | 2 | ∞
| K | 2 | 2 | 3 | 3 | 2 | 2 | 2 | 8
| W | 2 | 10 | 2 | 2 | 3 | 4 | 5
| Z | 2 | 2 | 2 | 2 | 2 | 2
| V | 6 | 2 | 2 | 3 | 3
| U | 2 | 2 | 2 | 18
| N | 2 | 3 | 3
| J | 2 | 3
| S | 5
| |
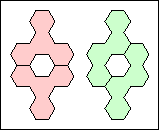
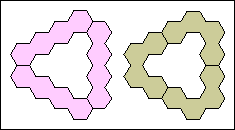
The pictures of the largest compatibility regions for pentahexes are here, and hexahexes are here: 1, 2, 3, 4, 5, 6, 7, 8, 9, and 10, courtesy of George Sicherman.
Margarita Lukjanska sent me her results on hexiamonds, many of which improved on my results. She and Mike Reid both gave me the reference of "Puzzle Fun" published in August 1995 by R. M. Kurchan from Buenos Aires, which published Mike's results on the problem.
| L | E | P | S | F | V | H | A | U | O | X | |
| I | 3 | 3 | 2 | 3 | 2 | 2 | 3 | 2 | 3 | ∞ | ∞ |
| L | 3 | 2 | 3 | 2 | 3 | 2 | 3 | 2 | 6 | 3 | |
| E | 6 | 4 | 6 | 6 | 2 | 3 | 3 | ∞ | 3 | ||
| P | 2 | 2 | 2 | 2 | 2 | 2 | 6 | 2 | |||
| S | 2 | 3 | 2 | 18 | 2 | 3 | ∞ | ||||
| F | 2 | 2 | 2 | 2 | 6 | 6 | |||||
| V | 3 | 2 | 3 | 3 | ∞ | ||||||
| H | 3 | 2 | 6 | 3 | |||||||
| A | 3 | ∞ | ∞ | ||||||||
| U | 3 | ∞ | |||||||||
| O | ∞ |
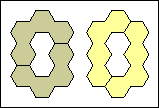
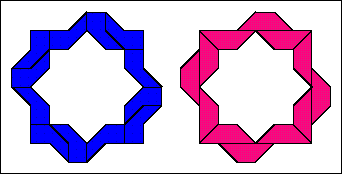
Here is a picture of the largest case:
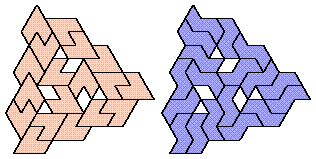
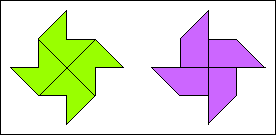
Scott Reynolds sent me these results on hexiamonds pairings that are infinite.
|
|
 |  |  |
 |  |  |
| Z | D | A | L | R | C | Y | K | O | S | G | V | J | |
| I | ∞ | 4 | 2 | ∞ | 4 | ∞ | ∞ | 4 | ∞ | ∞ | ∞ | ∞ | ∞ |
| Z | 2 | 2 | 2 | ∞ | ∞ | ∞ | 4 | ∞ | ∞ | ∞ | 2 | ∞ | |
| D | 4 | 4 | 2 | 12 | ∞ | 2 | ∞ | ∞ | 2 | 4 | 2 | ||
| A | 2 | 4 | 6 | ∞ | 2 | 4 | 4 | 4 | ∞ | 4 | |||
| L | 4 | ∞ | 2 | 4 | ∞ | ∞ | 2 | ∞ | 2 | ||||
| R | 2 | 4 | 4 | 4 | 4 | 2 | ∞ | 4 | |||||
| C | ∞ | 2 | ∞ | ∞ | 4 | ∞ | 4 | ||||||
| Y | 2 | ∞ | ∞ | ∞ | 4 | ∞ | |||||||
| K | ∞ | 4 | 4 | 16 | 2 | ||||||||
| O | ∞ | 2 | ∞ | 2 | |||||||||
| S | 2 | ∞ | 2 | ||||||||||
| G | 2 | 2 | |||||||||||
| V | 2 |
 |  |  |  |
 |  |  |  |
 |  |  |  |  |
 |  |  |  |
 |  |  |  |  |
 |  |  |  |  |
 |  |  |
 |  |  |
The 5-aboloes are here, here, and here.
 |  |  | 
| |
 | 2 | 2 | 2 | 3 |
 | 2 | 2 | 2 | |
 | 2 | 2 | ||
 | 2 |
 |  |
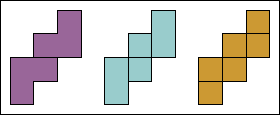 |  |
| Q | L | T | O | N | M | Z | A | B | D | R | J | C | E | Y | P | S | H | V | X | F | |
| I | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 12 | 2 | 4 | 4 | 4 | 2 | 4 | 4 | 2 | 2 | 4 | 4 | ∞ | 4 |
| Q | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 4 | 4 | |
| L | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | 8 | 4 | ||
| T | 4 | 2 | 2 | 2 | 2 | 4 | 2 | 4 | 2 | 4 | 4 | 4 | 2 | 2 | 2 | 2 | 2 | 4 | |||
| O | 2 | 2 | 4 | 4 | 2 | 4 | 4 | 4 | 2 | 4 | 4 | 2 | 2 | 4 | 2 | ∞ | 4 | ||||
| N | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | |||||
| M | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 2 | 4 | 2 | ||||||
| Z | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 2 | 2 | |||||||
| A | 2 | 2 | 4 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 2 | 4 | ||||||||
| B | 2 | 2 | 2 | 2 | 4 | 2 | 2 | 2 | 4 | 2 | 8 | 4 | |||||||||
| D | 2 | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 2 | 4 | 2 | ||||||||||
| R | 2 | 2 | 2 | 2 | 2 | 4 | 2 | 4 | 8 | 4 | |||||||||||
| J | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 4 | ||||||||||||
| C | 2 | 4 | 2 | 2 | 4 | 2 | 4 | 2 | |||||||||||||
| E | 2 | 2 | 2 | 4 | 2 | 2 | 2 | ||||||||||||||
| Y | 2 | 2 | 2 | 2 | 2 | 4 | |||||||||||||||
| P | 2 | 2 | 2 | 2 | 4 | ||||||||||||||||
| S | 2 | 2 | 4 | 2 | |||||||||||||||||
| H | 2 | 4 | 2 | ||||||||||||||||||
| V | 2 | 2 | |||||||||||||||||||
| X | 2 |
 |  |  |  |  | 
| ||||||||||||||||||||||||||||||||||||
 | 4 | 2 | 4 | 2 | 2 | 6
 3 | 2 | 6 | 2 | 2
|  4 | 6 | 5 | 6
|  2 | 4 | 2
|  2 | 5
|  6
| |
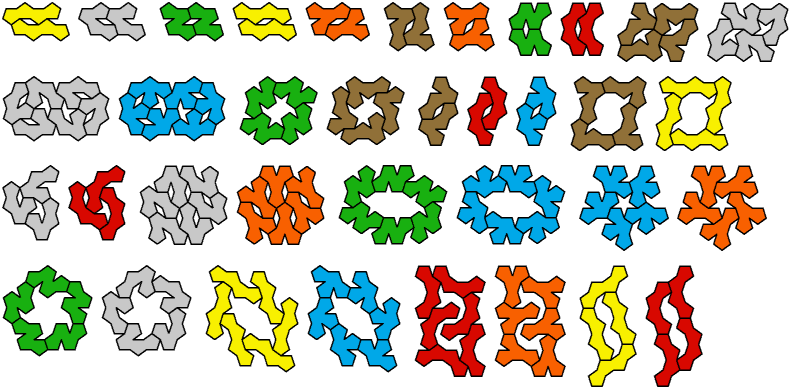
Then Scott Reynolds and George Sicherman tackled pentapents. The results can be seen at George Sicherman's site.
 |  |  |  |  | 
| ||||||||||||||||||||||||||||||||||||
 | 7 | 4 | 4 | 2 | 2 | 2
 4 | 4 | 6 | 7 | 6
|  4 | 6 | 2 | 4
|  2 | 4 | 4
|  2 | 6
|  7
| |
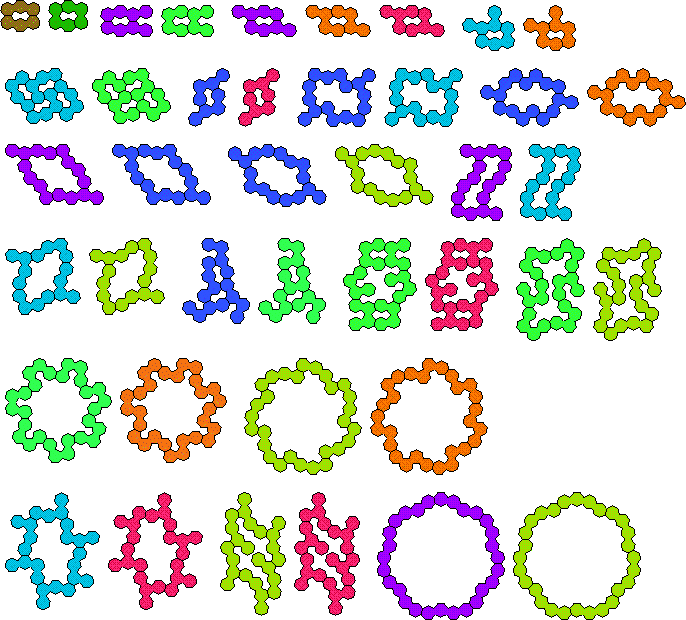
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 8/6/08.