

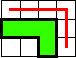
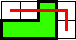
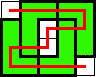
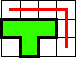
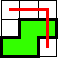
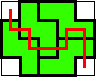
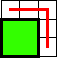
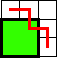
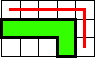
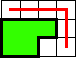
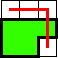
Although not a terribly hard puzzle, it got me (as a puzzle designer) thinking about how hard it was to produce such a puzzle without those pesky dark squares. In particular, given a polyomino P, and a non-negative integer n no greater than the area of P, what is the smallest rectangular puzzle containing some non-overlapping copies of P that has a unique path connecting opposite corners that intersects each copy of P in exactly n squares? We do not allow the path to start or end inside a copy of P.
What are the answers for the tetrominoes? Can you prove that many of the cases, especially with large n, are impossible? What about larger polyominoes? Is there a solution for every polyomino and n=1? Can you classify the polyominoes that have solutions where n is equal to the area of the polyomino? Can you classify the polyominoes that fail to have solutions for n=0?
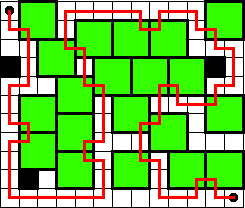
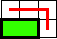
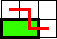
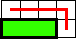
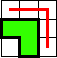
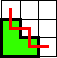
Here are the smallest known solutions for polyominoes of area 5 or less.
| P \ n | 0 | 1 | 2 | 3 |
|---|---|---|---|---|

| 
| 
| ||

| 
| 
| 
| |

| 
| 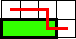
|  (George Sicherman) | 
|

| 
| 
| 
| 
|
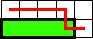
| P \ n | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|

| 
| 
| 
| ? | 
|

| 
| 
| 
| 
| 
|

| 
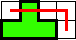
| 
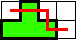
| 
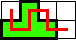
| ? | 
|

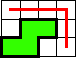
| 
| 
| 
| 
| 
|

| 
| 
| ? | ? | 
|
| 0 | 1 | 2 | 3 | 4 | 5 | |
|---|---|---|---|---|---|---|

| 
| 
| 
| 
| ? | 
|

| 
| 
| 
| ? | ? | 
|

| 
| 
| 
| 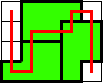 (George Sicherman) | 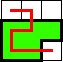 (George Sicherman) | 
|

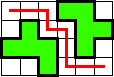
| 
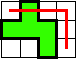
| 
| 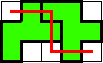 (George Sicherman) | ? | ? | 
|

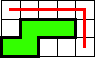
| 
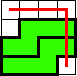
| 
| 
|  (George Sicherman) | ? | 
|

| 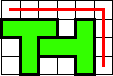 (Sune Kristian Jakobsen) | 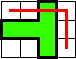
| 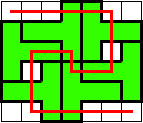 (George Sicherman) | ? | ? | 
|

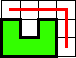
| 
| 
| 
| ? | ? | 
|

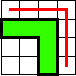
| 
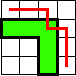
| 
| 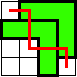
| 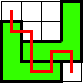 (George Sicherman) | ? | 
|

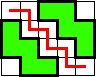
| 
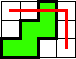
| 
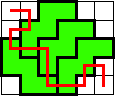
| 
| ? | ? | 
|

| 
| 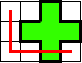 (George Sicherman) | ? | ? | ? | 
|

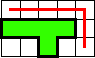
| 
| 
| 
| 
| ? | 
|

| 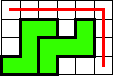 (Sune Kristian Jakobsen) | 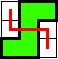
| 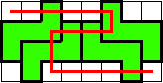 (George Sicherman) | ? | ? | 
|
Hexominoes:
All of the hexominoes have puzzles for n=0 using either 1 or 2 copies of P. Sune Kristian Jakobsen found the last case. They also all have solutions for n=1 using 1 or 2 copies of P. Most of them have solutions for n=2, but I couldn't find solutions for one of them. Gabriele Carelli found one of these. I found 14 hexominoes that needed more than 2 copies of P. In 2011, George Sicherman found one more. Can you improve any of them?
 | 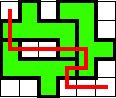 | 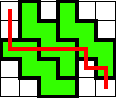 | 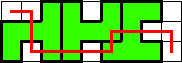 (George Sicherman) | 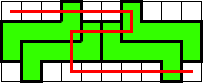 (George Sicherman) |
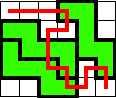 | 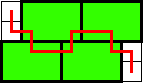 (George Sicherman) | 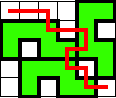 | 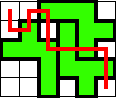 |  |
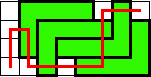 | 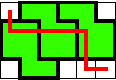 | 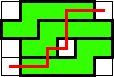 | 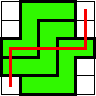 | 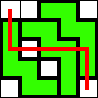 | 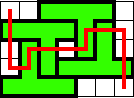 |
Sune Kristian Jakobsen found there are 9 heptominoes that need more than 2 copies of P, and 2 that have no solutions for n=0. Here are the smallest known solutions. Can you improve any of them?
 |  | 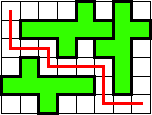 (Sune Kristian Jakobsen) | 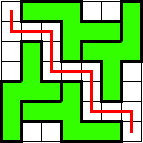 (George Sicherman) | 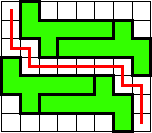 |
 (Sune Kristian Jakobsen) | 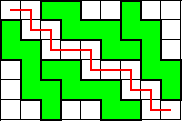 (Sune Kristian Jakobsen) | 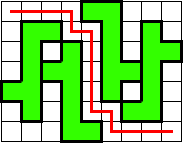 (George Sicherman) |
 | 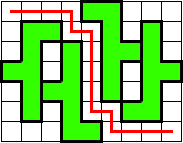 (George Sicherman) | 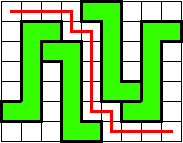 (George Sicherman) |
George Sicherman found that for n=1, all the heptominoes have solutions with no more than 3 tiles. For n=2, he found solutions for most of them, except for these:

Octominoes:
George Sicherman looked at octominoes for n=0, and found 8 that apparently need 6 copies, shown below. Can you improve any of these?
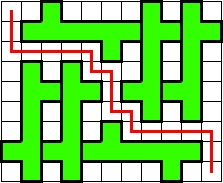 | 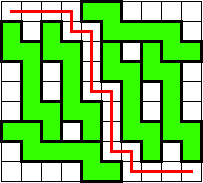 (George Sicherman) | 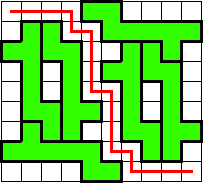 (George Sicherman) |
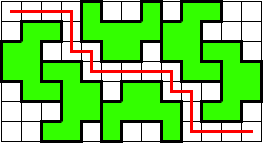 (George Sicherman) | 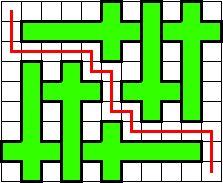 (George Sicherman) | 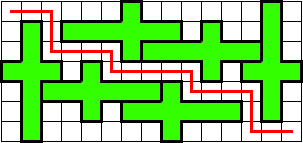 (George Sicherman) |
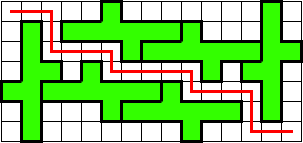 (George Sicherman) | 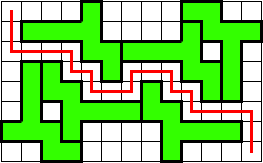 (George Sicherman) |
Here is the list of unsolved octominoes for n=0:
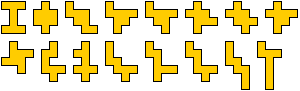
Sune Kristian Jakobsen found that this polyomino had no unique paths for any value of n.

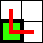
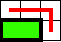
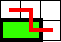
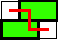
| polyomino | n=0 and n=1 solutions | n=1 and n=2 solutions |
|---|---|---|

|  
| 
|

|  
|  
|

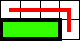
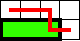
|  
|  
|

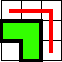
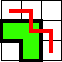
|  
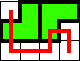
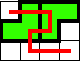
|  
|
| polyomino | n=0 and n=1 solutions | n=1 and n=2 solutions |
|---|---|---|

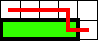
|  
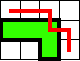
| ? |

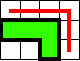
|  
| 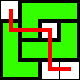 
|

|  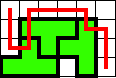
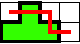
| 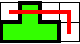 
|

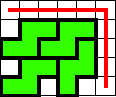
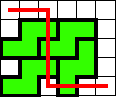
|  
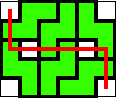
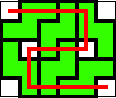
|  
|

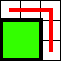
|  
| ? |
| polyomino | n=0 and n=1 solutions | n=1 and n=2 solutions |
|---|---|---|

|  
| 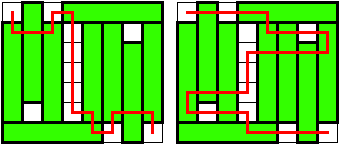 (George Sicherman) |

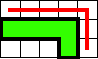
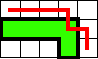
|  
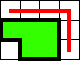
| 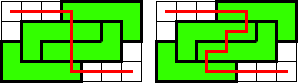 (George Sicherman) |

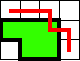
|  
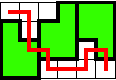
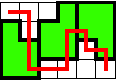
|  
|

| 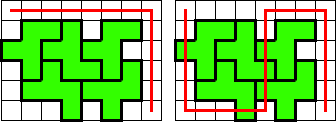 (George Sicherman) | 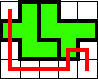 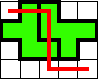
|

| 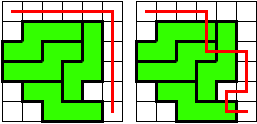 (George Sicherman) | 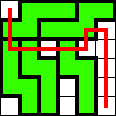 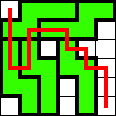
|

| 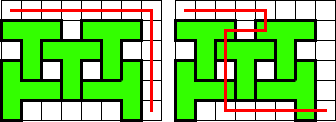 (George Sicherman) | ? |

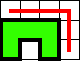
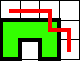
|  
| ? |

| 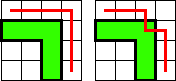 (George Sicherman) | ? |

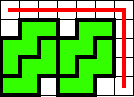
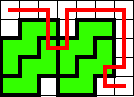
|  
| 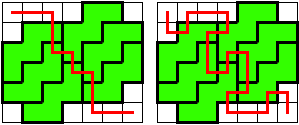 (George Sicherman) |

| 
| ? |

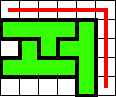
|  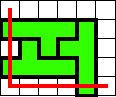
|  
|

| 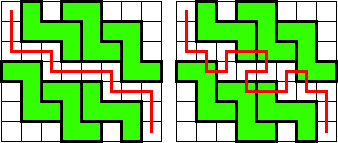 (George Sicherman) | 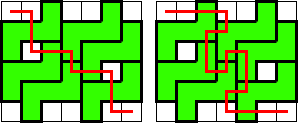 (George Sicherman) |
George Sicherman found that every hexomino has a joint n=0 and n=1 solution. He also found solutions for other pairs of n values:
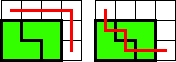 | 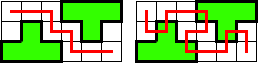 |
 | 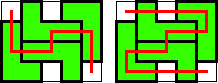 |
 |  |
 |  |
 |  |
George Sicherman also found these puzzles for n=0, n=1, and n=2:
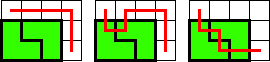 | 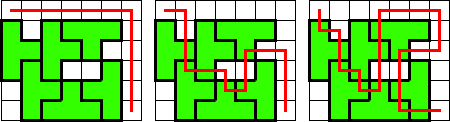 |
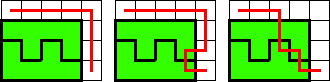 | 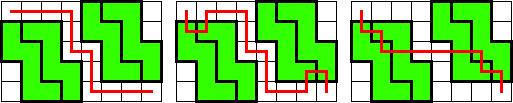 |
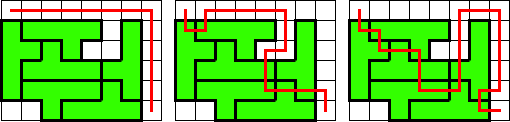 |
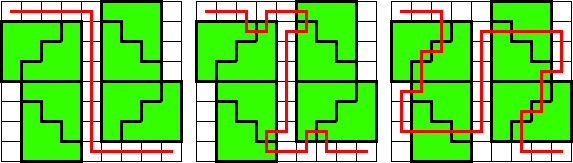 |
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 7/31/11.