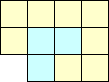
An { a₁, a₂, ... a_n } entanglement is a set of polyominoes with areas a₁, a₂, ... a_n which are entangled. Thus, the above figure is an {3,8} entanglement. What other entanglements exist?

{1,7+}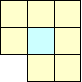
{2+,8+}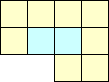
{2+,8+,8+}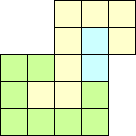
{2+,8+,8+,8+}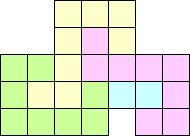
{1,8+,8+,8+,8+}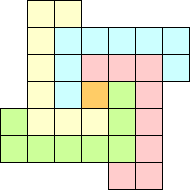
{6+,8+,8+,8+,8+}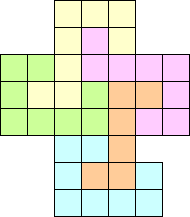
{6+,8+,8+,8+,8+,8+}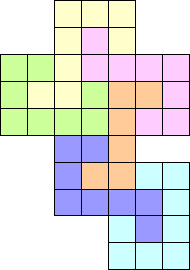
{6+,8+,8+,8+,8+,8+,8+}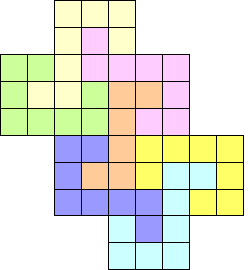

Joseph DeVincentis also found these entanglements using larger polyominoes:

{1,1,9+}
{1,1,1,1,11+}, {1,1,2+,10+}, {2,2+,10+}, {1,3+,9+}
{6+,6+,11+,11+}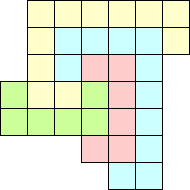
{12+,12+,12+,...}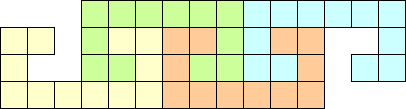

He also notes that to surround N² units worth of hole, you need either a (4N+3)-omino, a (2N+1)-omino and a (2N+7)-omino, or four (N+7)-ominoes.

Joseph Babcock found that any number of 11-ominoes can be entangled:

Claudio Baiocchi found a {2+,8+,9+,9+,9+,...} entanglement shown below:

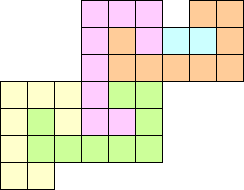
Berend Jan van der Zwaag found the {2,8+,8+,8+,8+...} entanglement below, answering a question of Joseph DeVincentis who asked whether arbitrarily many octominoes could be entangled. This can be modified to give a {1,7,8+,8+,8+,8+...} entanglement or a {2,7,9,8+,8+,8+} entanglement.

Berend Jan van der Zwaag showed that with a little help, arbitrarily many hexominoes could be entangled in a {3+,9+,6+,6+,6+,...} entanglement:

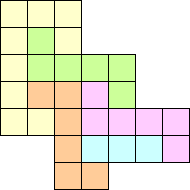
He also found this {7,7,7,9,9+,9+,9+,9+} entanglement:

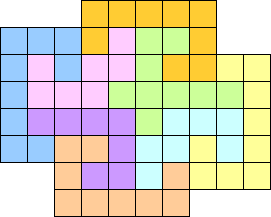
Jeremy Galvagni found a extendable configuration which he calls borromean, since the removal of any polyomino disconnects them all:

Zoltan Nemeth completely classified the entanglements using two polyominoes.

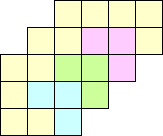
I found the {1,2,3,4,5,6,7,8,9,10} entanglement below.

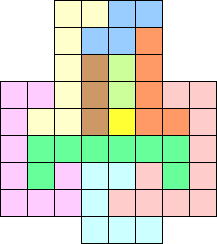
I thought that no {1,2,3,...,n} entanglement existed for n<10. But then Berend Jan van der Zwaag sent me the following {1,2,3,4,5,6,7,8,9} entanglement.

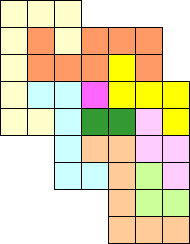
Sasha Ravsky proved that no {1,2,3,4,5,6} entanglement exists.

Guenter found a 12-omino that tiles the plane in an entangled way, but thought that 10 copies are needed to be entangled:

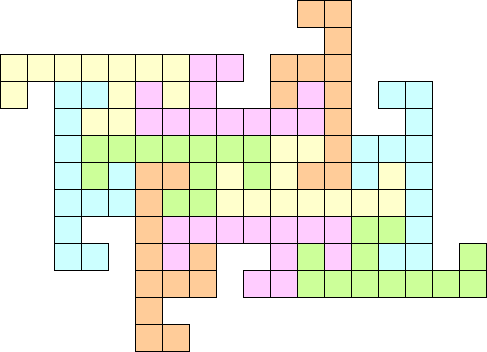
Joseph DeVincentis recently discovered these entanglements, one of which shows that {1,7} is not the only entanglement that only uses polyominoes of area 7 or less. These are the entanglements {5,7+,7+,7+,7+}, {1,1,3,7,8+,8+,8+,8+}, {7+,7+,7+,7+,8}, and {5+,5+,5+,5+,7+,7+,7+,7+,8}.

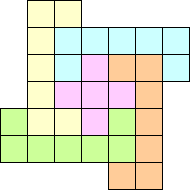
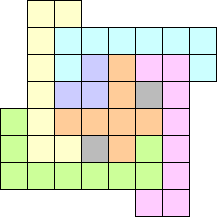
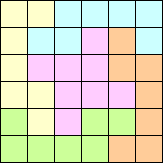
Even more recently, Joseph DeVincentis discovered entanglements using only area 6 or less. These are the entanglements {2,2,2,2,4,5+,5+,5+,5+,6,6,6,6,6+,6+,6+,6+,6+,6+,6+,6+} and {4+,4+,4+,4+,5,5+,5+,5+,5+,6,6,6,6,6+,6+,6+,6+}.

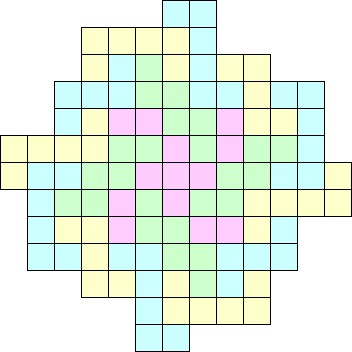
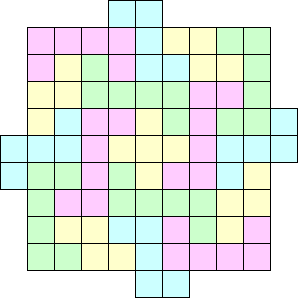
Berend Jan van der Zwaag discovered arbitrarily large entanglements using only 4 hexominoes and lots of smaller polyominoes:

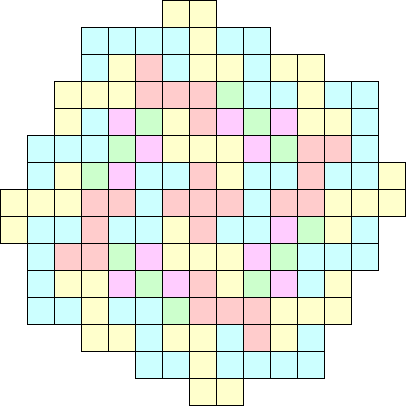
Sasha Ravsky tried to prove that all entanglements contain an n-omino with n≥6, but Boris Bukh found an error in the proof.

If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 12/21/02.