Problem of the Month (October 2014)

If n unit squares are placed into a square of area n, what is the smallest possible maximum area of overlap of two of the squares? What if the n squares are placed in a square of integer side m, with m2 ≤ n ? More generally, how do you place unit squares in the plane with density δ with the smallest possible overlap?

ANSWERS

Below are the placements of squares with the smallest known overlaps:

n Squares in a Square of Area n
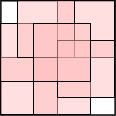
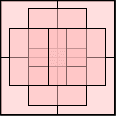
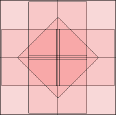
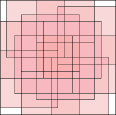
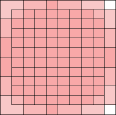
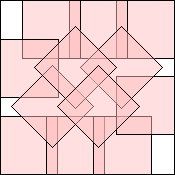
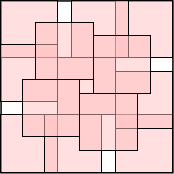
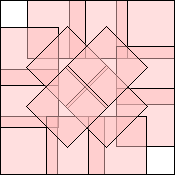
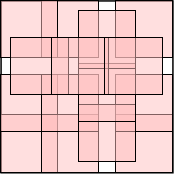
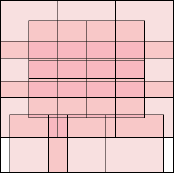
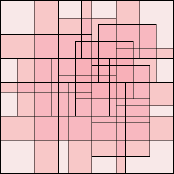
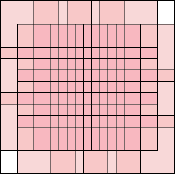
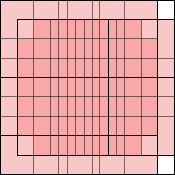
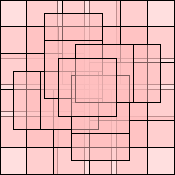
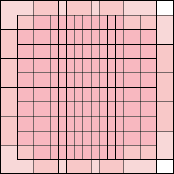
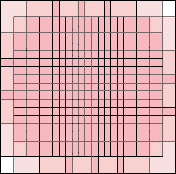
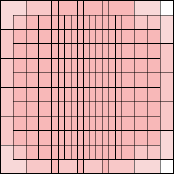
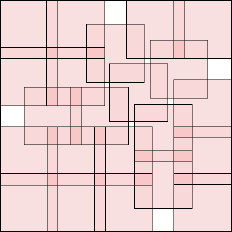
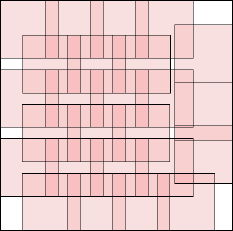
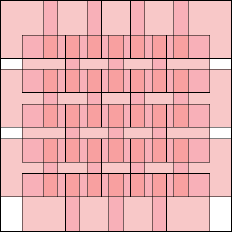
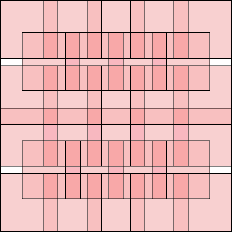
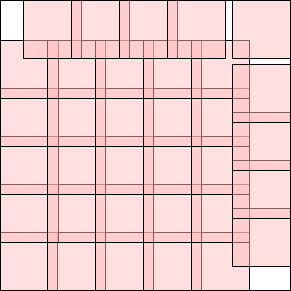
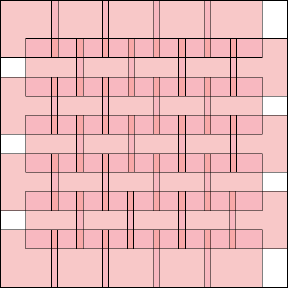
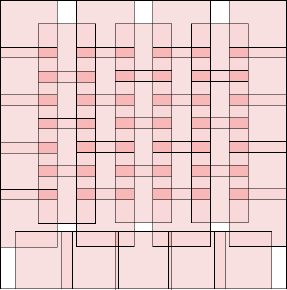
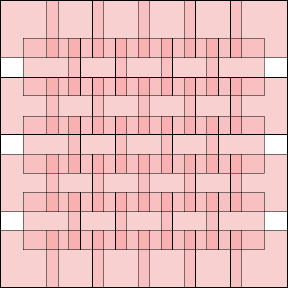
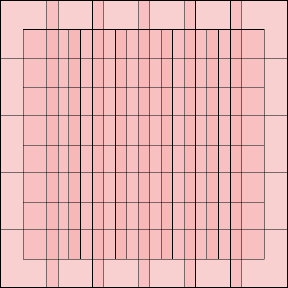
n=1

0 		n=2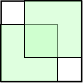

6 – 4√2
.343+ 		n=3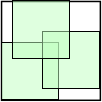

21 – 12 √3
.215+ 		n=4

0 		n=5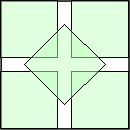

.110+
(M. Morandi) 		n=6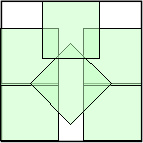

.132+
(M. Morandi) 		n=7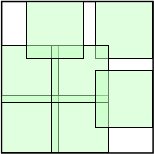

.127+
(M. Morandi) 		n=8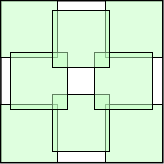

.070+ 		n=9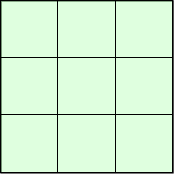

0
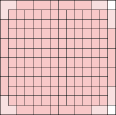
n=10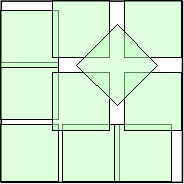

.092+
(Maurizio Morandi) 		n=11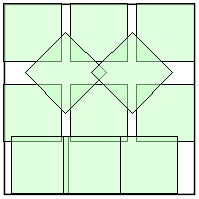

.087+
(Maurizio Morandi) 		n=12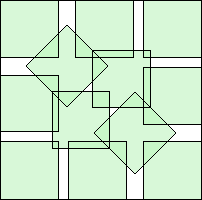

.085+
(Maurizio Morandi) 		n=13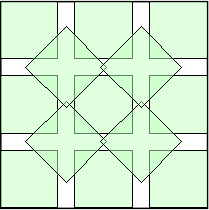

.081+
(Maurizio Morandi) 		n=14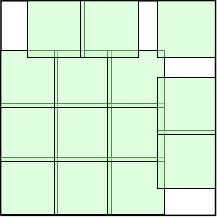

.066+
(Maurizio Morandi)
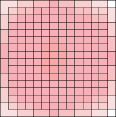
n=15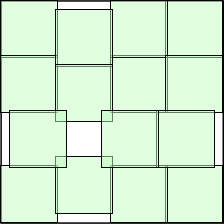

.037+ 		n=16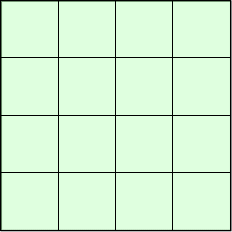

0 		n=17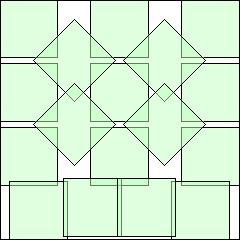

.070+
(Joe DeVincentis) 		n=18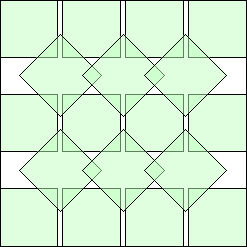

.063+
(Joe DeVincentis) 		n=19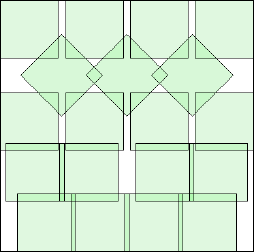

.073+
(Maurizio Morandi)
n=20

.066+
(Joe DeVincentis) 		n=21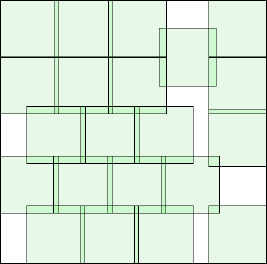

.070+
(Maurizio Morandi) 		n=22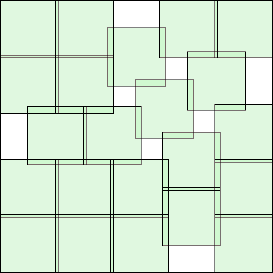

.053+
(Maurizio Morandi) 		n=23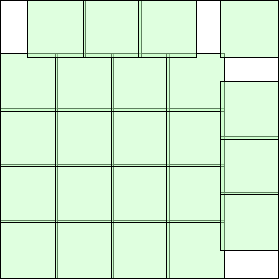

.041+
(Joe DeVincentis)
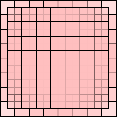
n=24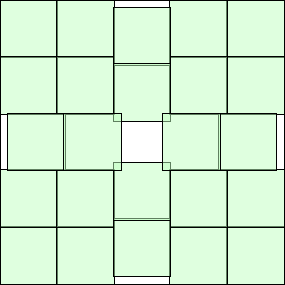

.023+
(Joe DeVincentis) 		n=25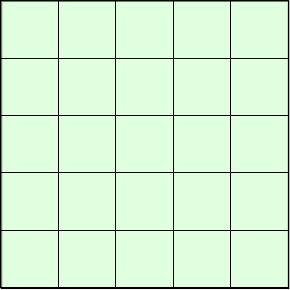

0 		n=26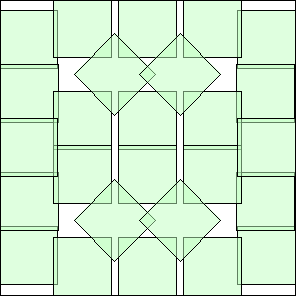

.062+
(Joe DeVincentis) 		n=27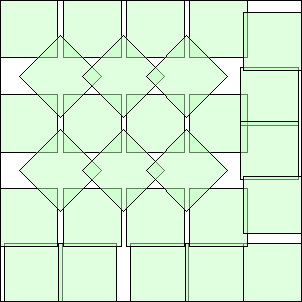

.059+
(Joe DeVincentis)

n Squares in a Square of Side 2
n=4

0
  		n=5

1/4
  		n=6

1/3
  		n=7

√2 – 1
(M. Morandi) 		n=8

(7–√13)/8
  		n=9

(3+2√2)/12
(M. Morandi) 		n=10

1/2
(M. Morandi) 		n=11

2–√34/4
(M. Morandi) 		n=12

58–10√33
(M. Morandi) 		n=13

(√17–3)/2
(M. Morandi)
n=15

3/5
(M. Morandi) 		n=18

16/25
(M. Morandi) 		n=21

2/3
(M. Morandi) 		n=25

25/36
(J. DeVincentis) 		n=28

5/7
(M. Morandi) 		n=32

36/49
(M. Morandi) 		n=36

3/4
(M. Morandi) 		n=41

49/64
(J. DeVincentis) 		n=45

7/9
(M. Morandi) 		n=50

64/81
(M. Morandi)

n Squares in a Square of Side 3
n=9

0
  		n=10

.143+
(Maurizio Morandi) 		n=11

.183+
(Maurizio Morandi) 		n=12

.197+
(Joe DeVincentis) 		n=13

√5 – 2
(Bryce Herdt) 		n=14

.274+
(Joe DeVincentis) 		n=15

.289+
(Maurizio Morandi)
n=16

(21–√249)/16
(Maurizio Morandi) 		n=18

1/3
(Maurizio Morandi) 		n=21

2/5
(Maurizio Morandi) 		n=22

√2–1
(Maurizio Morandi) 		n=24

3/7
(Maurizio Morandi) 		n=25

4/9
  		n=28

10/21
(Maurizio Morandi)
n=32

1/2
(Joe DeVincentis) 		n=33

(√13–2)/3
(Joe DeVincentis) 		n=36

15/28
(Maurizio Morandi) 		n=40

5/9
(Maurizio Morandi) 		n=41

9/16
(Joe DeVincentis) 		n=45

7/12
(Maurizio Morandi) 		n=50

3/5
(Joe DeVincentis)

n Squares in a Square of Side 4
n=16

0
  		n=17

.092+
(Maurizio Morandi) 		n=18

.104+
(Bryce Herdt) 		n=19

.133+
(Joe DeVincentis) 		n=20

.139+
(Joe DeVincentis)
n=21

.179+
(Maurizio Morandi) 		n=22

(√33–5)/4
(Maurizio Morandi) 		n=23

3/14
(Joe DeVincentis) 		n=24

.221+
(Joe DeVincentis) 		n=25

(11–√73)/10
(Maurizio Morandi)
n=27

1/4
(Maurizio Morandi) 		n=28

10/37
(Maurizio Morandi) 		n=30

4/13
(Maurizio Morandi) 		n=32

5/16
(Maurizio Morandi) 		n=35

1/3
(Maurizio Morandi)
n=36

5/14
(Maurizio Morandi) 		n=40

8/21
(Maurizio Morandi) 		n=41

25/64
(Maurizio Morandi) 		n=44

2/5
(Maurizio Morandi) 		n=45

5/12
(Maurizio Morandi)
n=46

.434+
(Joe DeVincentis) 		n=50

7/16
(Joe DeVincentis)

n Squares in a Square of Side 5
n=25

0
  		n=26

.076+
(Joe DeVincentis) 		n=27

3/2–√2
(Maurizio Morandi) 		n=28

.093+
(Maurizio Morandi)
n=29

.107+
(Joe DeVincentis) 		n=30

1/9
(Joe DeVincentis) 		n=32

(19–6√2)/72
(Maurizio Morandi) 		n=33

.151+
(Joe DeVincentis)
n=34

.175+
(Joe DeVincentis) 		n=35

5/27
(Maurizio Morandi) 		n=37

(47–√1409)/48
(Maurizio Morandi) 		n=39

1/5
(Maurizio Morandi)
n=40

5/21
(Maurizio Morandi) 		n=42

1/4
(Maurizio Morandi) 		n=44

9/35
(Maurizio Morandi) 		n=48

3/11
(Maurizio Morandi)
n=50

3/10
(Maurizio Morandi)

If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 10/30/14.