Problem of the Month (November 2014)

How can n equilateral triangles of one side and m of another side be packed into an equilateral triangle to fill the maximum area? Clearly when n, m, or n+m is a square number, the entire triangle can be filled. What about the more interesting cases?

ANSWERS

Solutions were received from Maurizio Morandi, George Sicherman, Bryce Herdt, and Joe DeVincentis.

Largest Area Covered by Triangles of Two Sizes
n \ m 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18
1 1
2 1 1
3 1 7/8 15/16
4 1 1 1 1
5 1 .818+
(MM) 		.917+ 1 125/144
6 1 8/9 1 1 67/72 68/75
7 1 1 17/18 1 .919+
(MM) 		17/18 35/36
8 1  1  35/36 1 .963+ .964+
(MM) 		 1  1
9 1 1 1 1 1 1 1 1 1
10 1 29/32 187/196 1 15/16 1 47/49
(MM) 		48/49 1 449/490
(JD)
11 1 47/50 .948+ 1 1 49/50 73/75 .977+ 1 139/144 .941+
(MM)
12 1 59/64
(JD) 		24/25 1 237/256
(MM) 		 1  139/144
(JD) 		120/121 1 23/24 383/400 24/25
13 1 15/16 1 1 137/144 379/400
(GS) 		115/121 .979+
(BH) 		1 31/32 63/64 1 377/392
(MM)
14 1 1 31/32 1 .954+
(MM) 		31/32 63/64  1  1 39/40 1 251/256
(JD) 		109/112
(MM) 		.976+
(MM)
15 1 31/32 63/64 1 .979+ .979+
(MM) 		71/72 143/144 1 1 .987+
(BH) 		.987+
(BH) 		.988+
(BH) 		127/128 255/256
16 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1
17 1  1 
(JD) 		.932+
(MM) 		1 .934+
(MM) 		249/256
(MM) 		80/81
(MM) 		1 1 26/27
(MM) 		79/81
(MM) 		80/81
(MM) 		 1 
(MM) 		143/144
(MM) 		.990+
(MM) 		1 221/225
18 1  1 
(JD) 		35/36
(MM) 		1 117/121
(JD) 		26/27
(MM) 		1  1 
(MM) 		1 162/169
(MM) 		31/32
(MM) 		35/36
(MM) 		27/28
(MM) 		 1 
(MM) 		143/144
(MM) 		1  1 
(MM) 		 1 

Largest Area Covered by Triangles of Two Sizes with m=n

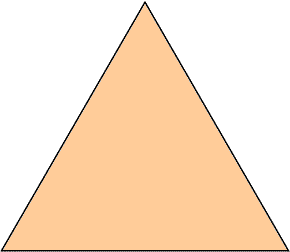
T(1) = 1
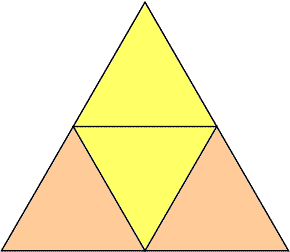
T(2) = 1
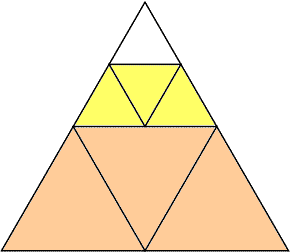
T(3) ≥ 15/16
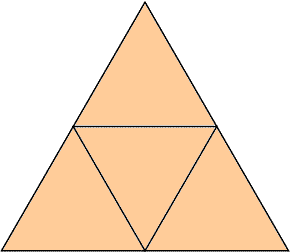
T(4) = 1

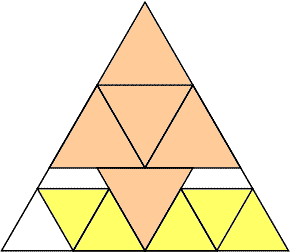
T(5) ≥ 125/144
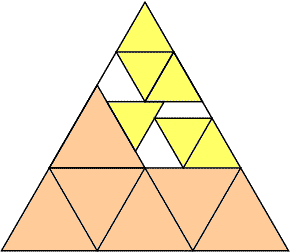
T(6) ≥ 68/75
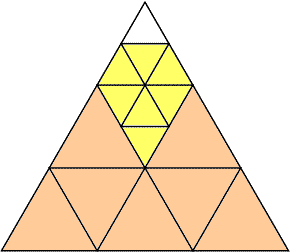
T(7) ≥ 35/36
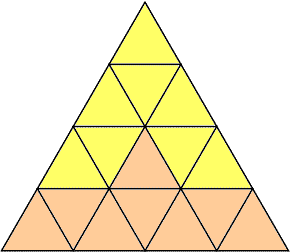
T(8) = 1

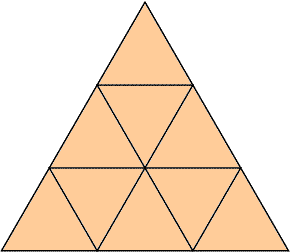
T(9) = 1
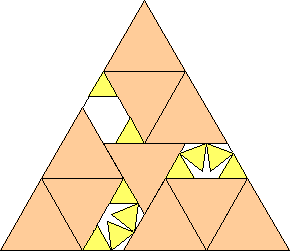
T(10) ≥ 449/490 (JD)
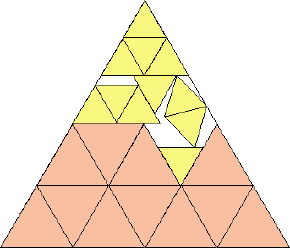
T(11) ≥ .941+ (MM)
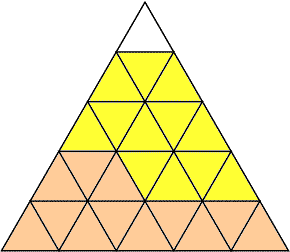
T(12) ≥ 24/25

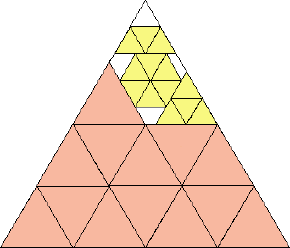
T(13) ≥ 377/392 (MM)
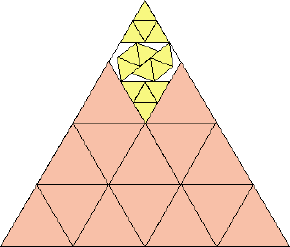
T(14) ≥ .976+ (MM)
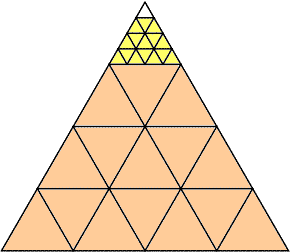
T(15) ≥ 255/256
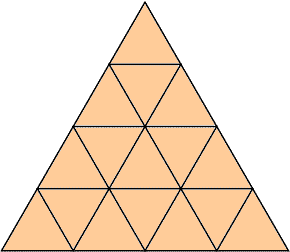
T(16) = 1

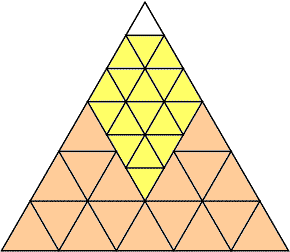
T(17) ≥ 221/225
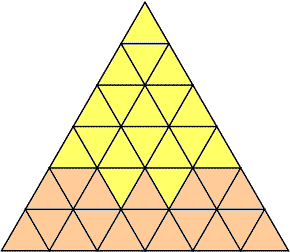
T(18) = 1
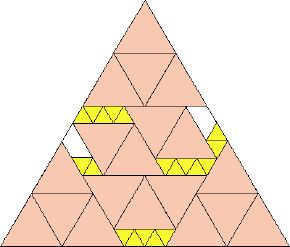
T(19) ≥ 95/98 (MM)
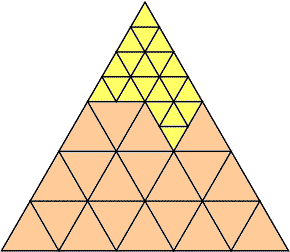
T(20) = 1

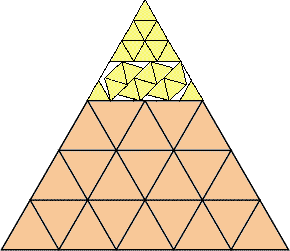
T(21) ≥ .978+ (MM)
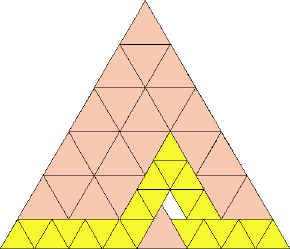
T(22) ≥ 286/289 (MM)
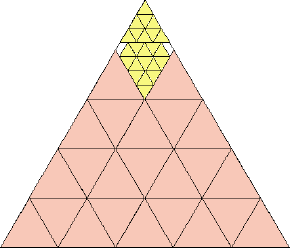
T(23) ≥ 1219/1225 (MM)
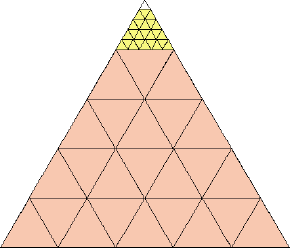
T(24) ≥ 624/625 (MM)

If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 11/11/14.