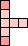
(2,2)
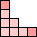
(1,1)
(0,3)
(0,2)
(0,1)
(1,4)
(2,3)
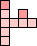
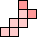
(1,3)
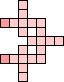
(GS)

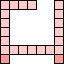









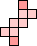
(GR)
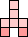

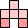






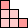
(GS)

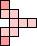








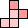
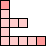




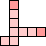

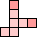
(1,3)
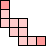





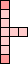
(2,3)
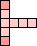
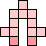
(GR)


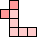

(1,4)
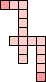
(GS)


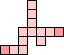
(GR)

(0,1)
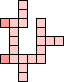

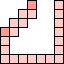

(0,2)


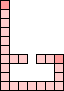
(0,3)
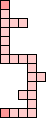
(BH)
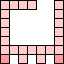
(1,1)
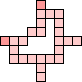
(GS)
1.
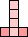
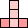
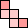
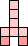
Given a collection of 2 or more chess pieces, what is the smallest polyomino with a starting square and an ending square so that it takes the same minimum number of moves by each piece to get from start to finish? What about 3 pieces? What about including other fairy chess pieces, such as these leapers: Alfil (2,2), Fers (1,1), Threeleaper (0,3), Dabbaba (0,2), Wazir (0,1), Giraffe (1,4), Zebra (2,3), Camel (1,3)?
2.
Given a polyomino containing one chess piece P, what is the smallest polyomino that can be tiled with copies of P so that 1) no chess piece threatens any other and 2) every vacant square is threatened by at least one piece? What about larger polyominoes? What if the polyominoes contain more than one chess piece?
3.
Given a polyomino containing one chess piece P, what is the smallest polyomino that can be tiled with copies of P so that every square is attacked the same number of times? What about larger polyominoes? What if we only require the empty squares to be attacked the same number of times?
4.
What is the smallest polynomial that contains a "mate in n" chess problem with a unique solution? What if a unique solution is not required?
1.
The best-known results are shown below.
| Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) | Wazir (0,1) | Giraffe (1,4) | Zebra (2,3) | Camel (1,3) | Rook | kNight | Bishop | Queen | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| King |  (GS) |  |  |  |  |  |  |  |  |  |  | 
|
| Queen |  |  |  |  |  |  |  (GR) |  |  |  | 
| |
| Bishop |  |  |  |  |  (GS) |  |  |  |  | 
| ||
| kNight |  |  |  |  |  |  |  |  | 
| |||
| Rook |  |  |  |  |  |  |  | 
| ||||
| Camel (1,3) |  |  |  |  |  |  | 
| |||||
| Zebra (2,3) |  | 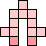 (GR) |  |  |  | 
| ||||||
| Giraffe (1,4) |  (GS) |  |  | 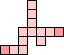 (GR) | 
| |||||||
| Wazir (0,1) |  |  |  | 
| ||||||||
| Dabbaba (0,2) |  |  | 
| |||||||||
| Threeleaper (0,3) |  (BH) | 
| ||||||||||
| Fers (1,1) |  (GS) |
| King+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) | Wazir (0,1) | Giraffe (1,4) | Zebra (2,3) | Camel (1,3) | Rook | kNight | Bishop |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Queen | 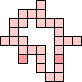 (BH) |  (GR) | 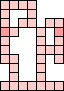 (GS) |  (GR) |  (GR) |  (GR) | 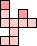 (GR) |  (GR) |  (GR) |  (GR) |  (GR) |
| Bishop | 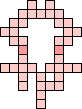 (GS) |  (GR) | 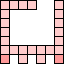 (GS) | 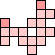 (GR) |  (GR) |  (GR) | 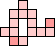 (GR) |  (GR) |  (GR) |  (GR) | |
| kNight | 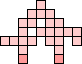 (JD) |  (GR) | 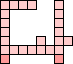 (GS) | 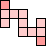 (GR) |  (GR) |  (GR) | 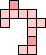 (GR) |  (GR) |  (GR) | ||
| Rook |  (GS) |  (GR) | 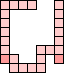 (GS) |  (GR) |  (GR) | 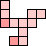 (GR) | 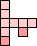 (GR) | 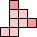 (GR) | |||
| Camel (1,3) | 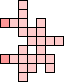 (GS) |  (GR) | 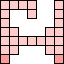 (GS) | 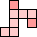 (GR) |  (GR) |  (GR) | 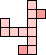 (GR) | ||||
| Zebra (2,3) | 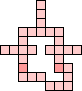 (GS) | 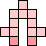 (GR) | 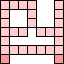 (GS) | 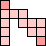 (GR) | 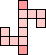 (GR) | 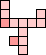 (GR) | |||||
| Giraffe (1,4) | 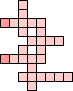 (GS) |  (GR) | 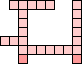 (GS) | 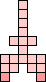 (GS) |  (GR) | ||||||
| Wazir (0,1) | 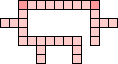 (GS) |  (GR) | none (JD) | none (JD) | |||||||
| Dabbaba (0,2) | 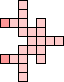 (GS) |  (GR) | 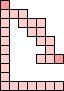 (JD) | ||||||||
| Threeleaper (0,3) | 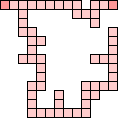 (GS) | 
| |||||||||
| Fers (1,1) | 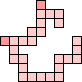 (JD) |
| Queen+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) | Wazir (0,1) | Giraffe (1,4) | Zebra (2,3) | Camel (1,3) | Rook | kNight |
|---|---|---|---|---|---|---|---|---|---|---|
| Bishop | 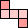 (GR) |  (GR) | 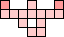 (GR) | 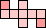 (GR) | none (BH) | 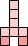 (GR) | 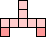 (GR) | 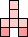 (GR) |  (GR) |  (GR) |
| kNight | 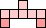 (GR) |  (GR) | 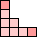 (GR) | 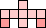 (GR) | none (BH) |  (GR) | 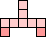 (GR) |  (GR) | 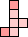 (GR) | |
| Rook | 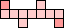 (GR) |  (GR) |  (GR) |  (GR) |  (GR) | 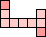 (GR) | 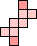 (GR) | 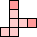 (GR) | ||
| Camel (1,3) | 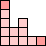 (GR) |  (GR) | 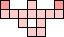 (GR) |  (GR) | none (BH) |  (GR) | 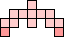 (GR) | |||
| Zebra (2,3) | 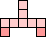 (GR) |  (BH) | 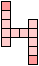 (GR) | 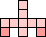 (GR) | none (BH) | 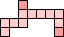 (GR) | ||||
| Giraffe (1,4) | 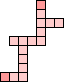 (GR) |  (GR) | 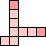 (GR) | 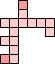 (GR) | none (BH) | |||||
| Wazir (0,1) | none (BH) | none (BH) | none (BH) | none (BH) | ||||||
| Dabbaba (0,2) |  (GR) | none (BH) | 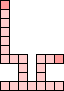 (BH) | |||||||
| Threeleaper (0,3) | 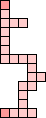 (BH) | 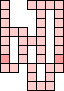 (GS) | ||||||||
| Fers (1,1) | none (BH) |
| Bishop+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) | Wazir (0,1) | Giraffe (1,4) | Zebra (2,3) | Camel (1,3) | Rook |
|---|---|---|---|---|---|---|---|---|---|
| kNight |  (GR) |  (GR) | 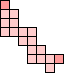 (GS) |  (GR) |  (GR) |  (GR) |  (GR) |  (GR) |  (GR) |
| Rook |  (GR) |  (GR) | 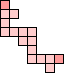 (GR) | 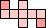 (GR) | none (GS) | 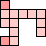 (GR) | 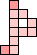 (GR) | 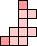 (GR) | |
| Camel (1,3) | 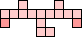 (GR) |  (GR) | 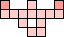 (GR) |  (GR) |  (GR) |  (GR) |  (GR) | ||
| Zebra (2,3) |  (GR) | 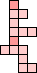 (GR) | 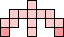 (GR) | 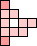 (GR) | 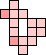 (GR) | 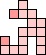 (GR) | |||
| Giraffe (1,4) | 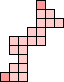 (GS) |  (BH) | 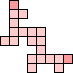 (GS) | 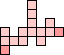 (GR) |  (GR) | ||||
| Wazir (0,1) | 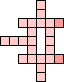 (GS) |  (GR) | 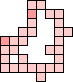 (GS) | 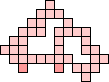 (GS) | |||||
| Dabbaba (0,2) |  (GR) | none (GS) | 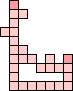 (GS) | ||||||
| Threeleaper (0,3) | 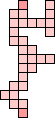 (GS) |  (GR) | |||||||
| Fers (1,1) | 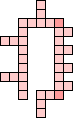 (GS) |
| kNight+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) | Wazir (0,1) | Giraffe (1,4) | Zebra (2,3) | Camel (1,3) |
|---|---|---|---|---|---|---|---|---|
| Rook | 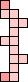 (GR) | 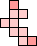 (GR) | 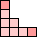 (GR) | 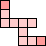 (GR) |  (GR) | 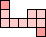 (GR) | 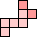 (GR) | 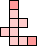 (GR) |
| Camel (1,3) |  (GR) |  (GR) | 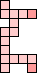 (GR) | 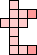 (GR) |  (GR) |  (GR) | 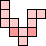 (GR) | |
| Zebra (2,3) | 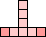 (GR) | 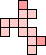 (GR) |  (GR) |  (GR) |  (GR) | 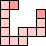 (GR) | ||
| Giraffe (1,4) | 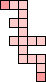 (GS) |  (GR) | 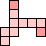 (GR) | 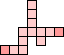 (GR) |  (GR) | |||
| Wazir (0,1) | 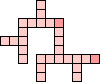 (GS) |  (GR) | 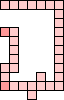 (GS) | 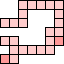 (GS) | ||||
| Dabbaba (0,2) |  (GR) | 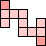 (GR) | 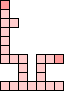 (BH) | |||||
| Threeleaper (0,3) | 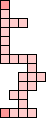 (BH) | 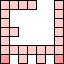 (GS) | ||||||
| Fers (1,1) | 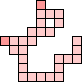 (GS) |
| Rook+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) | Wazir (0,1) | Giraffe (1,4) | Zebra (2,3) |
|---|---|---|---|---|---|---|---|
| Camel (1,3) | 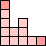 (GR) | 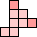 (GR) | 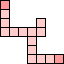 (GR) | 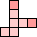 (GR) |  (GR) |  (GR) | 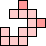 (GR) |
| Zebra (2,3) |  (GR) | 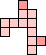 (GR) | 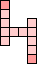 (GR) | 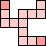 (GR) |  (GR) | 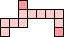 (GR) | |
| Giraffe (1,4) | 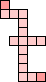 (GR) | 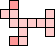 (GR) | 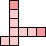 (GR) | 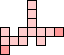 (GR) | 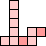 (GR) | ||
| Wazir (0,1) | none (GS) | none (GS) | 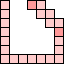 (GS) | 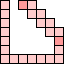 (GS) | |||
| Dabbaba (0,2) | 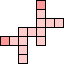 (GR) | 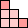 (GR) | 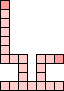 (BH) | ||||
| Threeleaper (0,3) | 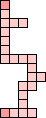 (BH) | 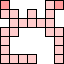 (GS) | |||||
| Fers (1,1) |  (GS) |
| Camel+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) | Wazir (0,1) | Giraffe (1,4) |
|---|---|---|---|---|---|---|
| Zebra (2,3) |  (GS) | 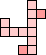 (GR) | 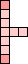 (GR) | 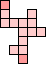 (GR) |  (GR) | 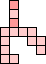 (GR) |
| Giraffe (1,4) | 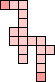 (GS) |  (GR) | 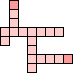 (GS) | 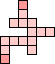 (GR) |  (GR) | |
| Wazir (0,1) | 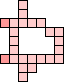 (GS) |  (GR) | 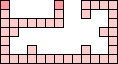 (GS) | 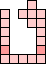 (GS) | ||
| Dabbaba (0,2) | 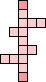 (GR) | 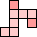 (GR) | 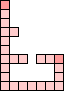 (BH) | |||
| Threeleaper (0,3) | 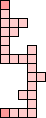 (GS) | 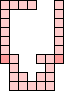 (GS) | ||||
| Fers (1,1) | 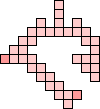 (GS) |
| Zebra+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) | Wazir (0,1) |
|---|---|---|---|---|---|
| Giraffe (1,4) | 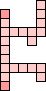 (GS) | 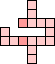 (GR) | 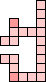 (GS) | 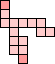 (GR) | 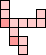 (GR) |
| Wazir (0,1) | 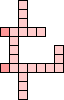 (GS) | 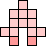 (GR) | 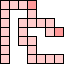 (GS) |  (GS) | |
| Dabbaba (0,2) | 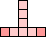 (GR) | 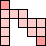 (GR) | 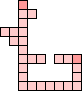 (GS) | ||
| Threeleaper (0,3) | 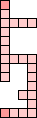 (GS) | 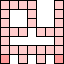 (GS) | |||
| Fers (1,1) | 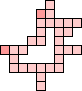 (GS) |
| Giraffe+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) | Dabbaba (0,2) |
|---|---|---|---|---|
| Wazir (0,1) | 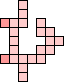 (GS) |  (GR) | 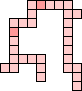 (GS) | 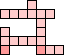 (GS) |
| Dabbaba (0,2) | 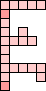 (GS) | 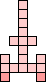 (GS) | 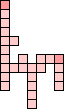 (GS) | |
| Threeleaper (0,3) | 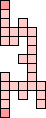 (GS) | 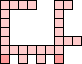 (GS) | ||
| Fers (1,1) | 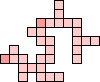 (GS) |
| Wazir+ | Alfil (2,2) | Fers (1,1) | Threeleaper (0,3) |
|---|---|---|---|
| Dabbaba (0,2) |  (GS) | 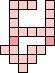 (GS) | 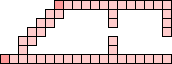 (GS) |
| Threeleaper (0,3) | 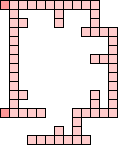 (GS) | 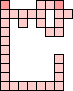 (GS) | |
| Fers (1,1) | 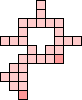 (GS) |
| Dabbaba+ | Alfil (2,2) | Fers (1,1) |
|---|---|---|
| Threeleaper (0,3) | 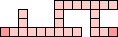 (GS) | 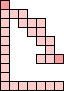 (GS) |
| Fers (1,1) | 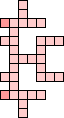 (GS) |
| Threeleaper+ | Alfil (2,2) |
|---|---|
| Fers (1,1) |  (GS) |
|
2.
The best-known results are shown below.
|
The best-known results are shown below.
|
Richard Sabey pointed out that a board with a "mate in n" also has all smaller mates, so we only show the longest mate on any size board. The best-known results are shown below.
|
|
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 12/27/10.