Problem of the Month (December 2014)
Given a polyomino, what is the smallest square it can tile, if at most one square is allowed to hang off each edge of the square? More generally, what are the smallest rectangles that can be tiled, with at most one square hanging off the side of each side? For those polyominoes that can form overlap squares or rectangles, what sizes can they make?
ANSWERS
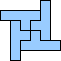
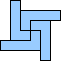
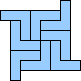
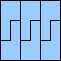
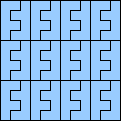
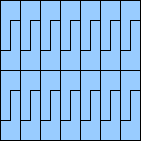
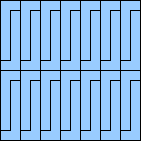
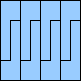
Smallest Squares With Possible Overlaps
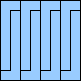
| 
| 
| 
(George Sicherman)
| 
(George Sicherman)
|
|
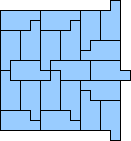
Smallest Rectangles With Possible Overlaps
George Sicherman considered the problem of tiling a square with at most one square missing from each side:
Smallest Squares With Possible Dents
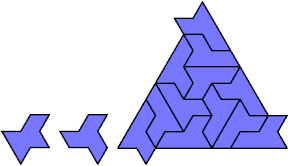
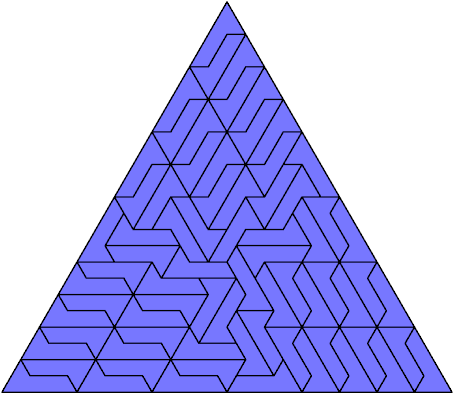
George Sicherman also considered the problem of polyiamonds tiling a triangle with possible overlap:
Smallest Triangles With Possible Overlaps
| 7
| 
| 8
| 
|
|
If you can extend any of these results, please
e-mail me.
Click here to go back to Math Magic. Last updated 12/1/14.