30 Two-Colored Points with
No Empty Monochromatic
Convex Fourgons

Erich Friedman
Stetson University, DeLand, FL 32723
efriedma@stetson.edu

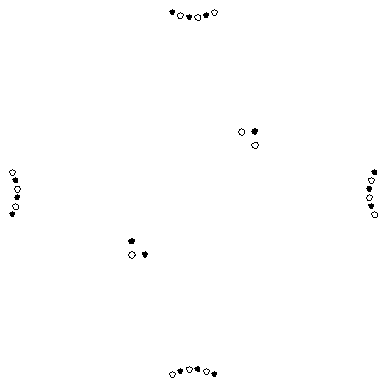
In [2], the authors consider the problem of finding the maximum number of points colored with 2 colors that contain no empty monochromatic convex fourgons, and gave the lower bound of 18. In [1], the author increased this lower bound by giving such a configuration of 20 points. We exhibit below 30 two-colored points, no three points colinear, with no empty monochromatic convex fourgons.

References

[1] P. Brass, Empty monochromatic fourgons in two-colored point sets. Geombinatorics XIV (2004) 5-7.

[2] O. Devillers, F. Hurtado, G. K‡rolyi, and C. Seara, Chromatic variants of the Erdšs-Szekeres theorem. Comput. Geom. Theory Appl. 26 (2003) 193-208.