The authors of [2] define a Heesch tile with Heesch number r to be a tile T which can be surrounded r times but not r+1 times by tiles congruent to T. Let us define the surround number of a tile T to be the minimum number of such tiles needed to surround T once. The author of [3] asks whether there exist Heesch tiles with Heesch number 1 and surround number N=2, 3, or 4. We illustrate such tiles for N=3 and N=4. We conjecture that there is no such tile for N=2.
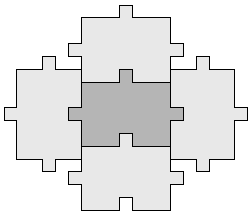
The N=4 example is shown in Figure 1. It is a 5x7 rectangle with three 1x1 additions and one 1x1 hole. It is clear that this tile is a Heesch tile with Heesch number 1.

The N=3 example is more complicated. It is essentially a polygon made of 28 equilateral triangles, except that small semicircles are added to the convex sides, and removed from the concave sides.
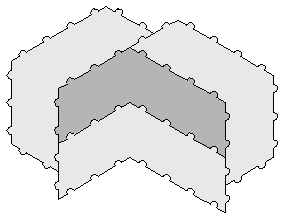
To show that this is indeed a Heesch tile with Heesch number 1, we start with the following observation: semicircular bumps in a straight line can only be completely covered by a single tile. If two tiles are used to cover the bumps, the region between these tiles cannot be covered (see Figure 3). Therefore five or more bumps cannot be completely covered, and four bumps can be covered only by the long concave side.

We now show that this tile T cannot be surrounded twice with tiles congruent to T. There are two ways to cover the four bumps on the long side of T. One results in a figure with five bumps in a row, which cannot be covered. The other results in a figure with 2 adjacent sides with four bumps in a row, which also cannot be covered. Therefore T has Heesch number 1.
[2] B. Gunbaum and G.C. Shephard, Tilings and Patterns. W.H. Freeman and Company, New York, 1987.
[3] P. Raedschelders, "Heesch Tiles Based on Regular Polygons". Geombinatorics, 7 (1998), 101-106.
[4] M. Senechal, Quasicrystals and Geometry, Cambridge Univ. Press, 1995.
[5] D. Eppstein, Heesch's Problem.
[6] M. Thompson, Self Surrounding Tiles.