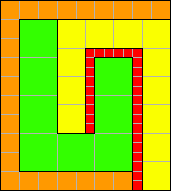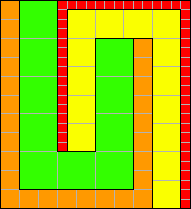Problem of the Month (February 2005)

Previous months have featured problems involving tiling strips of consecutive squares (December 2001) and L's made out of consecutive squares (April 2002). This month we are interested in tiling U's made out of consecutive squares into rectangles. For example, non-degenerate U's made of squares of size 1 through 4 can be made into these 3 different rectangles:

Are these the only such tilings for 4 U's (aside from extending these vertically by 12k units)? Can you prove that there are no tilings for n=2 and n=3? What are the U tilings for n=5? How about larger n?

What is the smallest n for which the first n U's can tile a square? I'll offer a prize of $10 to the smallest square tiled with consecutive U's by the end of the month, with ties going to the first one received.

ANSWERS

Joseph DeVincentis explained why no 2 U or 3 U tiling exists.

Joseph DeVincentis and Philippe Fondanaiche found another 4 U tiling:

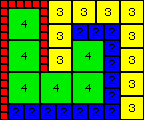
Joseph DeVincentis and Jeremy Galvagni found this 5 U tiling:

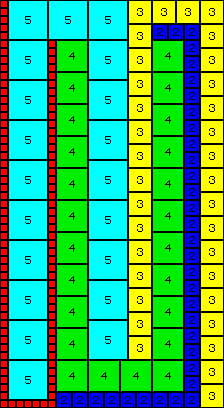
Philippe Fondanaiche found this 5 U tiling:

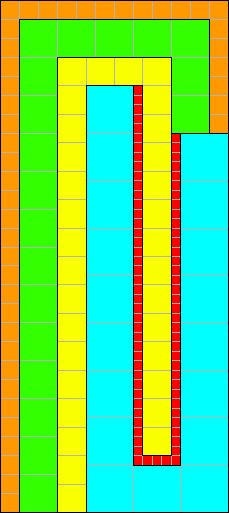
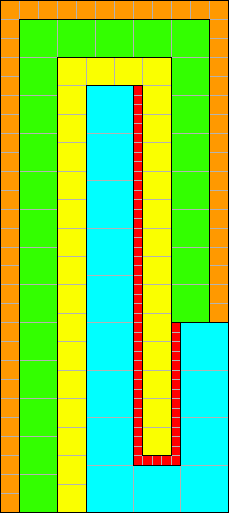
Berend Jan van der Zwaag found these two 5 U tilings:

Joseph DeVincentis found 4 trivial 5 U tilings made by wrapping an extended 4 U tiling with a width 5 U.

Joseph DeVincentis also found a U tiling of a 36×36 square using L's of sizes 1, 2, 4, 5, and 6. This isn't quite what i was looking for, but I sent him $10 anyway.

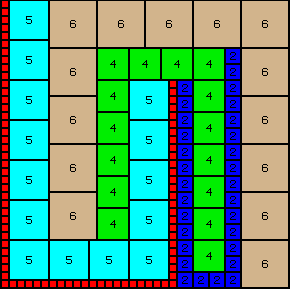
If you can extend any of these results, please e-mail me. Click here to go back to Math Magic. Last updated 3/29/05.