Problem of the Month (June 2005)

This month we generalize the reptile problem by asking which instances of

a₁² + a₂² + . . . + a_n² = b₁² + b₂² + . . . + b_m²

can be demonstrated by tilings of similar shapes. That is, does there exist a shape with the property that copies of size a₁ through a_n can tile the same region as copies of size b₁ through b_m?

Can you find one of the missing tilings? Can you prove that they do not exist? How about for larger sums? We continue the tradition of offering a $10 prize to the most numerous new contributions.

ANSWERS

Gavin Theobald found tilings for every formula of the form:

a² + b² = c² + d² (using right triangles)

Claudio Baiocchi generalized some existing tilings to give tilings for these formulas:

(2N+1)² + 3 × 1² = (N+2)² + 3 × N² (using equilateral triangles)
(5N)² = (5N–1)² + 2N × 2² + (2N–1) × 1²
(5N+1)² = (5N)² + 2N × 2² + (2N+1) × 1²
(5N+4)² = (5N)² + (2N+1) × 4² + (2N) × 2²
(6N)² = (6N–1)² + 2N × 2² + (4N–1) × 1²
(6N+1)² = (6N)² + 2N × 2² + (4N+1) × 1²
(7N)² = (7N–1)² + 2N × 2² + (6N–1) × 1²
(7N+1)² = (7N)² + 2N × 2² + (6N+1) × 1² (using trapezoids)

George Sicherman added these tilings:

a² + b² + c² = s² + (s–a)² + (s–b)² + (s–c)² (where 2s = a+b+c) (using equilateral triangles)

Here are the known tilings:

Sum	Equation	Packing
4	2=1111
9	3=221	?
9	3=211111
10	31=2211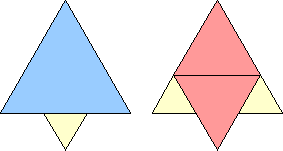
12	3111=222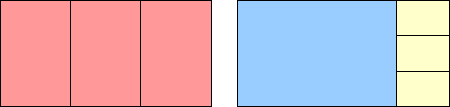
13	32=2221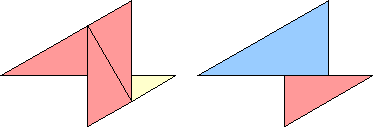
16	4=32111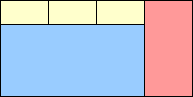
17	41=322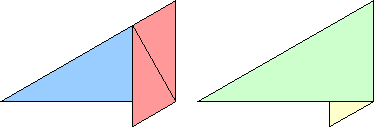
18	411=33	?
18	33=3221	?
18	33=222211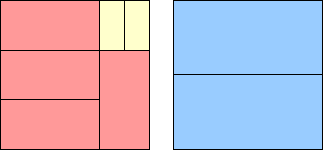
19	4111=331	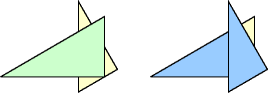George Sicherman
20	42=3311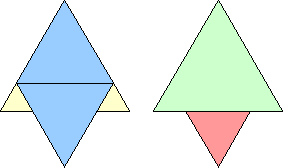
22	4211=332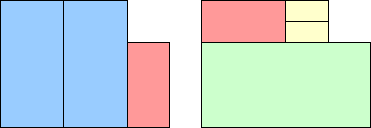
25	5=43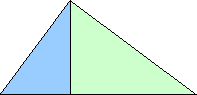
25	5=4221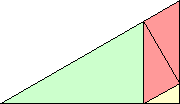
25	43=4221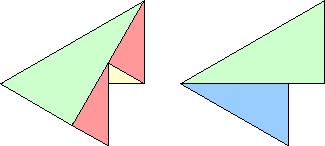
25	5=332111	?
26	51=3322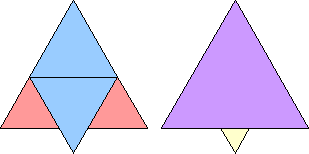
27	511=333	?
27	422111=333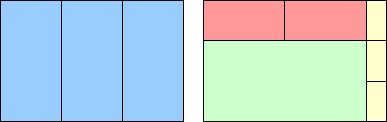
27	4311=333	?
28	5111=3331	George Sicherman
28	5111=4222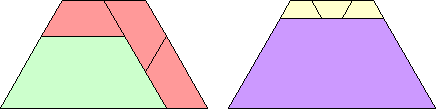
28	4222=3331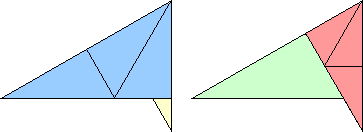
29	52=33311	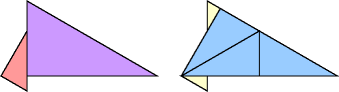George Sicherman
31	5211=3332	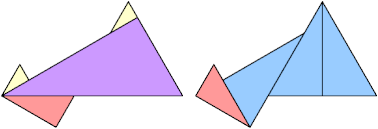George Sicherman
31	5111111=3332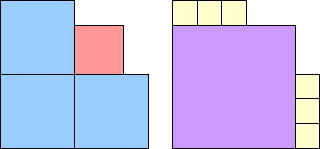
32	52111=44	?
32	51111111=44	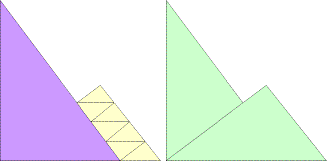Claudio Baiocchi
32	44=33321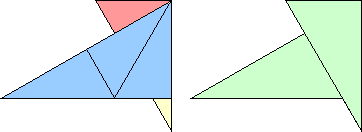
33	522=333211	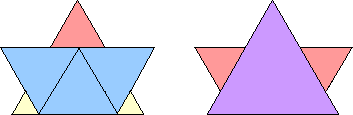Claudio Baiocchi
33	522=441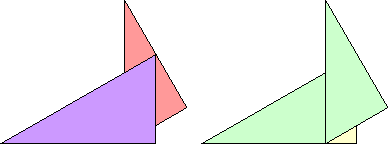
34	53=4411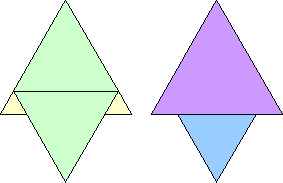
34	53=3332111	George Sicherman
35	44111=33322	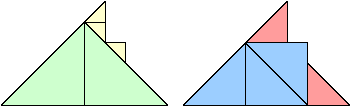George Sicherman
35	52211=4331	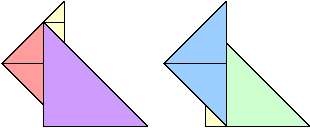George Sicherman
36	6=5311	?
36	6=522111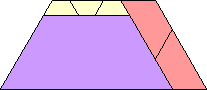
36	6=441111
36	6=43311	?
36	6=4322111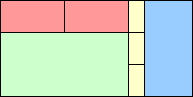
36	6=333221	?
36	6=33222211
36	5311=442	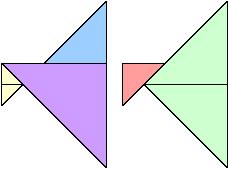George Sicherman
37	61=53111	?
37	61=5222	?
37	61=433111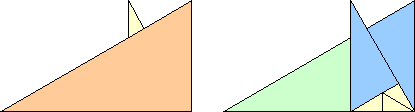
37	61=43222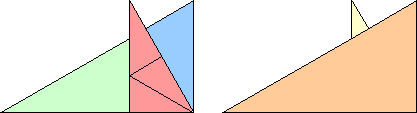
37	61=3332211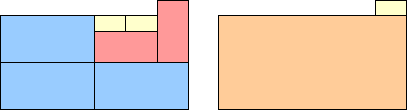
38	611=532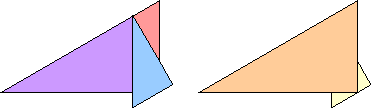
38	611=52221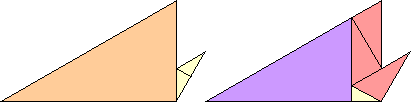
38	611=4332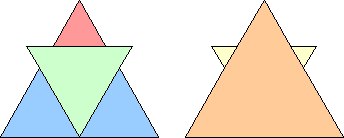
38	52221=4332	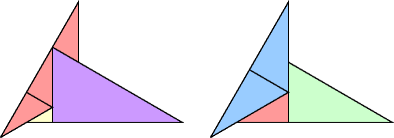George Sicherman
39	6111=333222	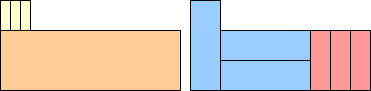Tino Jonker
40	62=53211	George Sicherman
40	62=433211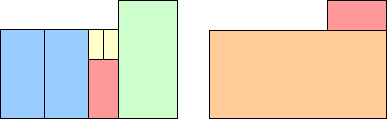
41	621=54	?
41	621=443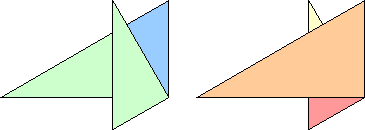
42	6211=541	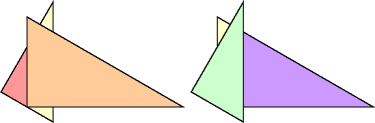George Sicherman
43	62111=4333	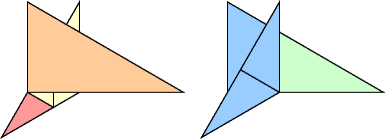George Sicherman
43	62111=533	?
44	622=5331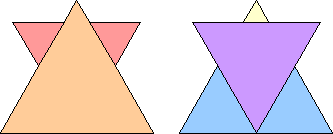
44	622=43331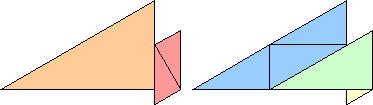
44	622=443111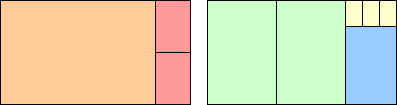
44	5331=44222	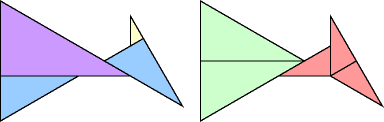George Sicherman
45	63=542	?
45	63=53311	?
45	53311=4432	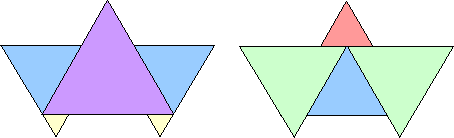Claudio Baiocchi
46	631=5421	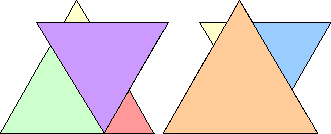Claudio Baiocchi
48	63111=444	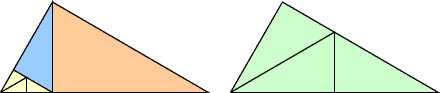George Sicherman
49	7=632	?
49	7=62221	?
49	7=62211111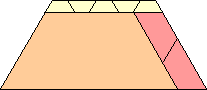
49	7=5422	?
49	7=5421111	?
49	7=5411111111	?
49	7=533211	?
49	7=53222111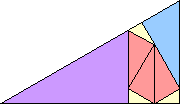
49	7=5222222	?
49	7=5222221111
49	7=4441	?
49	7=4333211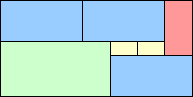
49	7=43222222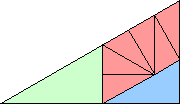
49	7=4222222221	?
49	7=4222222211111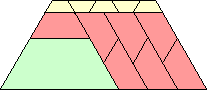
49	7=333332	?
49	632=5422	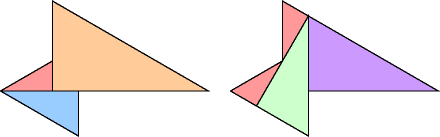George Sicherman
49	632=4441	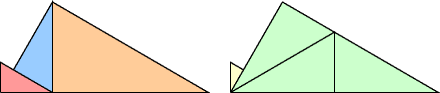George Sicherman
50	71=6321	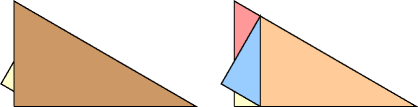George Sicherman
50	71=55	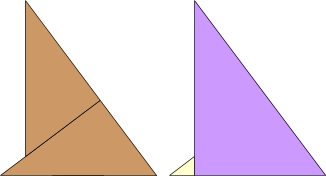Gavin Theobald
50	71=54221	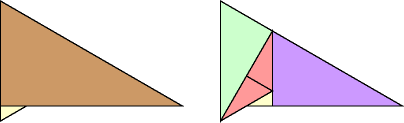George Sicherman
50	71=4433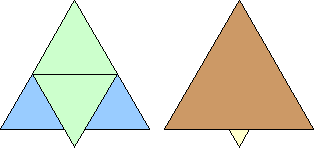
50	6321=55	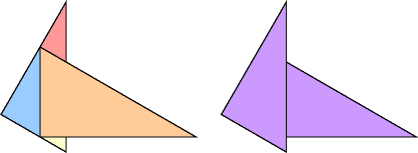George Sicherman
50	55=44411	?
51	711=5431	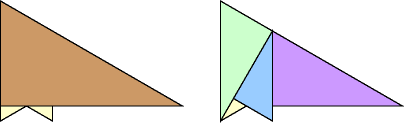George Sicherman
51	551=444111	?
52	7111=64	?
52	7111=62222
52	7111=5333	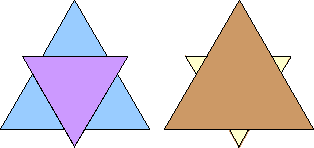Claudio Baiocchi
52	7111=4442	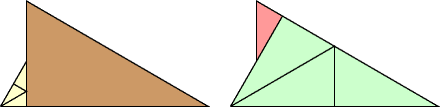George Sicherman
52	7111=43333	?
52	64=5511	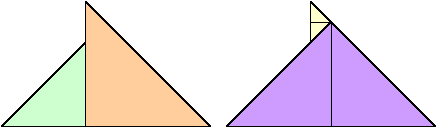George Sicherman
52	5333=4442	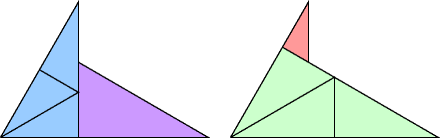George Sicherman
53	72=54222	?
53	72=54221111
53	72=44421	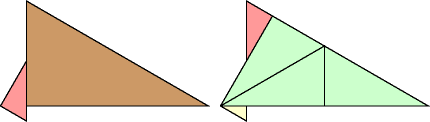George Sicherman
53	72=433331	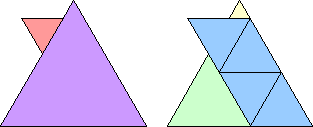Claudio Baiocchi
54	721=6411	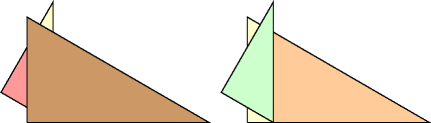George Sicherman
54	721=5432	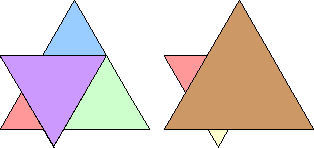Claudio Baiocchi
54	552=444211	George Sicherman
56	72111=63311	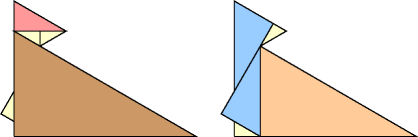George Sicherman
57	552111=4443	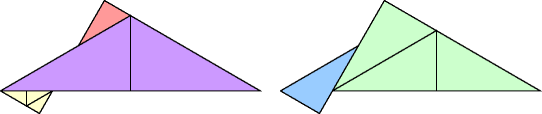George Sicherman
58	73=64211	George Sicherman
58	73=5522	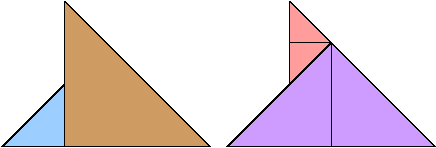George Sicherman
58	73=5441	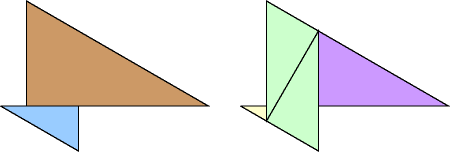George Sicherman
58	7221=6332	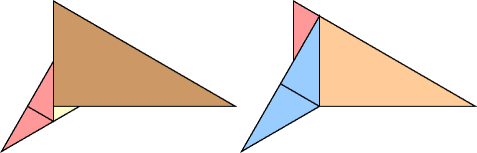George Sicherman
58	6332=5441	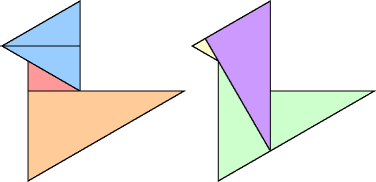George Sicherman